Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Worksheet on Fractions
In worksheet on fractions, all grade students can practice the questions on fractions on a whole number and also on representation of a fraction. This exercise sheet on fractions can be practiced by the students to get more ideas on fractions.
In this worksheet on fractions we will get different types of questions on fractions, comparison of fractions, fractions in ascending order, fractions in descending order, find the fractions to make the fractions equivalent, conversion of fractions, expressed as mixed fractions, express as improper fractions, fractions in lowest terms, reduce to the fractions to the lowest terms and express as a mixed fractions, addition of fractions, subtraction of fractions, multiplication of fractions, division of fractions and word problems on fractions.
1. Answer the following questions for each given shape:
(a)
(b)
(c)
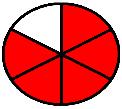
(d)

(e)

(i) How many equals are there in each shape?
(ii) Express each equal part as a fraction.
(iii) Express the shaded part as a fraction.
(iv) Express each unshaded part as a fraction.
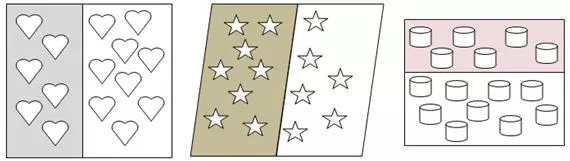
2. Write the fraction for the objects in the shaded part of the closed figure as a part of the whole collection.
3. Write the following fractions in words:
(i) 59 (five-ninth, five upon nine)
(ii) 37
(iii) 211
(iv) 47
(v) 714
(vi) 913
(vii) 310
4. Fill in the blanks:
(i) For two parts out of seven we say ‘two-seventh’ and write 27.
(ii) For ‘four parts out of five’ we say ……………… and write ……………….. .
(iii) For ‘nine parts out of seventeen’ we say …………… and write ………….. .
(iv) For ‘three parts out of six’ we say ……………… and write ……………… .
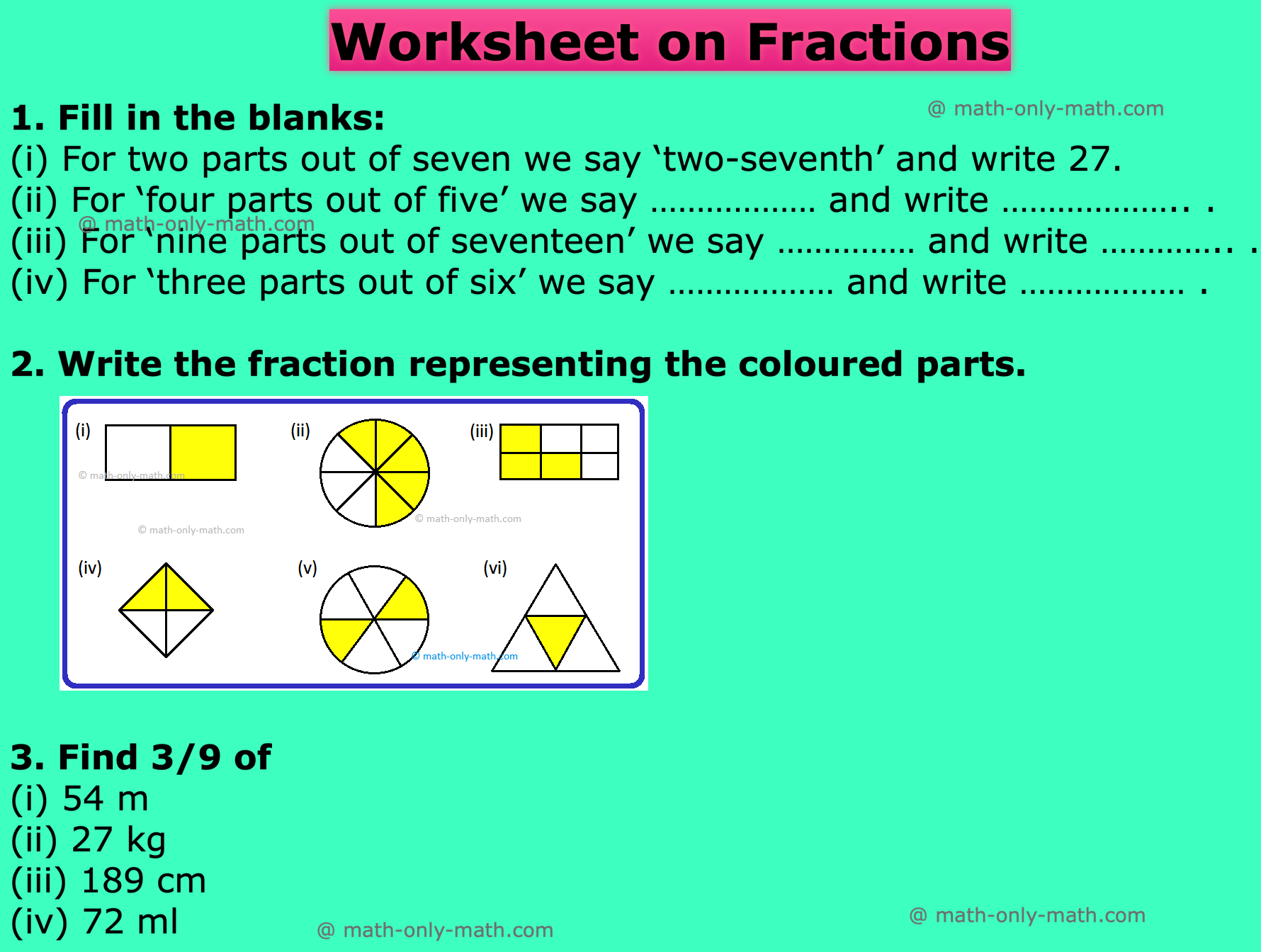
5. Write the fraction representing the coloured parts.
6. Write each of the following as a division.
(i) 37
(ii) 519
(iii) 819
(iv) 2329
7. Write each of the following as a fraction.
(1) 1 ÷ 9
(ii) 2 ÷ 25
(iii) 3 ÷ 74
(iv) 3 ÷ 91
8. Find 39 of
(i) 54 m
(ii) 27 kg
(iii) 189 cm
(iv) 72 ml
9. Represent each of the following fractions on the number line.
(i) 34
(ii) 23
(iii) 37
(iv) 25
10. Nairitee has 45 pencils. She uses 29 of them. How many pencils does she have now?
11. What fraction of an ordinary year is 220 days?
12. Look at the number line given in Fig. and answer the following questions:
(i) What fraction does the point A represent?
(ii) What fraction does the point C represent?
(iii) What fraction does the point D represent?
13. Draw pictures to show the following:
(i) five-eighth of a collection
(ii) five-twelfth of a collection
(iii) four-ninth of a rectangular shape
(iv) three-fourth of a square
14. Change into an equivalent fraction with denominator 32.
(i) 34
(ii) 58
(iii) 12
(iv) 916
15. Fill in each box with >, < or = to make the statements true:
(i) 34 □ 43
(ii) 78 □ 45
(iii) 56 □ 1518
(iv) 1513 □ 1511
(v) 2032 □ 12
(vi) 78 □ 710
(vii) 35 □ 25
(viii) 57 □ 811
(ix) 47 □ 2035
(x) 59 □ 1227
(xi) 23 □ 34
(xii) 36 □ 318
(xiii) 37 □ 57
(xiv) 45 □ 56
(xv) 1417 □ 4251
(xvi) 110 □ 320
(xvii) 45 □ 625
(xviii) 524 □ 1572
16. Reduce the following fractions into their lowest terms:
or, Express in the lowest terms.
(i) 1218
(ii) 2436
(iii) 6296
(iv) 40120
(v) 4056
(vi) 39
(vii) 414
(viii) 1080
(ix) 4060
(x) 3070
(xi) 99110
(xii) 82160
(xiii) 4048
(xiv) 5185
(xv) 763
17. Add the following fractions:
(i) 24 + 14
(ii) 710 + 310
(iii) 14 + 14
(iv) 314 + 114 + 414
(v) 318 + 118 + 718
(vi) 630 + 430
(vii) 414 + 814 + 214
(viii) 815 + 115 + 315
18. Subtract the following fractions.
(i) 914 - 414
(ii) 530 - 430
(iii) 712 - 212
(iv) 834 - 534
19. Which of the following are proper fractions?
(i) 43
(ii) 711
(iii) 619
(iv) 511
(v) 1511
(vi) 148
(vii) 1217
(viii) 2324
(ix) 98
(x) 62
20. Classify the following fractions as proper or improper or mixed fractions:
(i) 1510
(ii) 29
(iii) 267
(iv) 3551
(ν) 1629
(vi) 4111
(vii) 327128
(viii) 42072
21. Which of the following are groups of like fractions?
(i) 15, 25, 814, 618
(ii) 311, 211, 1511, 2211
(iii) 187, 137, 17, 227
(iv) 42, 1021, 2110, 76
22. Check whether the given fractions are equivalent or not.
(i) 35, 915
(ii) 47, 1923
(iii) 49, 4142
(iv) 712, 3245
(v) 311, 2177
(vi) 815, 3065
23. Write 🔲 in each of the following by the correct number:
(i) 45 = 8🔲
(ii) 57 = 20🔲
(iii) 16 = 🔲18
(iv) 512 = 15🔲
(v) 713 = 🔲39
(vi) 715 = 🔲60
24. Find the equivalent fraction of:
(i) 57 having numerator 25
(ii) 56 having denominator 42
(iii) 79 having denominator 72
(iv) 618 having numerator 3
(v) 2030 having numerator 12
(vi) 3033 having denominator 11
25. Convert each of the following mixed fractions into an improper fraction.
or, Express as improper fractions.
(i) 213
(ii) 437
(iii) 8417
(iv) 9620
(v) 235
(vi) 449
(vii) 316
(viii) 567
(ix) 729
(x) 357
(xi) 5712
(xii) 589
(xiii) 2421
(xiv) 8725
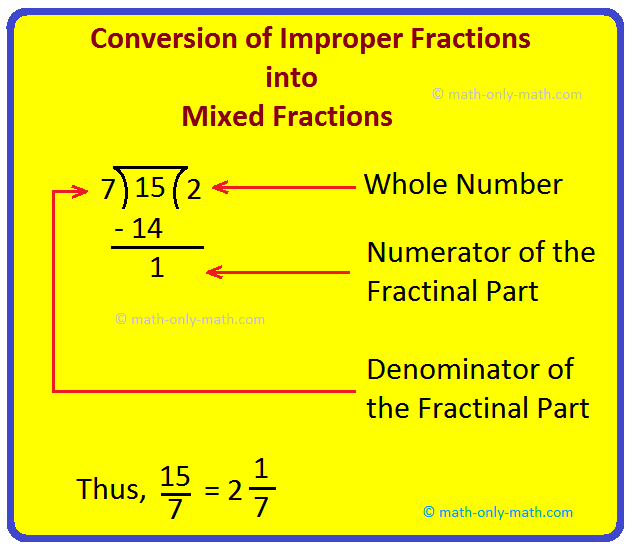
26. Convert each of the following improper fractions into a mixed fraction.
or, Express as mixed fraction
(i) 137
(ii) 287
(iii) 1057
(iv) 34712
(v) 154
(vi) 356
(vii) 194
(viii) 718
(ix) 965
(x) 297
(xi) 7713
(xii) 5417
(xiii) 907
(xiv) 209
27. Write the following in ascending order.
(i) 2/7, 8/7, 5/7, 3/7, 1/7
(ii) 7/5, 7/2, 7/4, 7/3, 7/6
(iii) 2/7, 4/9, 1/8, 3/7, 3/8
(iv) 3/5, 2/5, 2/9, 4/4, 1/3
(v) 7/12, 2/10, 3/8, 2/11, 4/9
(vi) 5/12, 5/24, 13/58, 6/7, 3/8
28. Write the following in descending order.
(i) 3/8, 5/8, 1/8, 7/8, 2/8
(ii) 2/9, 1/3, 4/15, 5/12, 5/6
(iii) 4/5, 2/7, 1/5, 6/7, 3/5
(iv) 9/7, 9/5, 9/4, 9/8, 9/2
(v) 1/2, 2/3, 3/4, 1/6, 3/7
(vi) 2/9, 3/4, 1/2, 2/3, 1/4
29. Fill in the boxes to make the fractions equivalent.
(i) 35 = 🔲20 = 30🔲
(ii) 25 = 16🔲 = 🔲80
(iii) 47 = 🔲28 = 36🔲
(iv) 34 = 15🔲 = 🔲28
(ν) 37 = 9🔲 = 🔲35
(vi) 14 = 3🔲 = 🔲24
(vii) 4🔲 = 1215 = 20🔲
(viii) 37 = 🔲42 = 36🔲
30. Reduce to the lowest terms and express each of them as a mixed number.
(i) 88/6
(ii) 78/12
(iii) 25/15
(iv) 24/9
(v) 77/5
(vi) 54/21
(vii) 45/10
(viii) 62/12
(ix) 69/15
(x) 35/14
31. Find the sum of the following fracions.
(i) 437 + 327
(ii) 1813 + 21139
(iii) 127 + 514 + 1121
(iv) 315 + 234 + 412
(v) 323 + 434 + 535
(vi) 234 + 145 + 135
(vii) 216 + 1114 + 736
(viii) 3316 + 278 + 234
31. Find the difference of the following fractions.
(i) 57 - 27
(ii) 1532 - 716
(iii) 49 - 227
(iv) 38 - 116
(v) 34 - 23
(vi) 234 - 158
(vii) 1316 - 34
(viii) 1634 - 9916
(ix) 567 - 329
(x) 458 - 21924
(xi) 423 - 325
(xii) 527 - 2521
(xiii) 834 - 416
(xiv) 7110 - 5320
(xv) 949 - 7227
32. Find the product of the following fractions:
(i) 47 × 9
(ii) 617 × 12
(iii) 928 × 12
(iv) 138 × 4
(v) 259 × 15
(vi) 6317 × 51
(vii) 259 × 23
(viii) 317 × 217
(ix) 438 × 14
(x) 114 × 235
(xi) 438 × 2115
(xii) 937 × 2617
(xiii) 534 × 315
(xiv) 3910 × 1311
(xv) 656 × 413
33. Divide the following fractions:
(i) 25 ÷ 9
(ii) 47 ÷ 12
(iii) 14 ÷ 12
(iv) 17 ÷ 38
(v) 217 ÷ 517
(vi) 322 ÷ 611
(vii) 158 ÷ 12
(viii) 435 ÷ 17
(ix) 547 ÷ 38
(x) 267 ÷ 1617
(xi) 2117 ÷ 1334
(xii) 838 ÷ 227
(xiii) 512 ÷ 413
(xiv) 723 ÷ 167
(xv) 638 ÷ 234
(xvi) 525 ÷ 3215
(xvii) 2516 ÷ 5512
(xviii) 627 ÷ 35
(xix) 267 ÷ 3124
(xx) 617 ÷ 1151
(xxi) 322 ÷ 12325
Worksheet on Fractions Answers:
3. (ii) three-seventh, three upon seven
(iii) two-eleventh, two upon eleven
(iv) four-seventh, four upon seven
(v) seven-fourteenth, seven upon fourteen
(vi) nine-thirteenth, nine upon thirteen
(vii) three-tenth, three upon ten
4. (ii) four-fifth, 45
(iii) nine-seventeenth, 917
(iv) three-sixth, 36
5. (i) 12
(ii) 58
(iii) 36
(iv) 24
(v) 26
(vi) 14
6. (i) 3 ÷ 7
(ii) 5 ÷ 19
(iii) 8 ÷ 19
(iv) 23 ÷ 29
7. (i) 19
(ii) 225
(iii) 374
(iv) 391
8. (i) 18 m
(ii) 9 kg
(iii) 63 cm
(iv) 24 ml
9.
10. 35 pencils
11. 220365
12. (i) A represents as 112
(ii) C represents as 712
(iii) D represents as 912
13.
14. (i) 2432
(ii) 2032
(iii) 1632
(iv) 1832
15. (i) <
(ii) <
(iii) =
(iv) <
(v) <
(vi) >
(vii) >
(viii) <
(ix) =
(x) >
(xi) <
(xii) >
(xiii) <
(xiv) <
(xv) =
(xvi) <
(xvii) >
(xviii) =
16. (i) 23
(ii) 23
(iii) 3148
(iv) 13
(v) 57
(vi) 13
(vii) 27
(viii) 1080
(ix) 18
(x) 37
(xi) 910
(xii) 4180
(xiii) 56
(xiv) 35
(xv) 19
17. (i) 34
(ii) 1010 = 1
(iii) 24 = 12
(iv) 814 = 47
(v) 1118
(vi) 1030 = 13
(vii) 1414 = 1
(viii) 1215 = 45
18. (i) 514
(ii) 130
(iii) 512
(iv) 334
19. (ii), (iii), (iv), (vii), (viii) are proper fractions because numerators are less than the denominators.
20. (i) improper fraction
(ii) proper fraction
(iii) mixed fraction
(iv) proper fraction
(ν) proper fraction
(vi) improper fraction
(vii) improper fraction
(viii) mixed fraction
21. (ii) and (iii) are like fractions.
22. (i) equivalent fraction
(ii) not equivalent fraction
(iii) not equivalent fraction
(iv) not equivalent fraction
(v) equivalent fraction
(vi) not equivalent fraction
23. (i) 10
(ii) 28
(iii) 3
(iv) 36
(v) 21
(vi) 28
24. (i) 2535
(ii) 3542
(iii) 5672
(iv) 39
(v) 1218
(vi) 1011
25. (i) 73
(ii) 317
(iii) 14017
(iv) 18620
(v) 135
(vi) 409
(vii) 196
(viii) 417
(ix) 659
(x) 267
(xi) 6712
(xii) 539
(xiii) 4621
(xiv) 20725
26. (i) 167
(ii) 4
(iii) 15
(iv) 281112
(v) 334
(vi) 556
(vii) 434
(viii) 878
(ix) 1915
(x) 417
(xi) 51213
(xii) 3317
(xiii) 1267
(xiv) 229
27. (i) 1/7, 2/7, 3/7, 3/7, 5/7, 8/7
(ii) 7/6, 7/5, 7/4, 7/3, 7/2
(iii) 1/8, 2/7, 3/8, 3/7, 4/9
(iv) 2/9, 1/3, 2/5, 3/5, 4/4
(v) 2/11, 2/10, 3/8, 4/9, 7/12
(vi) 5/24, 13/58, 3/8, 5/12, 6/7
28. (i) 7/8, 5/8, 3/8, 2/8, 1/8
(ii) 5/6, 5/12, 1/3, 4/15, 2/9
(iii) 6/7, 4/5, 3/5, 2/7, 1/5
(iv) 9/2, 9/4, 9/5, 9/7, 9/8
(v) 3/4, 2/3, 1/2, 3/7, 1/6
(vi) 3/4, 2/3, 1/2, 1/4, 2/9
29. (i) 12, 50
(ii) 40, 32
(iii) 16, 63
(iv) 20, 21
(ν) 21, 15
(vi) 12, 6
(vii) 5, 25
(viii) 18, 84
30. (i) 1423
(ii) 612
(iii) 123
(iv) 223
(v) 1525
(vi) 247
(vii) 412
(viii) 516
(ix) 435
(x) 212
31. (i) 757
(ii) 33539
(iii) 22942
(iv) 10920
(v) 14160
(vi) 6320
(vii) 3109252
(viii) 81316
31. (i) 37
(ii) 132
(iii) 1027
(iv) 516
(v) 112
(vi) 118
(vii) 716
(viii) 7316
(ix) 24063
(x) 156
(xi) 1415
(xii) 3121
(xiii) 4712
(xiv) 11920
(xv) 21027
32. (i) 517
(ii) 4417
(iii) 367
(iv) 512
(v) 3813
(vi) 315
(vii) 11927
(viii) 44119
(ix) 1332
(x) 314
(xi) 9124
(xii) 2222119
(xiii) 1825
(xiv) 45355
(xv) 291118
33. (i) 245
(ii) 121
(iii) 148
(iv) 821
(v) 25
(vi) 14
(vii) 314
(viii) 3215
(ix) 1467
(x) 218161
(xi) 13337
(xii) 385128
(xiii) 1726
(xiv) 4539
(xv) 2722
(xvi) 13447
(xvii) 44265
(xviii) 101121
(xix) 480511
(xx) 926
(xxi) 25352
If students have any queries regarding the Worksheet on expanded form of a number questions please fill-up the comment box so that we can help you.
However, suggestions for further improvement, from all quarters would be greatly appreciated.
● Fractional Numbers - worksheets
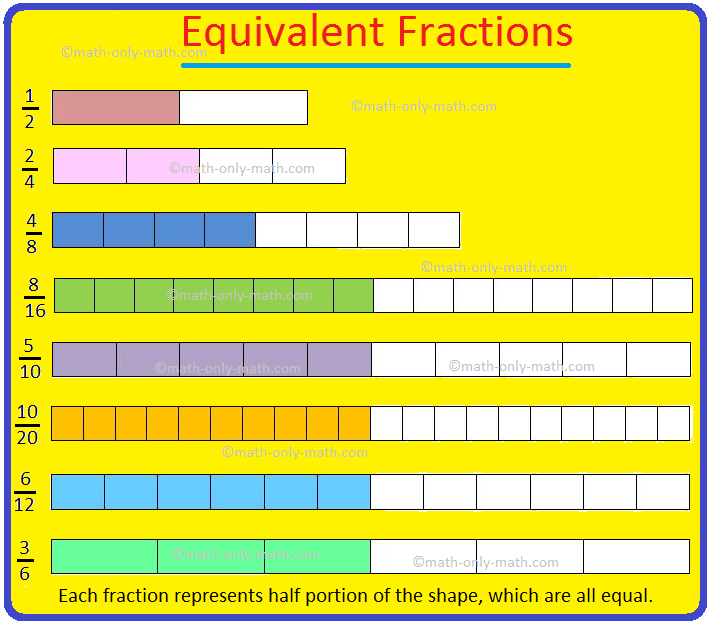
Worksheet on Equivalent Fractions.
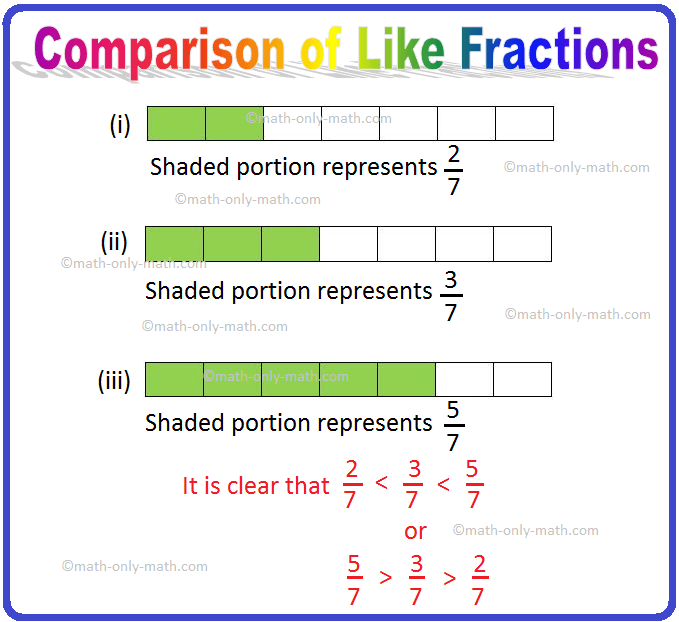
Worksheet on Comparison of Like Fractions.
Worksheet on Conversion of Fractions.
Worksheet on Changing Fractions.
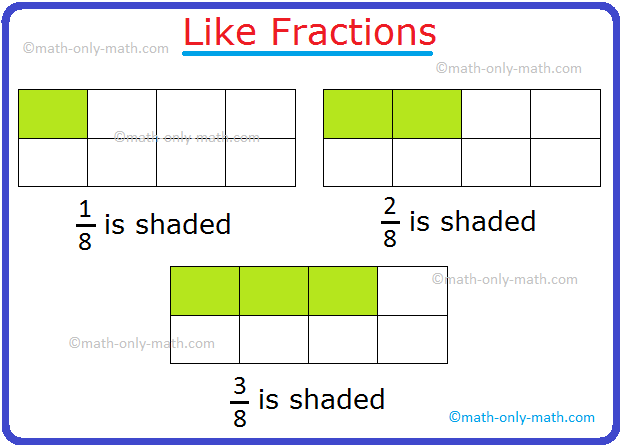
Worksheet on Types of Fractions.
Worksheet on Reducing Fraction.
Worksheet on Addition of Fractions having the Same Denominator.
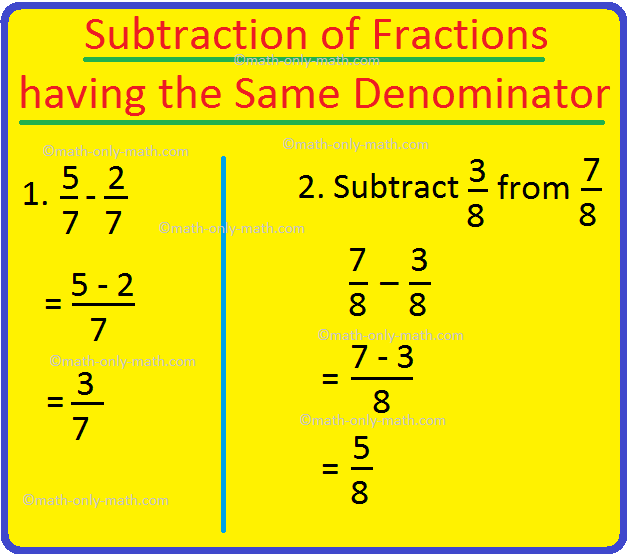
Worksheet on Subtraction of Fractions having the Same Denominator.
Worksheet on Add and Subtract Fractions.
Worksheet on Fractional Numbers.
From Worksheet on Fractions to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Successor and Predecessor | Successor of a Whole Number | Predecessor
Jul 29, 25 12:59 AM
The number that comes just before a number is called the predecessor. So, the predecessor of a given number is 1 less than the given number. Successor of a given number is 1 more than the given number… -
Worksheet on Area, Perimeter and Volume | Square, Rectangle, Cube,Cubo
Jul 28, 25 01:52 PM
In this worksheet on area perimeter and volume you will get different types of questions on find the perimeter of a rectangle, find the perimeter of a square, find the area of a rectangle, find the ar… -
Worksheet on Volume of a Cube and Cuboid |The Volume of a RectangleBox
Jul 25, 25 03:15 AM
We will practice the questions given in the worksheet on volume of a cube and cuboid. We know the volume of an object is the amount of space occupied by the object.1. Fill in the blanks: -
Volume of a Cuboid | Volume of Cuboid Formula | How to Find the Volume
Jul 24, 25 03:46 PM
Cuboid is a solid box whose every surface is a rectangle of same area or different areas. A cuboid will have a length, breadth and height. Hence we can conclude that volume is 3 dimensional. To measur… -
Volume of a Cube | How to Calculate the Volume of a Cube? | Examples
Jul 23, 25 11:37 AM
A cube is a solid box whose every surface is a square of same area. Take an empty box with open top in the shape of a cube whose each edge is 2 cm. Now fit cubes of edges 1 cm in it. From the figure i…

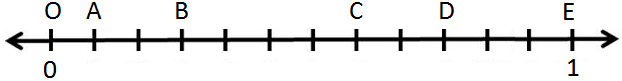
![To convert a percentage into a fraction, place the given number over 100 and reduce it to its lowest term. Consider the following example: (i) 20% [We know % = 1/100]](/images/convert-a-percentage-into-a-fraction.png)










New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.