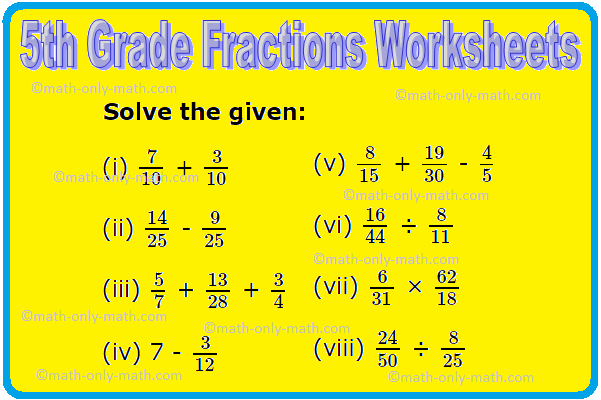
Worksheet on Add and Subtract Fractions

Recall the topic carefully and practice the questions given in the math worksheet on add and subtract fractions. The question mainly covers addition with the help of a fraction number line, subtraction with the help of a fraction number line, add the fractions with the same denominator, subtract the fractions with the same denominator and word problems on add and subtract fractions.

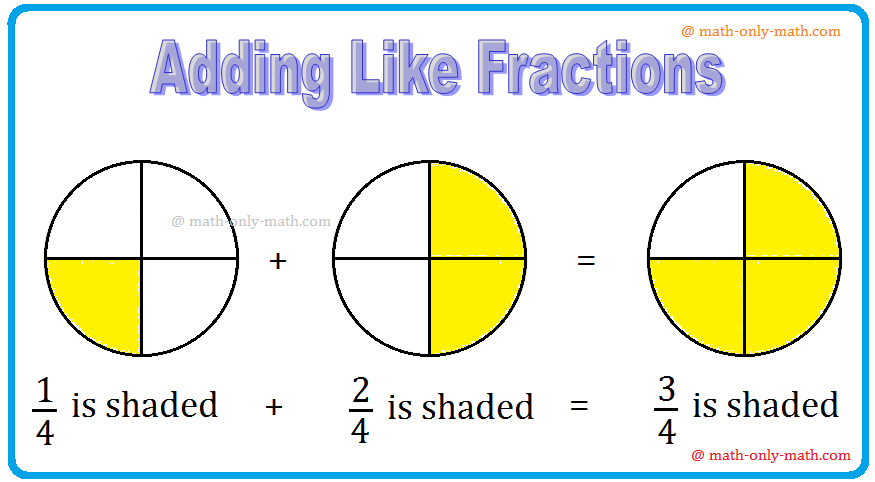
I. Addition and Subtraction of Like Fractions:

For adding or subtracting like fractions, we follow the following steps.

Working Rules for Addition and Subtraction of Like Fractions:

Step I: Add or subtract the numerators of the given fractions and keep the denominator as it is.

Step II: Reduce the fraction of its lowest term.

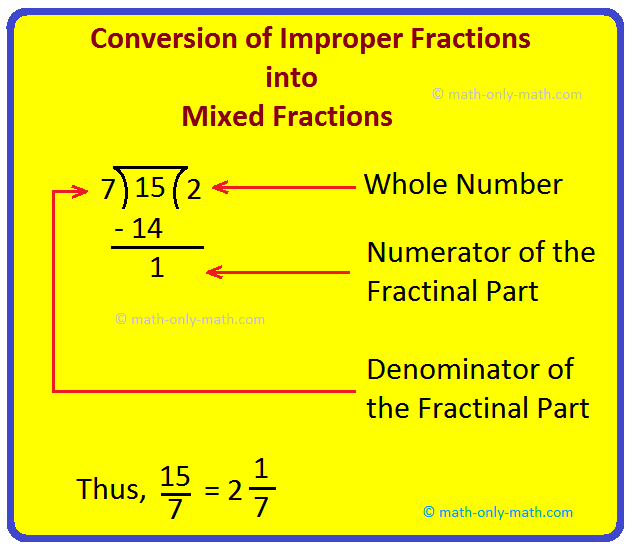
Step III: If the result is an improper fraction, convert it into a mixed fraction.

In short,

sum or difference of like fractions

                  = Sum of Difference of Numerators

                             Common denominator

II: Addition and Subtraction of Unlike Fractions:

For adding or subtracting unlike fractions, we follow these steps.

Working Rules  for Addition and Subtraction of Like Fractions:

Step I: Find the LCM of denominators of the given fractions.

Step II: Convert unlike fractions into like fractions by making LCM as their denominator.

Step III: Add or subtract the like fractions.

1. Add with the help of a fraction number line:

(a) $\frac{2}{3}$ + $\frac{1}{3}$

(b) $\frac{3}{7}$ + $\frac{2}{7}$

(c) $\frac{6}{10}$ + $\frac{1}{10}$

2. Subtract with the help of a fraction number line: 

(a) $\frac{9}{10}$ - $\frac{3}{10}$

(b) $\frac{5}{6}$ - $\frac{2}{6}$

(c) $\frac{7}{8}$ - $\frac{4}{8}$

3. Add: 

(a) $\frac{7}{10}$ + $\frac{2}{10}$

(b) $\frac{6}{8}$ + $\frac{4}{8}$

(c) $\frac{5}{9}$ + $\frac{2}{9}$ + $\frac{1}{9}$

(d) $\frac{8}{11}$ + $\frac{2}{11}$

(e) $\frac{4}{9}$ + $\frac{7}{9}$

(f) $\frac{3}{8}$ + $\frac{5}{8}$ + $\frac{2}{8}$

(g) $\frac{4}{6}$ + $\frac{2}{6}$ + $\frac{1}{6}$

(h) $\frac{7}{12}$ + $\frac{5}{12}$ + $\frac{6}{12}$

4. Find the difference. Remember to show the answer in the simplest form:

(a) $\frac{9}{14}$ - $\frac{4}{14}$

(b) $\frac{6}{11}$ - $\frac{3}{11}$

(c) $\frac{8}{12}$ - $\frac{4}{12}$

(d) $\frac{12}{15}$ - $\frac{9}{15}$

(e) $\frac{12}{13}$ - $\frac{9}{13}$

(f) $\frac{6}{10}$ - $\frac{2}{10}$

(g) $\frac{9}{16}$ - $\frac{5}{16}$

(h) $\frac{12}{14}$ - $\frac{8}{14}$

5. Add the following fractions:

(i) $\frac{4}{7}$ + $\frac{5}{7}$ 

(ii) $\frac{3}{7}$ + $\frac{11}{7}$

(iii) $\frac{3}{23}$ + $\frac{11}{23}$ + $\frac{1}{23}$

(iv) $\frac{2}{5}$ + $\frac{3}{20}$

(v) $\frac{2}{7}$ + $\frac{4}{9}$

(vi) 3$\frac{7}{9}$ + 2$\frac{5}{6}$ + 6$\frac{1}{3}$

(vii) $\frac{2}{15}$ + $\frac{5}{12}$ + $\frac{4}{20}$

(viii) $\frac{11}{16}$ + $\frac{3}{10}$ + $\frac{9}{24}$

(ix) 1$\frac{3}{8}$ + $\frac{5}{8}$

(x) 4$\frac{3}{5}$ + 2$\frac{7}{20}$

(xi) $\frac{8}{15}$ + 2$\frac{1}{15}$

(xii) 3$\frac{1}{5}$ + 4$\frac{1}{10}$ + 5$\frac{1}{15}$

6. Subtract the following fractions:

(i) $\frac{7}{9}$ - $\frac{2}{9}$

(ii) $\frac{3}{4}$ - $\frac{3}{4}$

(iii) 8$\frac{2}{9}$ - 2$\frac{5}{9}$

(iv) $\frac{11}{20}$ - $\frac{4}{15}$

(v) $\frac{8}{15}$ - $\frac{1}{7}$

(vi) 5$\frac{1}{40}$ - $\frac{4}{25}$

(vii) 6$\frac{2}{16}$ - 3$\frac{1}{6}$

(viii) 3$\frac{7}{8}$  - $\frac{6}{7}$

(ix) 7$\frac{3}{21}$ - 6$\frac{3}{28}$

7. Simplify the following combination of addition and subtraction of fractions:

(i) $\frac{3}{4}$ + $\frac{2}{3}$ - $\frac{1}{2}$

(ii) $\frac{3}{8}$ + $\frac{7}{8}$ - $\frac{1}{8}$

(iii) $\frac{12}{25}$ - $\frac{3}{25}$ + $\frac{9}{25}$

(iv) 2$\frac{1}{2}$+ 3$\frac{3}{5}$ - 1$\frac{1}{3}$

(v) 11$\frac{1}{4}$ - 4$\frac{3}{5}$ + $\frac{8}{11}$

(vi) $\frac{7}{18}$ - $\frac{1}{12}$ + $\frac{1}{6}$

Worksheet on Word Problems on Addition and Subtraction of Like Fractions:

8. Solve these problems:

(a) $\frac{1}{3}$ of the school garden has vegetable and another $\frac{1}{3}$ has flowers. What part of the garden is left to grow grass?

(b) Sam spent $\frac{1}{6}$ of his Sunday doing home work and $\frac{3}{6}$ of the day watching cricket. What part of the day was left to do other things?

(c) My mother ate $\frac{1}{8}$ of the cake and my father $\frac{3}{8}$. How much of the cake has been eaten and how much is left?

(d) Pearl bought $\frac{2}{3}$ of her school books last week. What part is still left to be bought?

(e) Sonia walked $\frac{3}{8}$ of the distance to school and ran $\frac{5}{8}$ of the distance. How much more of the distance does she need to cover?

(f) Emma likes chocolate. One day she bought a chocolate and ate $\frac{5}{8}$ of it in the morning and $\frac{2}{8}$ in the evening. How much part of the chocolate has she eaten?

(g) James and Lucas are eating a pizza. James ate $\frac{3}{4}$ of the pizza and Lucas ate $\frac{1}{4}$ of pizza. Who ate more pizza?

(h) Sophia completed $\frac{2}{5}$ of her homework before going out for play. She did $\frac{1}{5}$ of her homework after the play. How much homework did she complete altogether?

(i) David distributed $\frac{19}{24}$ apples in his class and gave $\frac{2}{24}$ to his friend Richard. What fraction of apples he gave away in all?

(j) Mary read $\frac{2}{9}$ of her book in the morning and $\frac{5}{9}$ in the evening. What fraction of the book has she read?

(k) A piece of ribbon is $\frac{12}{15}$ m long. A piece of $\frac{4}{15}$ m is cut from it. What is the fraction of the remaining ribbon?

(l) Nancy saves $\frac{2}{7}$ of her salary and uses $\frac{1}{7}$ for paying the house rent. How much salary is she left with?

Answers for the worksheet on add and subtract fractions are given below to check the exact answers of the above questions on adding & subtracting fractions. 

5. (i) 1$\frac{2}{7}$ 

(ii) 2

(iii) $\frac{15}{23}$ 

(iv) $\frac{11}{20}$ 

(v) $\frac{46}{63}$ 

(vi) 12$\frac{17}{18}$

(vii) $\frac{45}{60}$ or, $\frac{3}{4}$

(viii) 1$\frac{87}{240}$

(ix) 2

(x) 6$\frac{19}{20}$

(xi) 2$\frac{9}{15}$

(xii) 12$\frac{11}{30}$

6. (i) $\frac{5}{9}$

(ii) $\frac{1}{4}$

(iii) 5$\frac{6}{9}$ or 5$\frac{2}{3}$

(iv) $\frac{17}{60}$

(v) $\frac{41}{105}$

(vi) 4$\frac{173}{200}$

(vii) 2$\frac{46}{48}$ or 2$\frac{23}{24}$

(viii) 3$\frac{1}{56}$

(ix) 1$\frac{3}{84}$ or 1$\frac{1}{28}$

7. (i) $\frac{11}{12}$

(ii) 1$\frac{1}{8}$

(iii) $\frac{18}{25}$

(iv) 4$\frac{23}{30}$

(v) 7$\frac{83}{220}$

(vi) $\frac{17}{36}$

● Fractional Numbers - worksheets

Worksheet on Equivalent Fractions.

Worksheet on Fractions.

Worksheet on Comparison of Like Fractions.

Worksheet on Conversion of Fractions.

Worksheet on Changing Fractions.

Worksheet on Types of Fractions.

Worksheet on Reducing Fraction.

Worksheet on Addition of Fractions having the Same Denominator.

Worksheet on Subtraction of Fractions having the Same Denominator.

Worksheet on Add and Subtract Fractions.

Worksheet on Fractional Numbers.

4th Grade Math Activities

From Worksheet on Add and Subtract Fractions to HOME PAGE