Subtraction of Mixed Fractions

We will learn how to solve subtraction of mixed fractions or subtraction of mixed numbers.

There are two methods to subtract the mixed fractions.


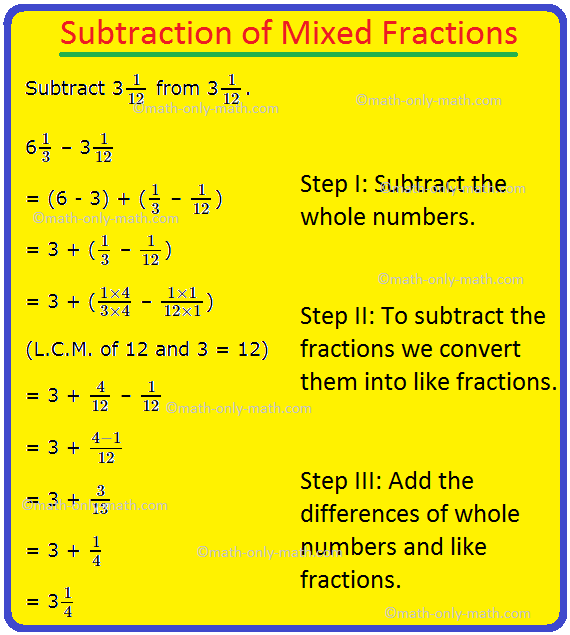
1. Subtract 3\(\frac{1}{12}\) from 3\(\frac{1}{12}\).

Solution:

Method I.

6\(\frac{1}{3}\) – 3\(\frac{1}{12}\)

= (6 - 3) + (\(\frac{1}{3}\) – \(\frac{1}{12}\))

= 3 + (\(\frac{1}{3}\) – \(\frac{1}{12}\))

= 3 + (\(\frac{1 × 4}{3 × 4}\) – \(\frac{1 × 1}{12 × 1}\))

(L.C.M. of 12 and 3 = 12)

= 3 + \(\frac{4}{12}\) – \(\frac{1}{12}\)

= 3 + \(\frac{4 - 1}{12}\)

= 3 + \(\frac{3}{13}\)

= 3 + \(\frac{1}{4}\)

= 3\(\frac{1}{4}\)

Step I: Subtract the whole numbers.


Step II: To subtract the fractions we convert them into like fractions.


Step III: Add the differences of whole numbers and like fractions.


Method II:

6\(\frac{1}{3}\) – 3\(\frac{1}{12}\)

= \(\frac{(6 × 3) + 1}{3}\) + \(\frac{(3 × 12) + 1}{12}\)

= \(\frac{19}{3}\) – \(\frac{37}{12}\)

= \(\frac{19 × 4}{3 × 4}\) – \(\frac{37 × 1}{12 × 1}\),

(L.C.M. of 3 and 12 = 12)

= \(\frac{76}{12}\) – \(\frac{37}{12}\)

= \(\frac{76 - 37}{12}\)

= \(\frac{39}{12}\)

= \(\frac{13}{4}\)

= 3\(\frac{1}{4}\)

Step I: Change the mixed numbers into improper fractions.


Step II: Make the fractions like fraction to have a common denominator.


Step III: Subtract and express the fraction in the simplest form.


2. Subtract 1\(\frac{5}{12}\) from 3\(\frac{3}{8}\)

Solution:

We first convert the mixed numbers into improper fractions.

1\(\frac{5}{12}\) = \(\frac{1  × 12 + 5}{12}\) = \(\frac{17}{12}\)

3\(\frac{3}{8}\) = \(\frac{3  × 8 + 3}{8}\) = \(\frac{27}{8}\)

Now, \(\frac{27}{8}\) - \(\frac{17}{12}\) = \(\frac{27 × 3}{8 × 3}\) - \(\frac{17 × 2}{12 × 2}\)

LCM of 8 and 12 is 24

\(\frac{81}{24}\) - \(\frac{34}{24}\) = \(\frac{81 - 34}{24}\)

            = \(\frac{47}{24}\)

            = 1\(\frac{23}{24}\)

Hence, 3\(\frac{3}{8}\) - 1\(\frac{5}{12}\) = 1\(\frac{23}{24}\)

Subtraction of Mixed Fractions


Word Problems on Subtraction of Mixed Fractions:

3. Ron used 3\(\frac{1}{4}\) litres of paint from a tin of 5\(\frac{1}{2}\) l, to color the walls of his room. What fraction of paint is still left in the tin?

Answer: \(\frac{9}{4}\) litres


4. Sam has a cloth of length 16 m. He took 13\(\frac{1}{4}\) m of cloth from it to make curtains for the house. How much cloth is still left with him for further use?

Answer: 2\(\frac{3}{4}\) m


5. Ten years ago a forest was spread up to a distance of 33 km. Due to forest fires and industrial set up now it is spread upto a distance of 16\(\frac{1}{5}\) km. What fraction of forest has been destroyed in the last 10 years? What measures can be taken to protect these forests?

Answer: 16\(\frac{4}{5}\) km


6. A drum full of rice weighs 84\(\frac{1}{2}\) kg. If the weight of empty drum is 12\(\frac{1}{6}\) kg, find the weight of the rice.

Answers: 72\(\frac{1}{3}\) kg

● Related Concepts







4th Grade Math Activities

From Subtraction of Mixed Fractions to HOME PAGE




Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.



New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.

Share this page: What’s this?

Recent Articles

RSS

  1. Word Problems on Area and Perimeter | Free Worksheet with Answers

    Jul 26, 24 04:58 PM

    word problems on area and perimeter

    Read More

  2. Worksheet on Perimeter | Perimeter of Squares and Rectangle | Answers

    Jul 26, 24 04:37 PM

    Most and Least Perimeter
    Practice the questions given in the worksheet on perimeter. The questions are based on finding the perimeter of the triangle, perimeter of the square, perimeter of rectangle and word problems. I. Find…

    Read More

  3. Perimeter and Area of Irregular Figures | Solved Example Problems

    Jul 26, 24 02:20 PM

    Perimeter of Irregular Figures
    Here we will get the ideas how to solve the problems on finding the perimeter and area of irregular figures. The figure PQRSTU is a hexagon. PS is a diagonal and QY, RO, TX and UZ are the respective d…

    Read More

  4. Perimeter and Area of Plane Figures | Definition of Perimeter and Area

    Jul 26, 24 11:50 AM

    Perimeter of a Triangle
    A plane figure is made of line segments or arcs of curves in a plane. It is a closed figure if the figure begins and ends at the same point. We are familiar with plane figures like squares, rectangles…

    Read More

  5. 5th Grade Math Problems | Table of Contents | Worksheets |Free Answers

    Jul 26, 24 01:35 AM

    In 5th grade math problems you will get all types of examples on different topics along with the solutions. Keeping in mind the mental level of child in Grade 5, every efforts has been made to introdu…

    Read More