Subscribe to our YouTube channel for the latest videos, updates, and tips.
Multiplying Fractions
We will discuss here about multiplying fractions by a whole number, by a fractional number or by another mixed fractional number.
I. Multiplication of Fractional Number by a Whole Number:
We have learnt 4 × 5 = 4 times 5
= 5 + 5 + 5 + 5
= 20
In the same way 6 × 17 = 6 times 17
= 17 + 17 + 17 + 17 + 17 + 17
= 1+1+1+1+1+17
= 67
i.e., 6 × 17 = 6×17 = 67
Multiply 4 × 35
4 × 35 = 4 times 4 × 35
= 35 + 35 + 35 + 35
= 125
i.e. 4 × 35 = 4×35 = 125
Product of a whole number and a fractional number =
Product of Whole Number × Numerator of the Fractional NumberDenominator of the Fractional Number
For examples:
34 × 5 = 3×54 = 154
67 × 2 = 6×27 = 127
7 × 45 = 7×45 = 285
4 × 311 = 4×311 = 1211
Let us multiply 14 by 3. We use the rule of repeated addition to find the product.
We can say that 14 of 3 = 34
To multiply a fraction by a whole number, we multiply the
numerator of the fraction by the whole number and reduce the fraction to the
lowest terms, if so required.
For example:
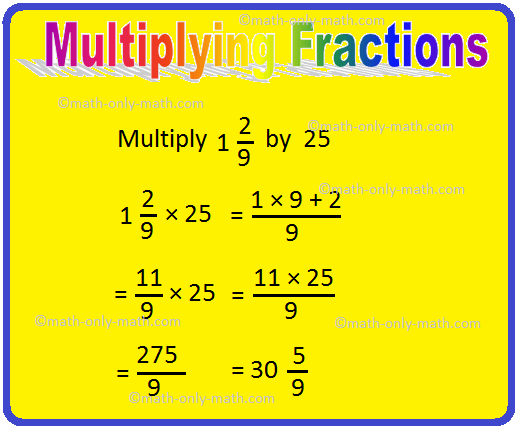
(i) Multiply 129 by 25
Solution:
129 × 25
= 1×9+29
= 119 × 25
= 11×259
= 2759
= 3059
|
(ii) Multiply 2/3 by 7 Solution: 2/3 × 7 = (2 × 7)/3 = 14/3 = 4 2/3 |
We simply multiply the numerator of the fractional number by the whole number. The denominator remains the same. |
|
(iv) Multiply 235 by 6 Solution: 235 × 6
= (2 × 5 + 3)/5 × 6 = (10 + 3)/5 × 6 = 13/5 × 6 = (13 × 6)/5 = 78/5 = 1535 |
We change the mixed numbers into improper fractions and then simply multiply the numerator of the fractional number by the whole number. The denominator remains the same. |
II. Multiplication of Fractional Number by Another Fractional Number:
For example:
|
(i) Multiply 2/5 by 4/5 Solution: 2/5 × 4/5 = (2 × 4)/(5 × 5)
= 8/25 |
Step I: We multiply the numerators. Step II: We multiply the denominators. Step III: We write the fraction in the simplest form. |
|
(ii) Multiply 8/9 by 7/10 Solution: 8/9 × 7/10 = (8 × 7)/(9 × 10)
= 56/90 |
We simply multiply the numerators of the fractional numbers and then multiply the denominators of the fractional numbers. Write the fraction in the simplest form. |
(iii) Multiply 47 × 25
Multiply the numerators to get the numerator of the product and
Multiply the denominators to get the denominator of the product.
Reduce the product to the lowest terms.
Therefore, 47 × 25 = 4×27×5 = 835
III: Product of More than Two Fractions:
For examples:
(i) Multiply 910 × 25 × 37
Method I: 910 × 25 × 37 = 9×2×310×5×7 = 54350
H.C.F. of 54 and 350 is 2
54÷2350÷2 = 27175
Therefore, 910 × 25 × 37 = 27175
Method II: 910 × 25 × 37 = ?
Write the numbers as the products of prime factors.
Cancel the numbers common in numerator and denominator.
Therefore, 910 × 25 × 37 = 3×3×⧸2×1×3×1⧸2×5×5×1×7×1
= 27175
(v) Multiply 47, 311 and 58.
Solution:
To multiply two or more fractions, we multiply the numerators of given fractions to find the new numerator of the product and multiply the denominators to get the denominator of the product.
Hence, 47 × 311 × 58 = 4×3×57×11×8
= 60616
|
(vi) Multiply 1021 × 524 × 350 1021 × 524 × 350 = 10×5×321×24×50 = ⧸2×⧸5×⧸5×⧸3⧸3×7×3×⧸2×2×2×2×⧸5×⧸5 = 1168 |
10 = 2 × 5 5 = 5 × 1 3 = 3 × 1 21 = 3 × 7 24 = 3 × 2 × 2 × 2 50 = 2 × 5 × 5 |
III. Multiplication of a Mixed Number by Another Mixed Number:
For Example:
|
(i) Multiply 2 1/3 by 1 ¾ Solution: 2 1/3 × 1 ¾ = 7/3 × 7/4
= 49/12 = 4 1/12 |
We change the mixed numbers into improper fractions and then we multiply as usual. |
|
(ii) Multiply 1 7/9 by 3 5/11
Solution: 1 7/9 × 3 5/11 = 16/9 × 38/11 = (16 × 38)/(9 × 11) = 608/99 = 6 14/99 |
We change the mixed numbers into improper fractions and then we multiply as usual. |
(iii) Multiply 1178 by 3124
Solution:
Let us first convert mixed numbers into improper fractions.
1178 = 11×8+78 = 958
3124 = 3×24+124 = 7324
Now, 958 × 7324 = 95×738×24
= 6935192
= 3623192
(iv) Multiply 312 × 215
Questions and Answers on Multiplying Fractions:
I. Find the product:
(i) 519 × 1
(ii) 67 × 5
(iii) 914 × 6
(iv) 413 × 0
(v) 17 × 56
(vi) 1110 × 8
(vii) 17 × 81
(viii) 13 × 75 × 29
(ix) 415 × 1021
(x) 12 of 100
(xi) 13 of 60
(xii) 45 of 811
Answers:
I. (i) 519
(ii) 427
(iii) 367
(iv) 0
(v) 542
(vi) 845
(vii) 117
(viii) 14135
(ix) 863
(x) 50
(xi) 20
(xii) 3255
II. Multiply and write the product in lowest terms.
(i) 12 × 40
(ii) 13 × 150
(iii) 27 × 21
(iv) 738 × 0
(v) 3165 × 1
(vi) 8 × 1724
(vii) 37 × 715
(viii) 932 × 836
(ix) 1115 × 4588
(x) 210 ×322 ×4030
(xi) 16 ×25 ×34
(xii) 317 ×2144
Answers:
II. (i) 20
(ii) 50
(iii) 6
(iv) 0
(v) 3165
(vi) 173
(vii) 15
(viii) 116
(ix) 38
(x) 255
(xi) 120
(xii) 112
III. Find the Product and Reduce it the Lowest Terms:
(i) 413 × 213
(ii) 6 × 512
(iii) 117 × 214
(iv) 34 × 13 × 26
(v) 112 × 523 × 415
(vi) 79 × 1015 × 321
(vii) 1648 × 1224 × 1530
(viii) 1938 × 24 × 820
(ix) 642 × 115 × 1550
Answer:
III. (i) 1019
(ii) 33
(iii) 247
(iv) 112
(v) 35710
(vi) 227
(vii) 112
(viii) 110
(ix) 9175
IV. Simplify. (use Prime Factorisation)
(i) 79 × 1821 × 610
(ii) 2436 × 8127 × 510
(iii) 1012 × 1214 × 1420
(iv) 1516 × 3230 × 14
(v) 12 × 48 × 166
(vi) 1322 × 1126 × 46
Answer:
IV. (i) 25
(ii) 1
(iii) 12
(iv) 14
(v) 23
(vi) 16
V. Multiply:
(i) 4 × 611
(ii) 813 × 3
(iii) 25 × 10
(iv) 57 × 5
(v) 8 × 56
(vi) 712 × 2
(vii) 15 × 14
(viii) 19 × 13
Answer:
V. (i) 2211
(ii) 11113
(iii) 4
(iv) 347
(v) 623
(vi) 116
(vii) 334
(viii) 613
VI. Find the given quantity.
(i) 17 of 28 kg apples
(ii) 215 of $300
(iii) 59 of 54 km
(iv) 25 of 70 chairs
Answers:
VI. (i) 4 kg apples
(ii) $40
(iii) 30 km
(iv) 28 chairs
VII: Word problems on Multiplying Fractions:
1. 215 m of cloth is required to make a shirt. Ron wants to make 25 shirts, what length of cloth does he need?
Answer: 55 m of cloth
2. 34 cups of milk is required to make a cake of 1 kg. How many cups of milk is required to make a cake of 412 kg?
Answer: 338 cups
3. Shelly bought 1634 liters of juice. If the cost of 1 liter juice is $8, find the total cost of juice?
Answer: $134
4. The weight of each bag is 414 Kg. What would be the weight of 36 such bags?
Answer: 153 kg
5. Sam works for 628 hours each day. For how much time will she work in a month if she works for 24 days in a month?
Answer: 150 hours
● Related Concepts
- Fraction of a Whole Numbers
- Representation of a Fraction
- Equivalent Fractions
- Properties of Equivalent Fractions
- Finding Equivalent Fractions
- Reducing the Equivalent Fractions
- Verification of Equivalent Fractions
- Finding a Fraction of a Whole Number
- Like and Unlike Fractions
- Comparison of Like Fractions
- Comparison of Fractions having the same Numerator
- Comparison of Unlike Fractions
- Fractions in Ascending Order
- Fractions in Descending Order
- Types of Fractions
- Changing Fractions
- Conversion of Fractions into Fractions having Same Denominator
- Conversion of a Fraction into its Smallest and Simplest Form
- Addition of Fractions having the Same Denominator
- Addition of Unlike Fractions
- Addition of Mixed Fractions
- Word Problems on Addition of Mixed Fractions
- Worksheet on Word Problems on Addition of Mixed Fractions
- Subtraction of Fractions having the Same Denominator
- Subtraction of Unlike Fractions
- Subtraction of Mixed Fractions
- Word Problems on Subtraction of Mixed Fractions
- Worksheet on Word Problems on subtraction of Mixed Fractions
- Addition and Subtraction of Fractions on the Fraction Number Line
- Word Problems on Multiplication of Mixed Fractions
- Worksheet on Word Problems on Multiplication of Mixed Fractions
- Multiplying Fractions
- Dividing Fractions
- Word Problems on Division of Mixed Fractions
- Worksheet on Word Problems on Division of Mixed Fractions
From Multiplying Fractions to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade Circle Worksheet | Free Worksheet with Answer |Practice Math
Jul 10, 25 11:41 AM
In 5th Grade Circle Worksheet you will get different types of questions on parts of a circle, relation between radius and diameter, interior of a circle, exterior of a circle and construction of circl… -
Construction of a Circle | Working Rules | Step-by-step Explanation |
Jul 09, 25 01:29 AM
Construction of a Circle when the length of its Radius is given. Working Rules | Step I: Open the compass such that its pointer be put on initial point (i.e. O) of ruler / scale and the pencil-end be… -
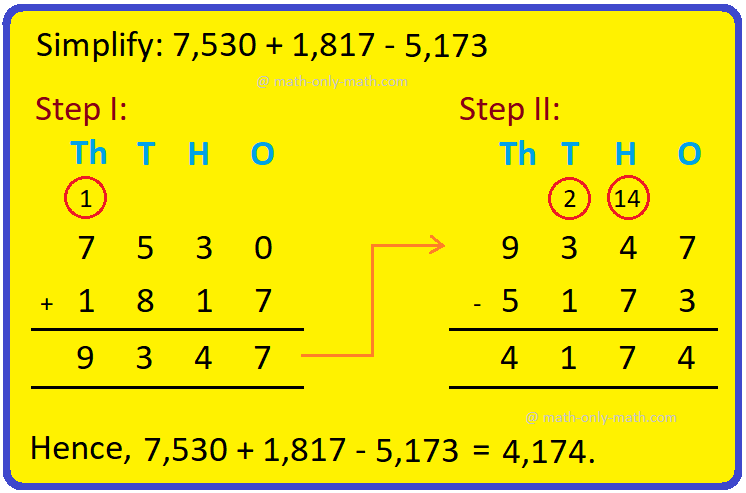
Combination of Addition and Subtraction | Mixed Addition & Subtraction
Jul 08, 25 02:32 PM
We will discuss here about the combination of addition and subtraction. The rules which can be used to solve the sums involving addition (+) and subtraction (-) together are: I: First add -
Addition & Subtraction Together |Combination of addition & subtraction
Jul 08, 25 02:23 PM
We will solve the different types of problems involving addition and subtraction together. To show the problem involving both addition and subtraction, we first group all the numbers with ‘+’ and… -
5th Grade Circle | Radius, Interior and Exterior of a Circle|Worksheet
Jul 08, 25 09:55 AM
A circle is the set of all those point in a plane whose distance from a fixed point remains constant. The fixed point is called the centre of the circle and the constant distance is known







New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.