Subscribe to our YouTube channel for the latest videos, updates, and tips.
Addition of Unlike Fractions
We will learn how to solve addition of unlike fractions.
In order to add unlike fractions, first we convert them as like fractions with same denominator in each fraction with the help of method explained earlier and then we add the fractions.
Let us consider some of the examples of adding unlike fractions:
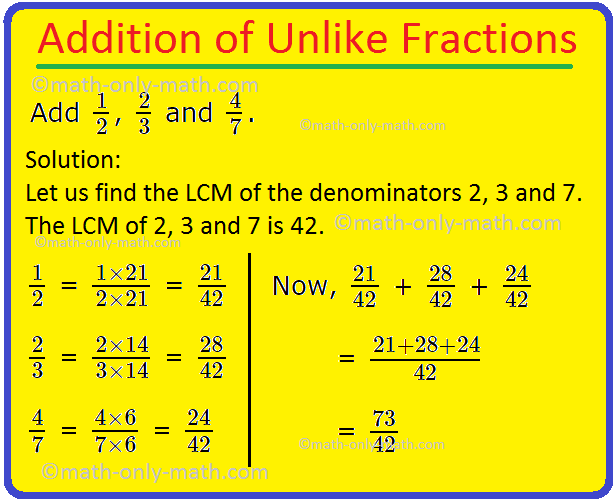
1. Add 12, 23 and 47.
Solution:
Let us find the LCM of the denominators 2, 3 and 7.
The LCM of 2, 3 and 7 is 42.
12 = 1×212×21 = 2142
23 = 2×143×14 = 2842
47 = 4×67×6 = 2442
Therefore, we get the like fractions 12, 23 and 47.
Now, 2142 + 2842 + 2442
= 21+28+2442
= 7342
2. Add 78 and 910
Solution:
The L.C.M. of the denominators 8 and 10 is 40.
78 = 7×58×5 = 3540, (because 40 ÷ 8 = 5)
78 = 9×410×4 = 3640, (because 40 ÷ 10 = 4)
Thus, 78 + 910
= 3540 + 3640
= 35+3640
= 7140
= 13140
3. Add 16 and 512
Solution:
Let L.C.M. of the denominators 6 and 12 is 12.
16 = 1×26×2 = 212, (because 12 ÷ 6 = 2)
512 = 5×112×1 = 512, (because 12 ÷ 12 = 1)
Thus, 16 + 512
= 212 + 512
= 2+512
= 712
4. Add 23, 115 and 56
Solution:
The L.C.M. of the denominators 3, 15 and 6 is 30.
23 = 2×103×10 = 2030, (because 30 ÷ 3 = 10)
115 = 1×215×2 = 230, (because 30 ÷ 15 = 2)
56 = 5×56×5 = 2530, (because 30 ÷ 6 = 5)
Thus, 23 + 115 + 56
= 2030 + 230 + 2530
= 20+2+2530
= 4730
= 11730
More examples on Addition of Unlike Fractions (Fractions having Different Denominators)
5. Add 16 + 34
|
Solution: First Method: Step I: Find the L.C.M. of the denominators 6 and 4. L.C.M. of 6 and 4 = 2 × 3 × 2 =12 |
Step II:Write the equivalent fractions of 16 and 34 with denominator 12.
1×26×2 = 212
3×34×3 = 912
Step III:Add the equivalent fractions
16 + 34
= 212 + 912
= 2+912
= 1112
Add 16 + 34
Second Method:
|
16 + 34
= (12÷6)×1+(12÷4)×312 = (2×1)+(3×312 = 2+912 = 1112 |
Steps: Divide 12 by I denominator. Multiply the quotient with I numerator. Divide 12 by II denominator. Multiply the quotient with II numerator. |
|
6. Add 38 + 24 + 616 Solution: L.C.M. of 8, 4, 16 = 2 × 2 × 2 × 2 = 16 38 + 24 + 616 = (16÷8)×3+(16÷4)×2+(16÷16)×616 = (2×3)+(4×2)+(1×6)16 = 6+8+616 = 2016 = 54 = 114 |
Addition of Mixed Numbers
7. Add 226 + 513 + 145
Solution:
First Method:
Separate the whole numbers and proper fractions.
226 + 513 + 145 = (2 + 5 + 1) + 26 + 13 + 45
= 8 + 26 + 13 + 45
L.C.M. of 6, 3 and 5 is 30.
= 8 + (30÷6)×2+(30÷3)×1+(30÷5)×430
= 8 + (5×2)+(10×1)+(6×4)30
= 8 + 10+10+2430
= 8 + 4430
= 8 + 2215
= 8 + 1715
Second Method:
Add 226 + 513 + 145
Convert the mixed number into improper fractions and find the sum
226 = (2×6)+26 = 146
513 = (5×3)+13 = 163
145 = (1×5)+45 = 95
Therefore, 226 + 513 + 145 = 146 + 163 + 95
= 14×56×5 + 16×103×10 + 9×65×6
= 7030 + 16030 + 5430
= 70+160+5430
= 28430
= 14215
= 9715
8. Add 26, 4 and 712
|
Solution: 4 = 41 26 + 4 + 712 = 26 + 41 + 712 L.C.M. of 6, 1, 12 is 12
= (2×2)+(12×4)+(1×7)12 = 4+48+712 = 5912 = 41112 |
To add unlike fractions, we first convert them into like fractions. In order to make a common denominator we find the LCM of all different denominators of the given fractions and then make them equivalent fractions with a common denominator.
Word Problems on Addition of Unlike Fractions:
1. On Monday Michael read 516 of the book. On Wednesday he reads 48 of the book. What fraction of the book has Michael read?
Solution:
On Monday Michael read 516 of the book.
On Wednesday he reads 48 of the book.
Now add the two fractions
516 + 48
Let us find the LCM of the denominators 16 and 8.
The LCM of 16 and 8 is 16.
516 = 5×116×1 = 516
48 = 4×28×2 = 816
Therefore, we get the like fractions 516 and 816.
Now, 516 + 816
= 5+816
= 1316
Therefore, Michael read in two days 1316 of the book.
2. Sarah ate 13 part of the pizza and her sister ate 12 of the pizza. What fraction of the pizza was eaten by both sisters?
Solution:
Sarah ate 13 part of the pizza.
Her sister ate 12 of the pizza.
Now add the two fractions
13 + 12
Let us find the LCM of the denominators 3 and 2.
The LCM of 3 and 2 is 6.
13 = 1×23×2 = 26
12 = 1×32×3 = 36
Therefore, we get the like fractions 26 and 36.
Now, 26 + 36
= 2+36
= 56
Therefore, 56 of the pizza was eaten by both sisters.
3. Catherine is preparing for her final exam. She study 922 hours on Wednesday and 511 hours on Sunday. How many hours she studied in two days?
Solution:
Catherine study 922 hours on Wednesday.
Again, she study 511 hours on Sunday.
Now add the two fractions
922 + 511
Let us find the LCM of the denominators 22 and 11.
The LCM of 22 and 11 is 22.
922 = 9×122×1 = 922
511 = 5×211×2 = 1022
Therefore, we get the like fractions 922 and 1022.
Now, 922 + 1022
= 9+1022
= 1922
Therefore, Catherine studied a total 922 hours in two days.
Questions and Answers Addition of Unlike Fractions:
1. Add the following Unlike Fractions:
(i) 34 + 56
(ii) 17 + 23 + 67
(iii) 78 + 56 + 410
(iv) 37 + 25 + 611
(v) 358 + 416 + 4712
Answer:
1. (i) 1712
(ii) 123
(iii) 213120
(iv) 1144385
(v) 1238
Related Concept
- Fraction of a Whole Numbers
- Representation of a Fraction
- Equivalent Fractions
- Properties of Equivalent Fractions
- Like and Unlike Fractions
- Comparison of Like Fractions
- Comparison of Fractions having the same Numerator
- Types of Fractions
- Changing Fractions
- Conversion of Fractions into Fractions having Same Denominator
- Conversion of a Fraction into its Smallest and Simplest Form
- Addition of Fractions having the Same Denominator
- Subtraction of Fractions having the Same Denominator
- Addition and Subtraction of Fractions on the Fraction Number Line
From Addition of Unlike Fractions to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade Circle Worksheet | Free Worksheet with Answer |Practice Math
Jul 10, 25 11:41 AM
In 5th Grade Circle Worksheet you will get different types of questions on parts of a circle, relation between radius and diameter, interior of a circle, exterior of a circle and construction of circl… -
Construction of a Circle | Working Rules | Step-by-step Explanation |
Jul 09, 25 01:29 AM
Construction of a Circle when the length of its Radius is given. Working Rules | Step I: Open the compass such that its pointer be put on initial point (i.e. O) of ruler / scale and the pencil-end be… -
Combination of Addition and Subtraction | Mixed Addition & Subtraction
Jul 08, 25 02:32 PM
We will discuss here about the combination of addition and subtraction. The rules which can be used to solve the sums involving addition (+) and subtraction (-) together are: I: First add -
Addition & Subtraction Together |Combination of addition & subtraction
Jul 08, 25 02:23 PM
We will solve the different types of problems involving addition and subtraction together. To show the problem involving both addition and subtraction, we first group all the numbers with ‘+’ and… -
5th Grade Circle | Radius, Interior and Exterior of a Circle|Worksheet
Jul 08, 25 09:55 AM
A circle is the set of all those point in a plane whose distance from a fixed point remains constant. The fixed point is called the centre of the circle and the constant distance is known
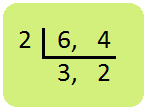

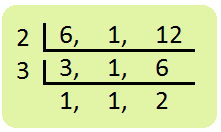



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.