Rules of Trigonometric Signs

In this section we will learn about the rules of trigonometric signs. On a plane paper let O be a fixed point. Draw two mutually perpendicular lines \(\overrightarrow{XOX'}\) and \(\overrightarrow{YOY'}\) through O divide the plane paper into four quadrants.

We know that, the distance measured from O along \(\overrightarrow{XO}\) is positive and that along \(\overrightarrow{OX'}\) is negative; similarly again, the distance from O along \(\overrightarrow{OY}\) is positive and that along \(\overrightarrow{OY'}\) is negative.

Now, take a rotating line \(\overrightarrow{OA}\) rotates about O in the clockwise or anti-clockwise direction and starting from the initial position angle ∠XOA = θ. Depending on the value of θ the final arm \(\overrightarrow{OA}\) may be in the first quadrant or second quadrant or third quadrant or fourth quadrant. Take a point B on \(\overrightarrow{OA}\) and draw \(\overline{BC}\) perpendicular to \(\overrightarrow{OX}\) (or, \(\overrightarrow{OX'}\)).

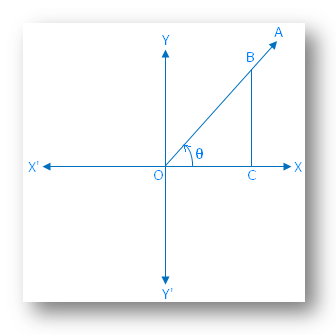
Diagram 1: (i) \(\overline{OC}\) will be positive if it is measured from O along \(\overrightarrow{OX}\) (ii) \(\overline{CB}\) will be positive if it is measured from O along \(\overrightarrow{OY}\) (iii) \(\overline{OB}\) is positive of the final arm \(\overrightarrow{OA}\)

Diagram 1

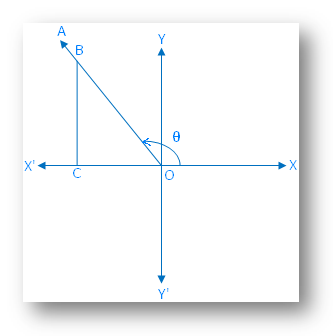
Diagram 2: (i) \(\overline{OC}\) will be negative if it is measured from O along \(\overrightarrow{OX'}\) (ii) \(\overline{CB}\) will be positive if it is measured from O along \(\overrightarrow{OY}\) (iii) \(\overline{OB}\) is positive of the final arm \(\overrightarrow{OA}\)

Diagram 2

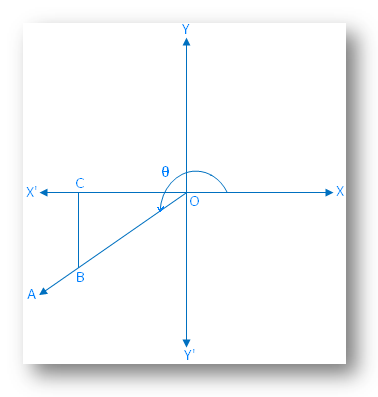
Diagram 3: (i) \(\overline{OC}\) will be negative if it is measured from O along \(\overrightarrow{OX'}\) (ii) \(\overline{CB}\) will be negative if it is measured from O along \(\overrightarrow{OY'}\) (iii) \(\overline{OB}\) is positive of the final arm \(\overrightarrow{OA}\)

Diagram 3

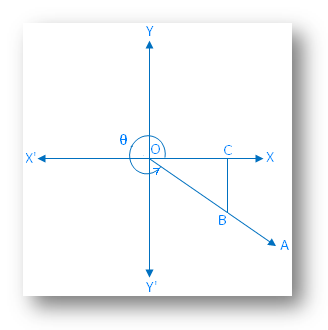
Diagram 4: (i) \(\overline{OC}\) will be positive if it is measured from O along \(\overrightarrow{OX}\) (ii) \(\overline{CB}\) will be negative if it is measured from O along \(\overrightarrow{OY'}\) (iii) \(\overline{OB}\) is positive of the final arm \(\overrightarrow{OA}\)

Diagram 4

Therefore, the rules of trigonometric signs of the sides of the right-angled triangle OBC are as follows:

(i) \(\overline{OC}\) will be positive if it is measured from O along \(\overrightarrow{OX}\) as shown in the diagram 1 and diagram 4

(ii) \(\overline{OC}\) will be negative if it is measured from O along \(\overrightarrow{OX'}\) as shown in the diagram 2 and diagram 3

(iii) \(\overline{CB}\) will be positive if it is measured from O along \(\overrightarrow{OY}\) as shown in the diagram 1 and diagram 2

(iv) \(\overline{CB}\) will be negative if it is measured from O along \(\overrightarrow{OY'}\) as shown in the diagram 3 and diagram 4

(v) \(\overline{OB}\) is positive for all positions of the final arm \(\overrightarrow{OA}\).

● Trigonometric Functions

• Basic Trigonometric Ratios and Their Names
• Restrictions of Trigonometrical Ratios
• Reciprocal Relations of Trigonometric Ratios
• Quotient Relations of Trigonometric Ratios
  
• Limit of Trigonometric Ratios
  
• Trigonometrical Identity
• Problems on Trigonometric Identities
• Elimination of Trigonometric Ratios
• Eliminate Theta between the equations
• Problems on Eliminate Theta
• Trig Ratio Problems
• Proving Trigonometric Ratios
• Trig Ratios Proving Problems
• Verify Trigonometric Identities
• Trigonometrical Ratios of 0°
• Trigonometrical Ratios of 30°
• Trigonometrical Ratios of 45°
• Trigonometrical Ratios of 60°
• Trigonometrical Ratios of 90°
• Trigonometrical Ratios Table
• Problems on Trigonometric Ratio of Standard Angle
  
• Trigonometrical Ratios of Complementary Angles
• Rules of Trigonometric Signs
• Signs of Trigonometrical Ratios
  
• All Sin Tan Cos Rule
• Trigonometrical Ratios of (- θ)
• Trigonometrical Ratios of (90° + θ)
• Trigonometrical Ratios of (90° - θ)
• Trigonometrical Ratios of (180° + θ)
• Trigonometrical Ratios of (180° - θ)
• Trigonometrical Ratios of (270° + θ)
• Trigonometrical Ratios of (270° - θ)
• Trigonometrical Ratios of (360° + θ)
• Trigonometrical Ratios of (360° - θ)
• Trigonometrical Ratios of any Angle
• Trigonometrical Ratios of some Particular Angles
• Trigonometric Ratios of an Angle
• Trigonometric Functions of any Angles
• Problems on Trigonometric Ratios of an Angle
• Problems on Signs of Trigonometrical Ratios

11 and 12 Grade Math

From Rules of Trigonometric Signs to HOME PAGE