Word Problems on Multiplication and Division of Whole Numbers
We will learn how to solve step-by-step the word problems on multiplication and division of whole numbers. We know, we need to do multiplication and division in our daily life. Let us solve some word problem examples.
Word problems on multiplying and dividing of large numbers:
1. The cost of a chair is $980.50. Find the cost of such 2035 chairs.
Solution:
Cost of a chair = 980.50
Number of chairs = × 2035
490250
2991500
0000000
+ 196100000
Cost of 2035 chairs = 1995317.50
Therefore, cost of 2035 chairs is $1995317.50.
2. A tyre factory produces 6348 tyres a day. How many tyres will the factory produce in 460 days?
Solution:
Number of tyres a tyre factory produces in one day = 6348
Number of day = × 460
0000
380880
+ 2539200
Total number of tyres produces in 460 days = 2920080
Therefore, the tyre factory will produce 2920080 tyres in 460 days.
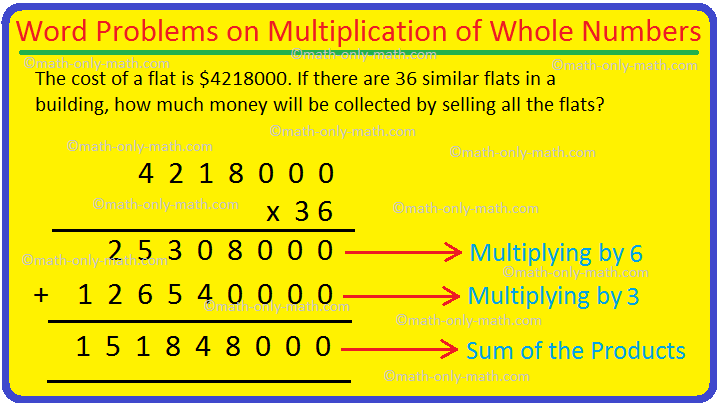
3. The cost of a flat is $4218000. If there are 36 similar flats in a building, how much money will be collected by selling all the flats?
Solution:
Cost of one flat = $4218000
Number of similar flats = 36
Cost of 36 flats = $4218000 × 36
Hence, the money collected = $151848000
4. 470988 books are to be arranged equally in shelves. If 378 books are arranged in each shelf, how many shelves will be needed?
Solution:
Total number of books = 470988
Number of books arranged in each shelf = 378
Number of shelves needed = 470988 ÷ 378
Therefore, 1246 shelves needed to arrange 470988 books equally.
5. The cost of 534 train tickets was $457104. Find the cost of one ticket?
Solution:
Number of train tickets = 534
Cost of 534 train tickets = $457104
Cost of 1 train ticket = 457104 ÷ 534
Therefore, cost of one train ticket is $856.
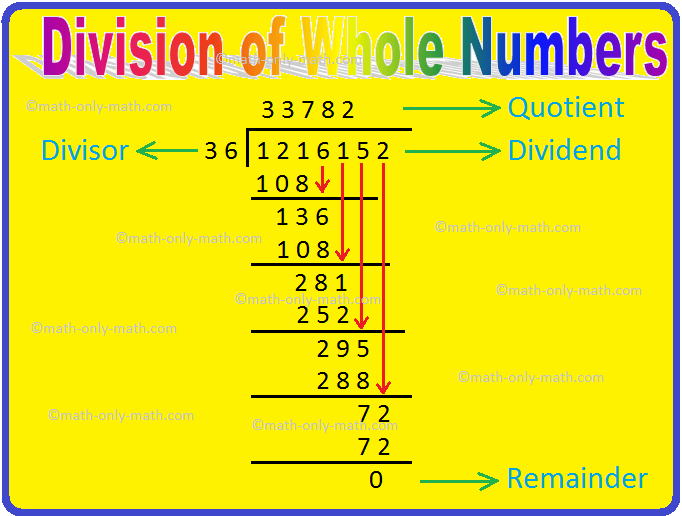
6. 36 families went on trip which cost them $1216152. If each family share the cost equally, how much did each family pay?
Solution:
Total number of families = 36
Total cost paid by 36 families = $1216152
Cost paid by each family = $1216152 ÷ 36
Hence, cost paid by each family = $33782
7. A machine produces 2175 screws a day and after a month (30 days), these screws are distributed equally to 25 dealers in different parts of the city. How many screws does each dealer get?
Solution:
Screw production per day = 2175
Screw production in 30 days = 2175 × 30 = 65,250
65,250 screws are distributed equally among 25 dealers
Therefore, number of screws per dealer 65,250 ÷ 25 = 2,610
Hence, each dealer gets 2,610 screws.
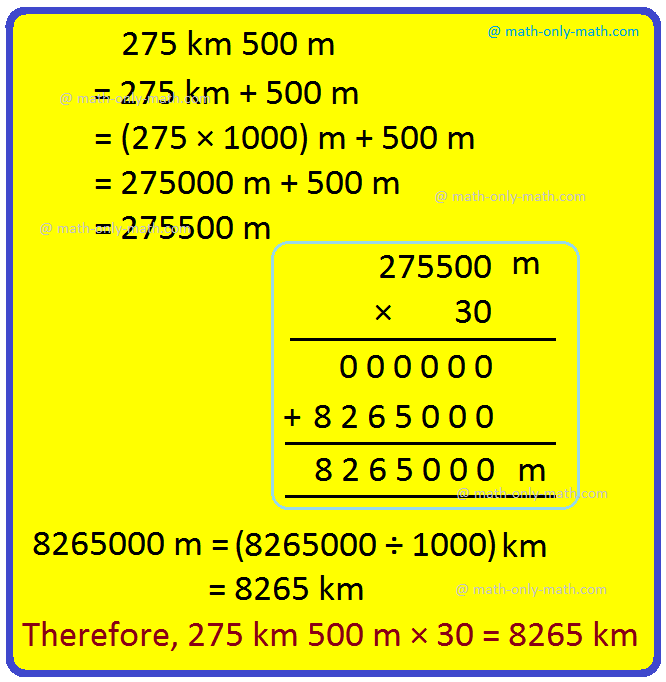
8. A bus travels a distance of 275 km 500 m daily and for this travelling it consumes 1 ℓ of diesel per 15 km. Find
(i) how much distance does the bus travel in a month of 30 days?
(ii) how much diesel does the bus consume in a month?
Solution:
(i) In 1 day, the bus travels a distance of 275 km 500 m.
In 30 days (1 month), it travels a distance of 275 km 500 m × 30.
Hence, the bus travels a distance of 8265 km in a month.
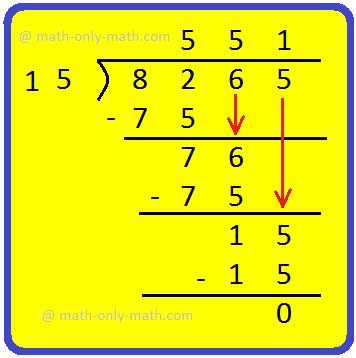
(ii) The bus travels a distance of 8265 km in a month.
The quantity of diesel consumed by the bus for 8265 km (in 1 month) is given by 8265 ÷ 15.
Hence, the bus consumes 551 ℓ of diesel in a month.
You might like these
Round off to Nearest 100 | Rounding Numbers To Nearest Hundred | Rules
While rounding off to the nearest hundred, if the digit in the tens place is between 0 – 4 i.e. < 5, then the tens place is replaced by ‘0’. If the digit in the units place is equal to or >5, then the tens place is replaced by ‘0’ and the hundreds place is increased by 1.
Round off to Nearest 10 |How To Round off to Nearest 10?|Rounding Rule
Round off to nearest 10 is discussed here. Rounding can be done for every place-value of number. To round off a number to the nearest tens, we round off to the nearest multiple of ten. A large number may be rounded off to the nearest 10. Rules for Rounding off to Nearest 10
Worksheet on H.C.F. and L.C.M. | H.C.F. by Long Division Method | Ans
We will solve different types of problems given in the Worksheet on H.C.F. and L.C.M. I. Find highest common factor of the following by complete factorisation: (i) 48, 56, 72 (ii) 198, 360 (iii) 102, 68, 136 (iv) 1024, 576 (v) 405, 783, 513
Addition of Integers | Adding Integers on a Number Line | Examples
We will learn addition of integers using number line. We know that counting forward means addition. When we add positive integers, we move to the right on the number line. For example to add +2 and +4 we move 4 steps to the right of +2. Thus, +2 +4 = +6.
Rules to Add Integers | Rules For Addition of Integers | Examples |
The rules to add integers are as follows: Rule 1: When the two integers have the positive sign, add the integers and assign the (+) sign to the sum.
What are integers? | Negative and Positive Integers | Natural Numbers
What are integers? The negative numbers, zero and the natural numbers together are called integers. A collection of numbers which is written as …….. -4, -3, -2, -1, 0, 1, 2, 3, 4……… . These numbers are called integers.
Divisibility Rules | Divisibility Test|Divisibility Rules From 2 to 18
To find out factors of larger numbers quickly, we perform divisibility test. There are certain rules to check divisibility of numbers. Divisibility tests of a given number by any of the number 2, 3, 4, 5, 6, 7, 8, 9, 10 can be perform simply by examining the digits of the
Round off to Nearest 1000 |Rounding Numbers to Nearest Thousand| Rules
While rounding off to the nearest thousand, if the digit in the hundreds place is between 0 – 4 i.e., < 5, then the hundreds place is replaced by ‘0’. If the digit in the hundreds place is = to or > 5, then the hundreds place is replaced by ‘0’ and the thousands place is
Rounding Numbers | How do you Round Numbers?|Nearest Hundred, Thousand
Rounding numbers is required when we deal with large numbers, for example, suppose the population of a district is 5834237, it is difficult to remember the seven digits and their order
Common Multiples | How to Find Common Multiples of Two Numbers?
Common multiples of two or more given numbers are the numbers which can exactly be divided by each of the given numbers. Consider the following. (i) Multiples of 3 are: 3, 6, 9, 12, 15, 18, 21, 24, …………etc. Multiples of 4 are: 4, 8, 12, 16, 20, 24, 28, …………… etc.
Common Factors | Find the Common Factor | Worksheet | Answer
Common factors of two or more numbers are a number which divides each of the given numbers exactly. For examples 1. Find the common factor of 6 and 8. Factor of 6 = 1, 2, 3 and 6. Factor
Properties of Division | Division of Property Overview|Math Properties
The properties of division are discussed here: 1. If we divide a number by 1 the quotient is the number itself. In other words, when any number is divided by 1, we always get the number itself as the quotient. For example: (i) 7542 ÷ 1 = 7542 (ii) 372 ÷ 1 = 372
Multiplication by Ten, Hundred and Thousand |Multiply by 10, 100 &1000
To multiply a number by 10, 100, or 1000 we need to count the number of zeroes in the multiplier and write the same number of zeroes to the right of the multiplicand. Rules for the multiplication by 10, 100 and 1000: If we multiply a whole number by a 10, then we write one
- Addition Of Whole Numbers.
- Word Problems on Addition and Subtraction of Whole Numbers
- Subtraction Of Whole Numbers.
- Multiplication Of Whole Numbers.
- Properties Of Multiplication.
- Division Of Whole Numbers.
- Properties Of Division.
- Word Problems on Multiplication and Division of Whole Numbers
- Worksheet on Addition and Subtraction of Large Numbers
- Worksheet on Multiplication and Division of Large Numbers
- Worksheet on Operations On Whole Numbers
From Word Problems on Multiplication and Division of Whole Numbers to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Worksheet on Addition of Capacity | Word Problems on Adding capacity
Nov 17, 24 10:30 AM
Practice the third grade math worksheet on addition of capacity. This sheet provides different types of questions where you need to arrange the values of capacity under different columns of litres and -
Measuring Capacity | Standard Unit of Capacity | Litre | Millilitres
Nov 17, 24 09:36 AM
We will discuss about measuring capacity. The milkman measures milk in liters. Petrol is given in liters. Mobil oil is sold in liters. Two milk bottles contain 1 liter of milk. One milk bottle -
Addition of Capacity | Add the Different Units of Capacity | Examples
Nov 17, 24 09:24 AM
In addition of capacity we will learn how to add the different units of capacity and volume together. While adding we need to follow that the units of capacity i.e., liter and milliliter -
Subtraction of Mass | Difference Between the Units of Mass | Examples
Nov 14, 24 09:16 AM
In subtraction of mass we will learn how to find the difference between the units of mass or weight. While subtracting we need to follow that the units of mass i.e., kilogram and gram -
Worksheet on Subtraction of Mass |Word Problems on Subtraction of Mass
Nov 13, 24 02:00 PM
Practice the third grade math worksheet on subtraction of mass or weight. This sheet provides different types of questions where you need to arrange the values of mass under different columns
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.