Reading and Interpreting a Calendar

In reading and interpreting a calendar we need to know days in a week, days in a month and months in a year.

Time is not only measured by days, hours and minutes, but also by months, weeks and years. A calendar of a year shows 12 months. February has 28 days normally. In a leap year it has 29 days. 

A month has few weeks. A week has 7 days. They are – Monday, Tuesday, Wednesday, Thursday, Friday, Saturday and Sunday.

There are 7 days in a week. The first day of the week is Sunday. 

The seven days of a week are:

(i) Sunday

(ii) Monday

(iii) Tuesday

(iv) Wednesday

(v) Thursday

(vi) Friday

(vii) Saturday

We also know that there are 12 months in a year. The first month of the year is January. 

The 12 months of the year are:

(i) January

(ii) February

(iii) March

(iv) April

(v) May

(vi) June

(vii) July

(viii) August

(ix) September

(x) October

(xi) November

(xii) December

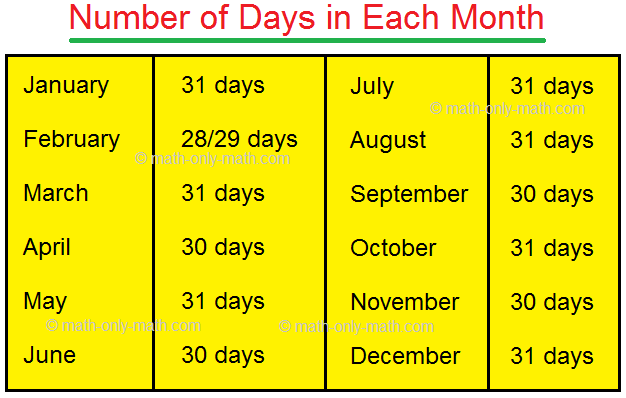
All the months have different numbers of days.

These are the number of days each month has:

(i) January = 31 days

(ii) February = 28/29 days

(iii) March = 31 days

(iv) April = 30 days

(v) May = 31 days

(vi) June = 30 days

(vii) July = 31 days

(viii) August = 31 days

(ix) September = 30 days

(x) October = 31 days

(xi) November = 30 days

(xii) December = 31 days

Thus 7 months have 31 days each; 4 months have 30 days each, only the month of February has 28 days ordinarily. But in a leap year, the month of February has 29 days.

In an ordinary year, the numbers of days are

(7 x 31) + (4 x 30) + (1 x 28)

= 217 + 120 + 28

= 365 days.

But a leap year (in which February is of 29 days) has 217 + 120 + 29 = 366 days.

To remember the number of days in different months, we may remember the following rhyme:

“Thirty days have September, April, June and November.

February alone has twenty eight, all the rest have thirty one.

But leap year coming once in four, February has one day more."

A year which is divisible by 4 is a leap year. As, 1988, 1992, 1996, 2000, 2004 all are divisible by 4, so these are leap years.

A leap year has 366 days and the month of February is of 29 days in such a year.

The calendar of a year shows all the above mentioned facts. We may gather different information with the help of a calendar.

Here is a Calendar for 2011.

We can count the number of days between the given two dates. We include the starting day and the last day in our calculation.

For Example:

1. How many days are there from 5^th March to 12^th April?

Solution:

Let us count the number of days from the given date.

Number of days in March = 31 – 4

                                     = 27 days

Number of days in April = 12

Total number of days = 27 + 12

                                = 39 days

2. Shelly went on vacation from 15^th April for 20 days. On what date will she return to work?

Solution:

Number of days remaining in April = 30 – 14

                                                  = 16 days

Number of holidays left = 20 – 16 = 4 days

4 days after 30^th April is 4^th May

She will return to work on 5^th May.

Facts about Calendar:

A calendar helps us to know the days and months of a year. So, it is also a tool to tell time. You must have seen many different kinds of calendars in the market like; wall calendars, desk calendars, etc. They may look different but they all contain the same.

🟢 A year has 12 months.

🟢 Each month is divided into weeks. A week is of 7 days.

🟢 A month has 4 weeks and a few more days.

🟢 Every fourth year is a leap year.

    For example, 2004, 2008, 2012, 2016, ...., 2024 etc.

🟢 A leap year has 366 days.

🟢 In a leap year February has 29 days.

🟢 If a year can be divisible by 4, then it is a leap year.

🟢 You can see that most months have 30 or 31 days.

🟢 February has 28 or 29 days.

Remember

A century is a leap year only when it is divided by 400. Such as year 2000 is a leap year but 2100 is not.

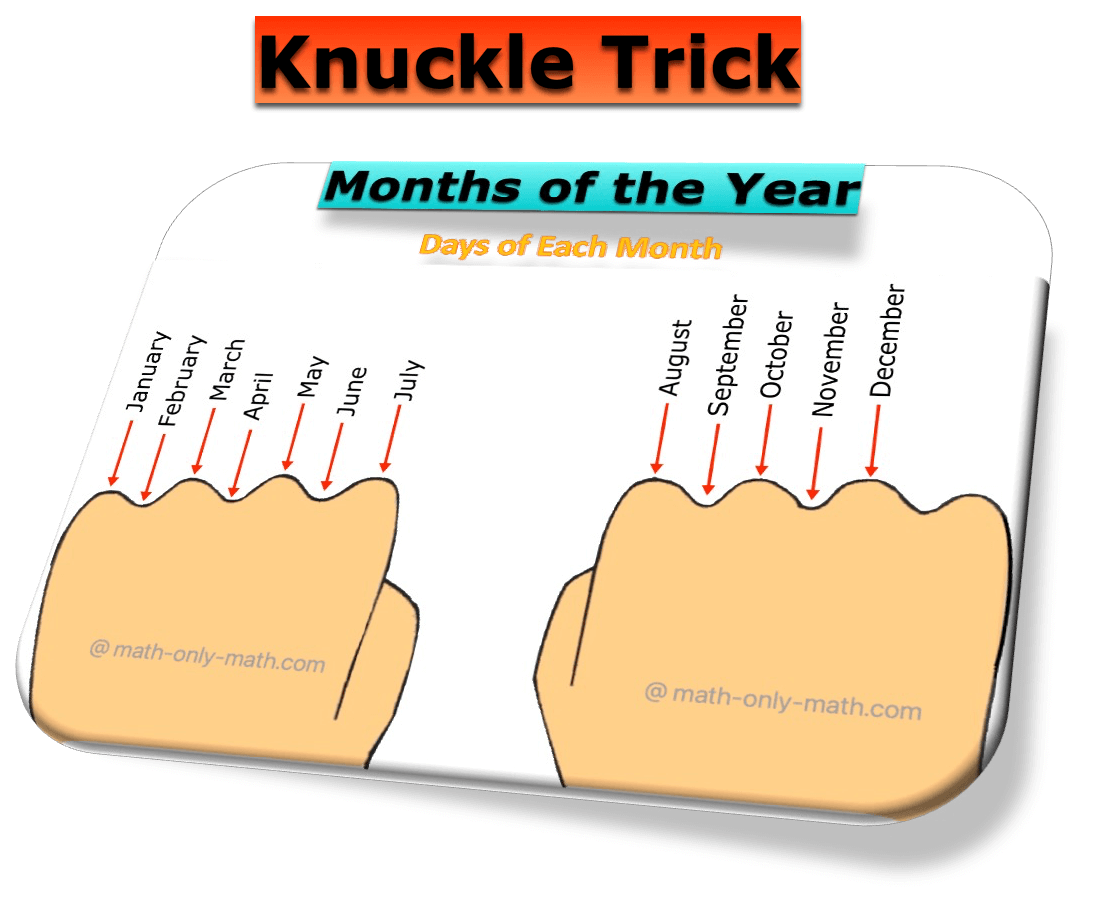
Knuckle Trick:

Close your fist. The high joints are the months with 31 days.

The lower positions show months with 30 days.

Only February has 28 or 29 days.

Writing Date:

We write date in a short form Date/Month/Year

For Example: 9 October 2025 is written as 9/10/2025.

Worksheet on Reading and Interpreting a Calendar:

Questions and answers on Interpreting a Calendar:

I. Fill in the blanks:

(i) Number of months in a year ……………….

(ii) Number of minutes in a week ……………….

(iii) Number of weeks in a year ……………….

(iv) Number of weeks and days in 45 days ……………….

(v) Number of hours in a week ……………….

(vi) Number of weeks and days in 70 days ……………….

Answers:

I. (i) 12

(ii) 10080 minutes

(iii) 52

(iv) 6 weeks 3 days

(v) 168

(vi) 10 weeks

II. Answer the following:

1. What is the date, twelve days after twentieth of September?

2. If 7 August, 2018 was a Tuesday, what was the day on 30 July, 2018?

3. Harry’s summer vacations are starting from 2^nd June. The school will open after 4 weeks 5 days. On which date is his school reopening?

4. How many months have 30 days? Name them.

5. Ron was on leave from 17 August to 4 September. Find the number of days Ron was on leave.

6. How many days are there from 25 March to 14 May?

7. How many days are there from 28 December to 3 February?

Answers:

II. 1. 2^nd October

2. Monday

3. 4^th July

4. 4, April, June, September and November

5. 19 days

6. 51

7. 38

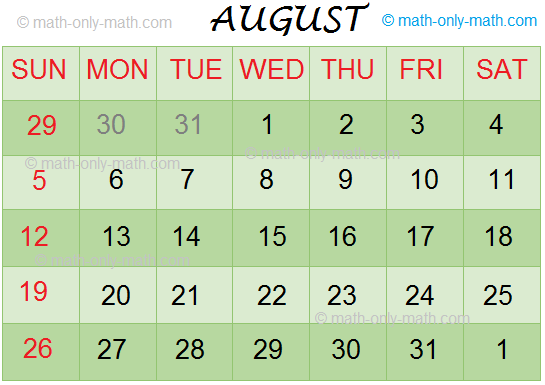
III. Observe the month of August and answer the following question

(i) How many days are there in this month?

(ii) How many Wednesdays are there in this month? Mention the dates.

(iii) Which day is on 28th of this month?

(iv) 3rd Monday is on which date?

(v) Write the date of 1st of this month?

IV: Look at the calendar of present year and answer the following questions:

(i) First Sunday in the month of January is on

(ii) August month has Mondays.

(iii) There are Sundays in the month of September.

(iv) Emily has holidays from 15th October to 17th October. She has holidays for _____ days. Her school starts on _____ which is a __________.

(v) Last Sunday in the month of December is on __________.

V: Fill in the blanks:

(i) The September month has _____ days.

(ii) There are _____ months in a year

(iii) There are _____ weeks in a year.

(iv) The January month has _____ days.

(v) There are _____ days in a week.

(vi) There are _____ days in a year.

Answer:

V: (i) 30

(ii) 12

(iii) 52

(iv) 31

(v) 7

(vi) 365/366

Related Concepts

● Units for Measuring Length

● Measuring Instruments

● To Measure the Length of a Line-segment

● Perimeter of a Figure

● Unit of Mass or Weight

● Examples on Unit of Mass or Weight

● Units for The Measurement of Capacity

● Examples on Measurement of Capacity

● Measurement of Time

● Read a Watch or a Clock

● Antemeridian (a.m.) or Postmeridian (p.m.)

● What Time it is?

● Time in Hours and Minutes

● 24 Hour Clock

● Units of Time

● Examples Units of Time

● Time Duration

● Calendar

● Reading and Interpreting a Calendar

● Calendar Guides us to Know

4th Grade Math Activities

From Reading and Interpreting a Calendar to HOME PAGE