Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.

Properties of Factors

The properties of factors are discussed step by step according to its property.

Property (1):

Every whole number is the product of 1 and itself so

(i) Each number is a factor of itself.

19 x 1 = 19,

So, 19 is the factor of 19.

(ii) 1 is the factor of every number.

We know that a number multiplied by 1 is the number itself. So, 1 is a factor of every number.

For example

21 ÷ 1 = 21. So, 1 is the factor of 21,

96 ÷ 1 = 96, So, 1 is a factor of 96.

31 x 1 = 31, So, 1 is the factor of 31.

Property (2):

Every number is a factor of zero (0)

As, 7 x 0 = 0,

17 x 0 = 0,

93 x 0 = 0

So, 7, 17, 93, ……, etc., are the factors of 0.

Property (3):

1 is the smallest factor of every number.

1 is the smallest factor of a multiple and the greatest factor of a multiple is the multiple itself.

A number is a factor of itself. SO, a number itself is its own greatest factor. For example 73 ÷ 1 = 73 so, 73 and 1 are the factors. 73 is the greatest factors.

Property (4):

Every number other than 1 has at least two factors, namely the number itself and 1.

We know that 1 and the number itself are always the factors of every number. This means that every number has at least 2 factors.

Therefore, the properties of factors are explained above so, that student can understand each property.

Properties of Factors

Property 1: A number has a finite number of factors.

Property 2: 1 and the number itself are the factors of every number.

Property 3: 1 is the smallest factor of any number.

Property 4: The number itself is the greatest factor of every number.

Property 5: Factors of a number are always smaller or equal to the number.

Property 6: Every factor of a number is the exact divisor of the number.

$Properties of Factors$

To find that whether a number is a factor of another number, we divide the bigger number by the smaller number. If the remainder is zero, we say that the divisor is a factor of the dividend.

For example:

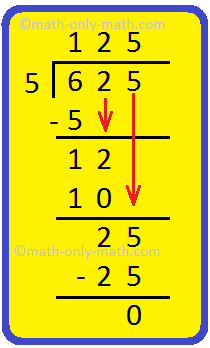
1. Is 5 a factor of 625?

$5 is a Factor of 625$

Here, 5 divides 625 exactly. So 5 is a factor of 625.

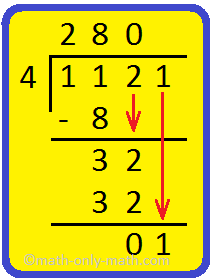
2. Is 4 a factor of 1121?

$4 is Not a Factor of 1121$

Here, 4 does not divide 1121 exactly. So 4 is not a factor of 1121.

You might like these

Check for Subtraction and Addition | Checking Subtraction | Problems
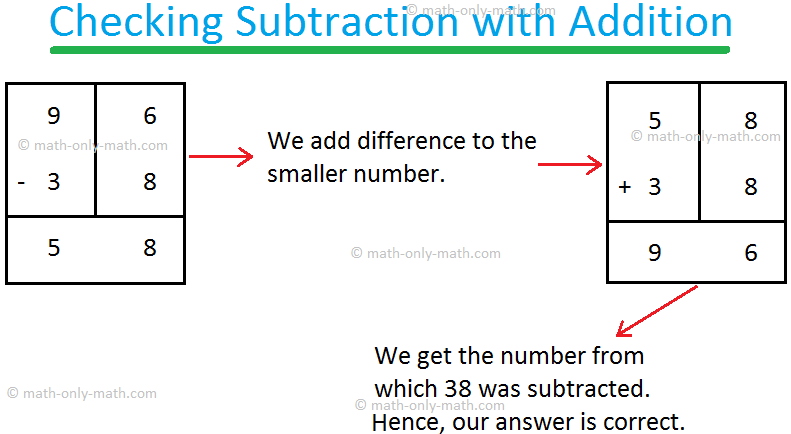
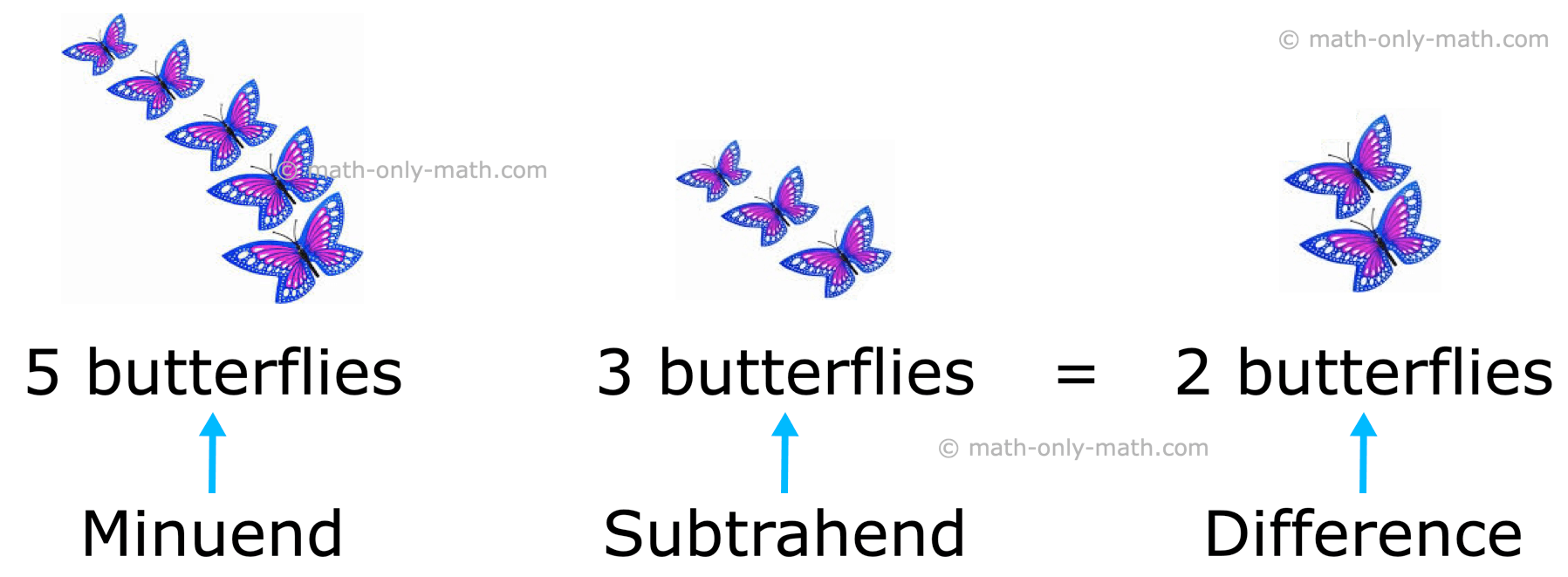
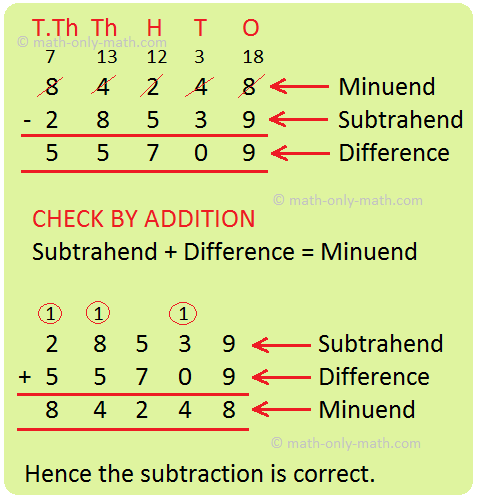
We will learn to check for subtraction and addition answers after solving. Difference of two numbers is correct when the sum of the subtrahend number and the difference is equal to the minuend.
Successor and Predecessor | Successor of a Whole Number | Predecessor
The number that comes just before a number is called the predecessor. So, the predecessor of a given number is 1 less than the given number. Successor of a given number is 1 more than the given number. For example, 9,99,99,999 is predecessor of 10,00,00,000 or we can also
Formation of Numbers | Smallest and Greatest Number| Number Formation
In formation of numbers we will learn the numbers having different numbers of digits. We know that: (i) Greatest number of one digit = 9,
Addition & Subtraction Together |Combination of addition & subtraction
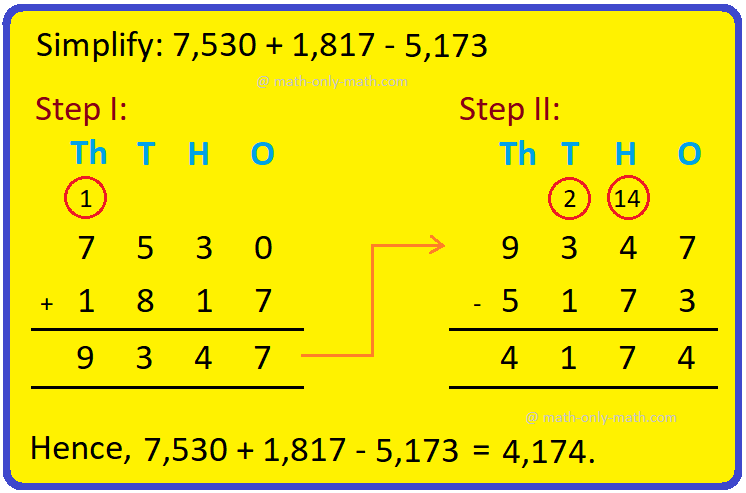
We will solve the different types of problems involving addition and subtraction together. To show the problem involving both addition and subtraction, we first group all the numbers with ‘+’ and ‘-‘ signs. We find the sum of the numbers with ‘+’ sign and similarly the sum
Examples on the Formation of Greatest and the Smallest Number |Example
In examples on the formation of greatest and the smallest number we know that the procedure of arranging the numbers in ascending and descending order.
Formation of Greatest and Smallest Numbers | Arranging the Numbers
the greatest number is formed by arranging the given digits in descending order and the smallest number by arranging them in ascending order. The position of the digit at the extreme left of a number increases its place value. So the greatest digit should be placed at the
Estimating Sums and Differences | Estimations | Practical Calculations
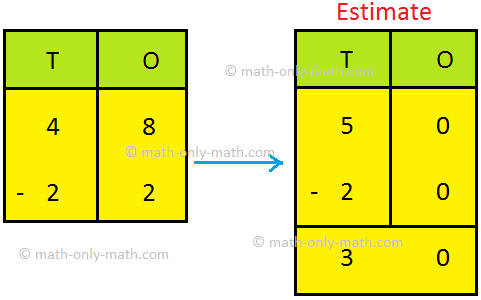
For estimating sums and differences in the number we use the rounded numbers for estimations to its nearest tens, hundred, and thousand. In many practical calculations, only an approximation is required rather than an exact answer. To do this, numbers are rounded off to a
Worksheet on Expanded form of a Number | Expanded Form of a Number
Worksheet on expanded form of a number for fourth grade math questions to practice the expanded form according to the place values of its digit. 1. Write the expanded form of the following numbers
Skip Counting Numbers | Method of Skip Counting | Skip Counting
We know skip counting numbers with smaller numbers. Now, we will learn skip counting with larger numbers. Write the number as per following instruction: 1. Write six numbers more after 2,00,000
Magic Square | Add upto 15 | Add upto 27 | Fibonacci Sequence | Videos
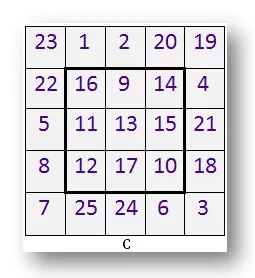
In a magic square, every row, column and each of the diagonals add up to the same total. Here is a magic square. The numbers 1 to 9 are placed in the small squares in such a way that no number is repe
Division by 10 and 100 and 1000 |Division Process|Facts about Division
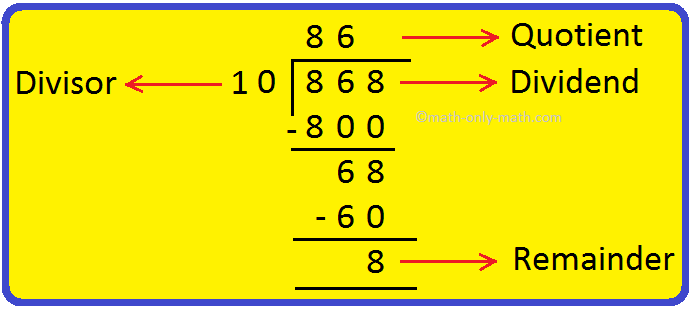
Division by 10 and 100 and 1000 are explained here step by step. when we divide a number by 10, the digit at ones place of the given number becomes the remainder and the digits at the remaining places of the number given the quotient.
Expanded Form of a Number | Writing Numbers in Expanded Form | Values
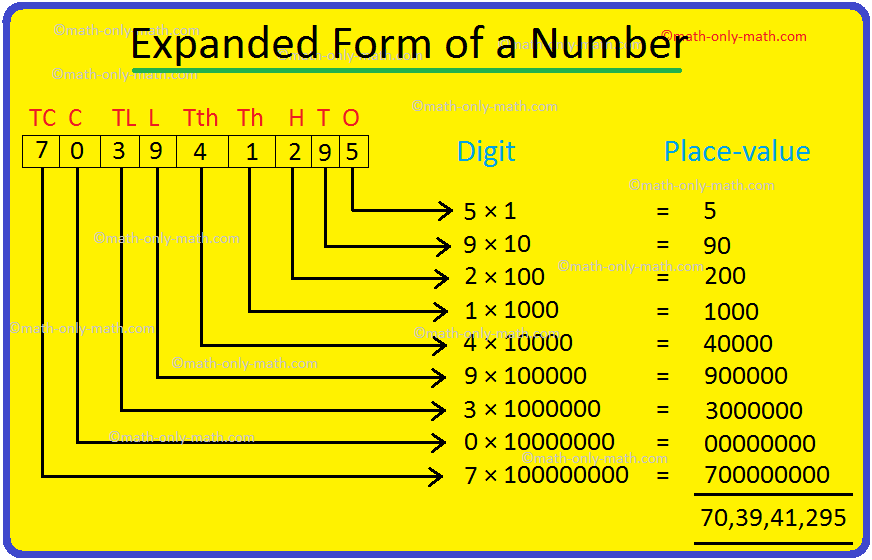
We know that the number written as sum of the place-values of its digits is called the expanded form of a number. In expanded form of a number, the number is shown according to the place values of its digits. This is shown here: In 2385, the place values of the digits are
Division by Two-Digit Numbers | Knowledge of Estimation | Division
In division by two-digit numbers we will practice dividing two, three, four and five digits by two-digit numbers. Consider the following examples on division by two-digit numbers: Let us use our knowledge of estimation to find the actual quotient. 1. Divide 94 by 12
Subtraction | How to Subtract 2-digit, 3-digit, 4-digit Numbers?|Steps
The answer of a subtraction sum is called DIFFERENCE. How to subtract 2-digit numbers? Steps are shown to subtract 2-digit numbers.
Subtraction with Regrouping | 4-Digit, 5-Digit and 6-Digit Subtraction
We will learn subtraction 4-digit, 5-digit and 6-digit numbers with regrouping. Subtraction of 4-digit numbers can be done in the same way as we do subtraction of smaller numbers. We first arrange the numbers one below the other in place value columns and then we start