Circle Passes through the Origin

We will learn how to form the equation of a circle passes through the origin.

The equation of a circle with centre at (h, k) and radius equal to a, is (x - h)\(^{2}\) + (y - k)\(^{2}\) = a\(^{2}\).

When the centre of the circle coincides with the origin i.e., a\(^{2}\) = h\(^{2}\) + k\(^{2}\)

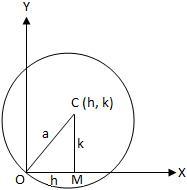
Let O be the origin and C(h, k) be the centre of the circle. Draw CM perpendicular to OX.

Circle Passes through the Origin

In triangle OCM, OC\(^{2}\) = OM\(^{2}\) + CM\(^{2}\)

i.e., a\(^{2}\) = h\(^{2}\) + k\(^{2}\).

Therefore, the equation of the circle (x - h)\(^{2}\) + (y - k)\(^{2}\) = a\(^{2}\) becomes

(x - h)\(^{2}\) + (y - k)\(^{2}\) = h\(^{2}\) + k\(^{2}\)

⇒ x\(^{2}\) + y\(^{2}\) - 2hx – 2ky = 0

The equation of a circle passing through the origin is

x\(^{2}\) + y\(^{2}\) + 2gx + 2fy = 0 ……………. (1)

or, (x - h)\(^{2}\) + (y - k)\(^{2}\) = h\(^{2}\) + k\(^{2}\) …………………………. (2)

 We clearly see that the equations (1) and (2) are satisfied by (0, 0).

Solved examples on the central form of the equation of a circle passes through the origin:

1. Find the equation of a circle whose centre is (2, 3) and passes through the origin.

Solution:

The equation of a circle with centre at (h, k) and passes through the origin is

(x - h)\(^{2}\) + (y - k)\(^{2}\) = h\(^{2}\) + k\(^{2}\)

Therefore, the required equation of the circle is (x - 2)\(^{2}\) + (y - 3)\(^{2}\) = 2\(^{2}\) + 3\(^{2}\)

⇒ x\(^{2}\) - 4x + 4 + y\(^{2}\) – 6y + 9 = 4 + 9

⇒ x\(^{2}\) + y\(^{2}\) - 4x – 6y = 0.

2. Find the equation of a circle whose centre is (-5, 4) and passes through the origin.

Solution:

The equation of a circle with centre at (h, k) and passes through the origin is

(x - h)\(^{2}\) + (y - k)\(^{2}\) = h\(^{2}\) + k\(^{2}\)

Therefore, the required equation of the circle is (x + 5)\(^{2}\) + (y - 4)\(^{2}\) = (-5)\(^{2}\) + 4\(^{2}\)

⇒ x\(^{2}\) + 10x + 25 + y\(^{2}\) – 8y + 16 = 25 + 16

⇒ x\(^{2}\)+ y\(^{2}\) + 10x – 8y = 0.

● The Circle

• Definition of Circle
• Equation of a Circle
• General Form of the Equation of a Circle
• General Equation of Second Degree Represents a Circle
• Centre of the Circle Coincides with the Origin
• Circle Passes through the Origin
• Circle Touches x-axis
• Circle Touches y-axis
• Circle Touches both x-axis and y-axis
• Centre of the Circle on x-axis
• Centre of the Circle on y-axis
• Circle Passes through the Origin and Centre Lies on x-axis
• Circle Passes through the Origin and Centre Lies on y-axis
• Equation of a Circle when Line Segment Joining Two Given Points is a Diameter
• Equations of Concentric Circles
  
• Circle Passing Through Three Given Points
• Circle Through the Intersection of Two Circles
• Equation of the Common Chord of Two Circles
• Position of a Point with Respect to a Circle
• Intercepts on the Axes made by a Circle
• Circle Formulae
• Problems on Circle

11 and 12 Grade Math 

From Circle Passes through the Origin to HOME PAGE