Proof of Compound Angle Formula
cos (α + β)

We will learn step-by-step the proof of compound angle formula cos (α + β). Here we will derive formula for trigonometric function of the sum of two real numbers or angles and their related result. The basic results are called trigonometric identities.

The expansion of cos (α + β) is generally called addition formulae. In the geometrical proof of the addition formulae we are assuming that α, β and (α + β) are positive acute angles. But these formulae are true for any positive or negative values of α and β.

Now we will prove that, cos (α + β) = cos α cos β - sin α sin β; where α and β are positive acute angles and α + β < 90°.

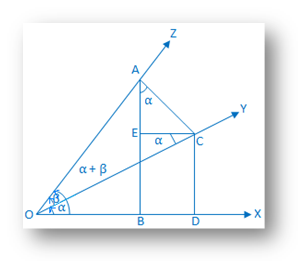
Let a rotating line OX rotate about O in the anti-clockwise direction. From starting position to its initial position OX makes out an acute ∠XOY = α.

Again, the rotating line rotates further in the same direction and starting from the position OY makes out an acute ∠YOZ = β.

Thus, ∠XOZ = α + β < 90°.    

We are suppose to prove that, cos (α + β) = cos α cos β - sin α sin β.

Construction: On the bounding line of the compound angle (α + β) take a point A on OZ, and draw AB and AC perpendiculars to OX and OY respectively. Again, from C draw perpendiculars CD and CE upon OX and AB respectively.

Proof: From triangle ACE we get, ∠EAC = 90° - ∠ACE = ∠ECO = alternate ∠COX = α.

Now, from the right-angled triangle AOB we get,

cos (α + β) = $\frac{OB}{OA}$

                = $\frac{OD - BD}{OA}$

                = $\frac{OD}{OA}$ - $\frac{BD}{OA}$

                = $\frac{OD}{OA}$ - $\frac{EC}{OA}$

                = $\frac{OD}{OC}$ ∙ $\frac{OC}{OA}$ - $\frac{EC}{AC}$ ∙ $\frac{AC}{OA}$

                = cos α cos β - sin ∠EAC sin β

                = cos α cos β - sin α sin β, (since we know, ∠EAC = α)

Therefore, cos (α + β) = cos α cos β - sin α sin β.  Proved

1. Using the t-ratios of 30° and 45°, evaluate cos 75°

Solution:

   cos 75°

= cos (45° + 30°)

= cos 45° cos 30° - sin 45° sin 30

= $\frac{1}{√2}$ ∙ $\frac{√3}{2}$ - $\frac{1}{√2}$ ∙ $\frac{1}{2}$

= $\frac{√3  -  1}{2√2}$

2. Find the values of cos 105°

Solution:

Given, cos 105°

= cos (45° + 60°)

= cos 45° cos 60° - sin 45° sin 60°

= $\frac{1}{√2}$ ∙ $\frac{1}{2}$ - $\frac{1}{√2}$ ∙ $\frac{√3}{2}$

= $\frac{1  -  √3}{2√2}$

3. If sin A = $\frac{1}{√10}$, cos B = $\frac{2}{√5}$ and A, B are positive acute angles, then find the value of (A + B).

Solution:

Since we know that, cos$^{2}$ A = 1 - sin$^{2}$ A

                                      = 1 - ($\frac{1}{√10}$)$^{2}$

                                      = 1 - $\frac{1}{10}$

                                      = $\frac{9}{10}$

                             cos A = ± $\frac{3}{√10}$

Therefore, cos A = $\frac{3}{√10}$,  (since, A is a positive acute angle)

Again, sin$^{2}$ B = 1 - cos$^{2}$ B

                  = 1 - ($\frac{2}{√5}$)$^{2}$

                  = 1 - $\frac{4}{5}$

                  = $\frac{1}{5}$

           sin B = ± $\frac{1}{√5}$              

Therefore, sin B = $\frac{1}{√5}$, (since, B is a positive acute angle)

Now, cos (A + B) = cos A cos B - sin A sin B

                        = $\frac{3}{√10}$ ∙ $\frac{2}{√5}$ - $\frac{1}{√10}$ ∙ $\frac{1}{√5}$

                        = $\frac{6}{5√2}$ - $\frac{1}{5√2}$

                        = $\frac{5}{5√2}$

                        = $\frac{1}{√2}$

    ⇒ cos (A + B) = cos π/4   

Therefore, A + B = π/4.

4. Prove that cos (π/4 - A) cos (π/4 - B) - sin (π/4 - A) sin (π/4 - B) = sin (A + B)

Solution:

L.H.S. = cos (π/4 - A) cos (π/4 - B) - sin (π/4 - A) sin (π/4 - B)

         = cos {(π/4 - A) + (π/4 - B)}

         = cos (π/4 - A + π/4 - B)

         = cos (π/2 - A - B)

         = cos [π/2 - (A + B)]

         = sin (A + B) = R.H.S.  Proved.

5. Prove that sec (A + B) = $\frac{sec A sec B}{1   -   tan A tan B}$

Solution:

L.H.S. = sec (A + B)

         = $\frac{1}{cos (A   +   B) }$

         = $\frac{1}{cos A cos  B   -   sin A sin B}$, [Applying the formula of cos (A + B)]

         = $\frac{\frac{1}{cos A cos B}}{\frac{cos A cos B}{cos A cos B}  +  \frac{sin A sin B}{cos A cos B}}$, [dividing numerator and denominator by cos A cos B]

          = $\frac{sec A sec B}{1   -   tan A tan B}$.  Proved

● Compound Angle

• Proof of Compound Angle Formula sin (α + β)
• Proof of Compound Angle Formula sin (α - β)
• Proof of Compound Angle Formula cos (α + β)
• Proof of Compound Angle Formula cos (α - β)
• Proof of Compound Angle Formula sin 22 α - sin 22 β
• Proof of Compound Angle Formula cos 22 α - sin 22 β
• Proof of Tangent Formula tan (α + β)
• Proof of Tangent Formula tan (α - β)
• Proof of Cotangent Formula cot (α + β)
• Proof of Cotangent Formula cot (α - β)
• Expansion of sin (A + B + C)
• Expansion of sin (A - B + C)
• Expansion of cos (A + B + C)
• Expansion of tan (A + B + C)
• Compound Angle Formulae
• Problems using Compound Angle Formulae
• Problems on Compound Angles
• 
  

11 and 12 Grade Math

From Proof of Compound Angle Formula cos (α + β) to HOME PAGE