Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Properties of Subtracting Integers
The properties of subtracting integers are explained here along with the examples.
1. Closure Property: The difference (subtraction) of any two integers is always an integer.
i.e., The difference of two integers is always an integer.
Hence, integers are closed under subtraction.
If x and y are any two integers, then x - y always an integer
For Examples:
(i) (+7) – (+4) = 7 - 4 = 3, which is an integer.
(ii) (-8) – (+3) = -8 – 3 = -11, which is an integer.
(iii) 6 - 14 = 6 + (-14) = - 8, which is an integer.
(iv) (- 18) - 10 = (- 18) + (- 10) = - 28, which is an integer.
2. Commutative Property: Subtraction is not commutative for integers.
For any two different integers ‘x’ and ‘y’,
x - y ≠ y - x
For Examples:
(i) 4 - 8 = 4 + (-8) = -4 and 8 - 4 = 8 + (- 4) = 4
Therefore, 4 - 8 ≠ 8 - 4
(ii) (- 4) - 7 = (- 4) + (- 7) = - 11 and 7 - (-4) = 7 + 4 = 11
Therefore, (- 4) - 7 ≠ 7 - (-4)
3. Associative Property: Subtraction is not associative for integers.
For any three integers ‘x’, ‘y’ and ‘z’,
x - (y - z) ≠ (x - y) - z
For Example:
[2 - (- 3)] - (- 6) = [2 + (3)] + 6 = 5 + 6 = 11 and
2 - [(- 3) - (- 6)] = 2 - [(- 3) + 6] = 2 - 3 = -1
Therefore, [2 - (- 3)] - (- 6) ≠ 2 - [(- 3) - (- 6)]
4. Subtraction Property of Zero: The result of subtracting zero from an integer is the integer itself.
For any three integers ‘x’,
x - 0 = x
For Example:
(i) 5 - 0 = 5
(ii) 8 - 0 = 8
(iii) 100 - 0 = 100
(iv) 9999 - 0 = 9999
(v) 99999999 - 0 = 99999999
5. For any integer ‘x’, x - 0 ≠ 0 - x
For Example:
(i) 7 - 0 = 7 and 0 - 7 = -7
Therefore, 7 - 0 ≠ 0 - 7
(ii) 10 - 0 = 10 and 0 - 10 = -10
Therefore, 10 - 0 ≠ 0 - 10
6. For any three integers ‘x’, ‘y’ and ‘z’, and x > y, then
x - z > y - z
To evaluate an expression containing various integers with plus and
minus sign:
1. Evaluate:
(i) (+15) + (-11) - (+5) - (-7)
= 15 - 11 - 5 + 7
= 22 – 16, [Adding all integers with plus (+) sign together and with minus (-) sign respectively together]
= +6 or simply 6.
(ii) (-72) + (-93) - (-85) + (+78)
= -72 -93 + 85 + 78
= -165 + 163, [Adding all integers with plus (+) sign together and with minus (-) sign respectively together]
= - 2
2. Evaluate the expression (-45) + (-32) – (-69) + (87)
Solution:
(-45) + (-32) – (-69) + (87)
= -45 – 32 + 69 +87
Add all the positive terms and add all the negative terms
= -(45 + 32) + (69 + 87)
= -77 + 156
= +79
= 79
3. Simplify: 32 – 13 + 35 + 18 - 60
Solution:
32 – 13 + 35 + 18 – 60
Add all the positive terms and add all the negative terms
= (32 + 35 + 18) – (13 + 60)
= 85 – 73
= +12 or simply 12
4. The sum of two integers is -17. If one of them is -7, find the other.
Solution:
Other integer = Sum of two integers - the given integer
= (-17) – (-7)
= -17 + 7
= -10
Therefore the other number is -10.
6th Grade Page
From Properties of Subtracting Integers to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
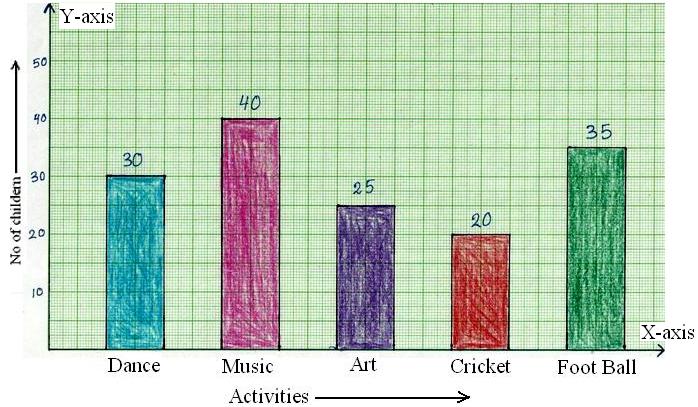
Construction of Bar Graphs | Examples on Construction of Column Graph
Jul 30, 25 03:20 PM
Now we will discuss about the construction of bar graphs or column graph. In brief let us recall about, what is bar graph? Bar graph is the simplest way to represent a data. In consists of rectangular… -
Successor and Predecessor | Successor of a Whole Number | Predecessor
Jul 29, 25 12:59 AM
The number that comes just before a number is called the predecessor. So, the predecessor of a given number is 1 less than the given number. Successor of a given number is 1 more than the given number… -
Worksheet on Area, Perimeter and Volume | Square, Rectangle, Cube,Cubo
Jul 28, 25 01:52 PM
In this worksheet on area perimeter and volume you will get different types of questions on find the perimeter of a rectangle, find the perimeter of a square, find the area of a rectangle, find the ar… -
Worksheet on Volume of a Cube and Cuboid |The Volume of a RectangleBox
Jul 25, 25 03:15 AM
We will practice the questions given in the worksheet on volume of a cube and cuboid. We know the volume of an object is the amount of space occupied by the object.1. Fill in the blanks: -
Volume of a Cuboid | Volume of Cuboid Formula | How to Find the Volume
Jul 24, 25 03:46 PM
Cuboid is a solid box whose every surface is a rectangle of same area or different areas. A cuboid will have a length, breadth and height. Hence we can conclude that volume is 3 dimensional. To measur…







New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.