Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Line Symmetry
Line symmetry is discussed here. We know that any object or shape which can be cut in two equal halves in such a way that both the parts are exactly the same is called symmetrical and the line which divides the shape in two equal halves is called the line of symmetry. A shape can have many lines of symmetry.
Let us follow some of the pictures or figures given below.
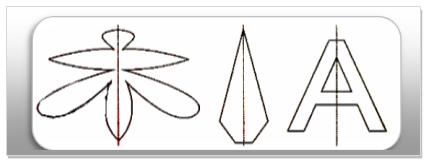
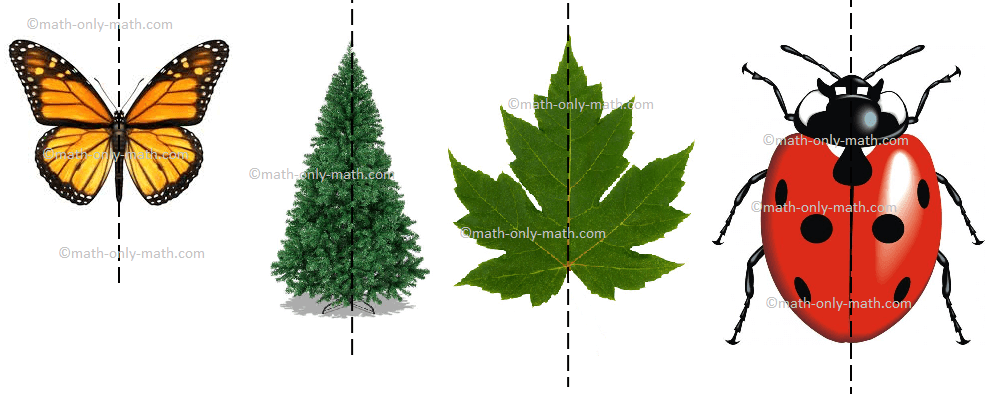
(i) Examples of vertical lines of symmetry:
Vertical Lines of Symmetry
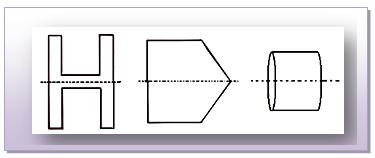
(ii) Examples of horizontal lines of symmetry:
Horizontal Lines of Symmetry
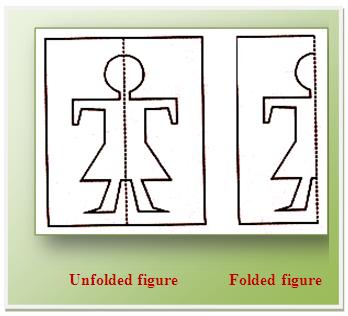
Each is divided by a straight line. Both the parts are equal in all
respects. When the pictures or figures are folded along the straight
lines, one part will fit or cover the other part exactly.
Such
pictures or figures of which either of the two parts made by a straight
line, covers the other part exactly when folded along this line, are
called symmetrical figures. The straight lines are called the lines of symmetry of the respective figures. In the above pictures or figures the lines of symmetry are both horizontal and vertical.
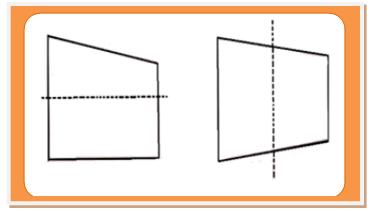
In the above figure the straight lines shown are not lines of symmetry.
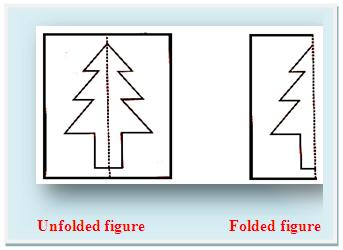
Let us consider some of the symmetrical figures which have been folded along the lines of symmetry:
(i)
(ii)
These are the basic and main points to understand line symmetry.
Worksheet on Line Symmetry:
Questions and Answers on Line Symmetry:
1. What is Line of Symmetry in Geometry?
Answer:
When a line divides a picture in the middle to get two equal halves, the halves are said to be symmetrical. The line is called the line of symmetry.
The line of symmetry can be vertical or horizontal.
2. Choose the right answer.
(i) The number of lines of symmetry in a regular pentagon are:
(a) 3
(b) 4
(c) 5
(d) 6
(ii) The number of lines of symmetry in an isosceles triangle:
(a) 1
(b) 2
(c) 3
(d) 4
Answer:
2. (i) (c)
(ii) (c)
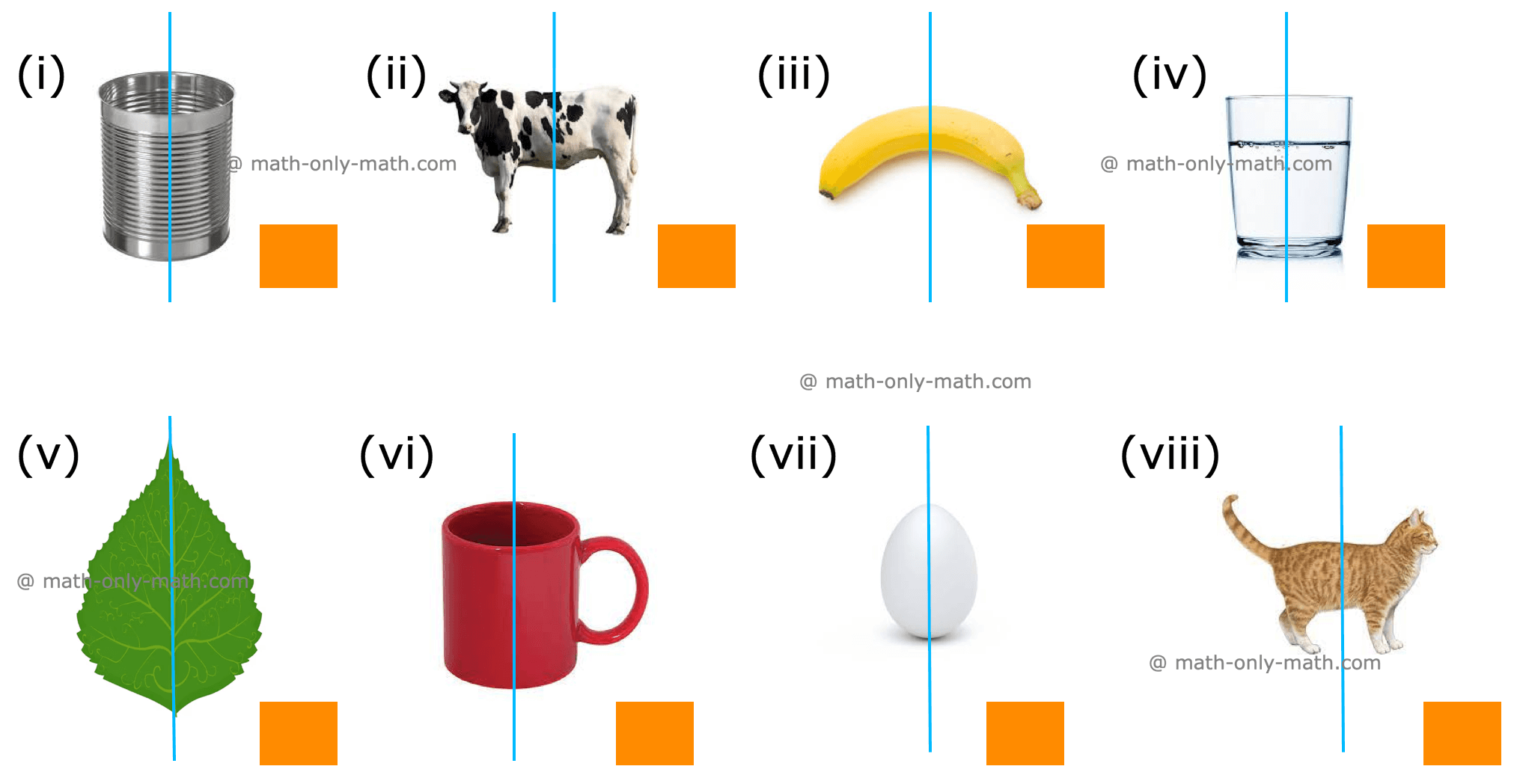
3. Tick (✔) the images which are symmetrical:
Answer:
3. (i) symmetrical
(ii) not symmetrical
(iii) not symmetrical
(iv) symmetrical
(v) symmetrical
(vi) not symmetrical
(vii) symmetrical
(viii) not symmetrical
Note: Figures through which a line can be drawn, to divide them into two equal similar halves are called symmetrical figures.
You might like these
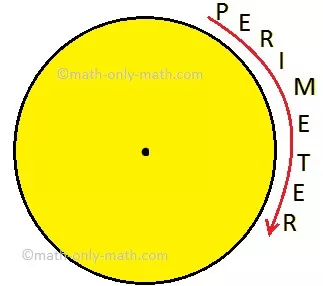
Perimeter of a figure is explained here. Perimeter is the total length of the boundary of a closed figure. The perimeter of a simple closed figure is the sum of the measures of line-segments which have surrounded the figure.
In worksheet on construction of triangles we will solve different types of questions. 1. Construct a triangle ABC in which BC = 6 cm, CA = 5 cm and AB = 4 cm.
Symmetrical shapes are discussed here in this topic. Any object or shape which can be cut in two equal halves in such a way that both the parts are exactly the same is called symmetrical. The line which divides the shape is called the symmetry. So, if we place a mirror
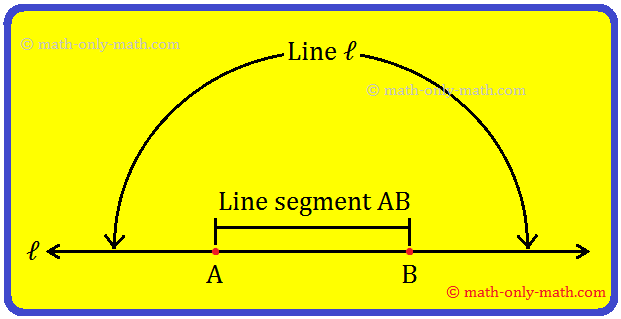
We will discuss here about constructing a line segment. We know how to draw a line segment of a certain length. Suppose we want to draw a line segment of 4.5 cm length.
We will discuss here about measuring the line segment i.e. how to draw and measure a line segment using scale or ruler. We can measure a line segment by two ways. (i) With the help of scale
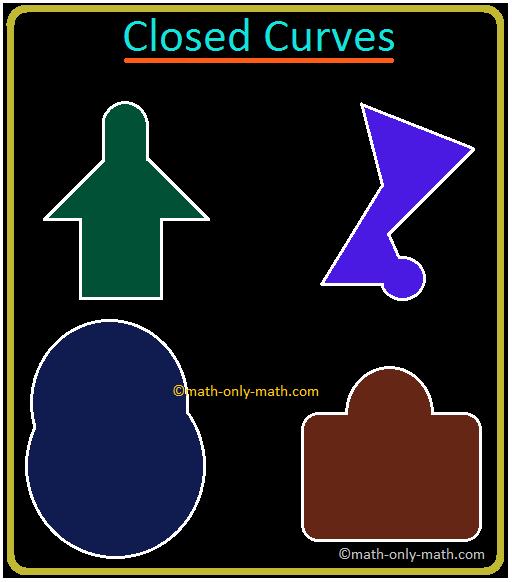
In simple closed curves the shapes are closed by line-segments or by a curved line. Triangle, quadrilateral, circle, etc., are examples of closed curves.
Worksheet on line symmetry we will solve different types of questions. 4th grade students can practice this geometry worksheet to get the basic ideas on line symmetry. Fill in the blanks: (i) A square has …... lines of symmetry. (ii) An equilateral triangle has
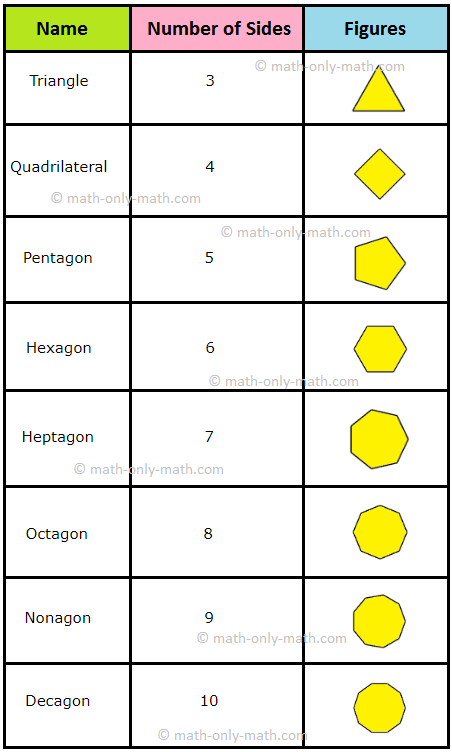
The different types of polygons are discussed here. In any polygon a pair of sides that are inclined to each other meet at a point. This point is called a vertex of the polygon. In the polygon there
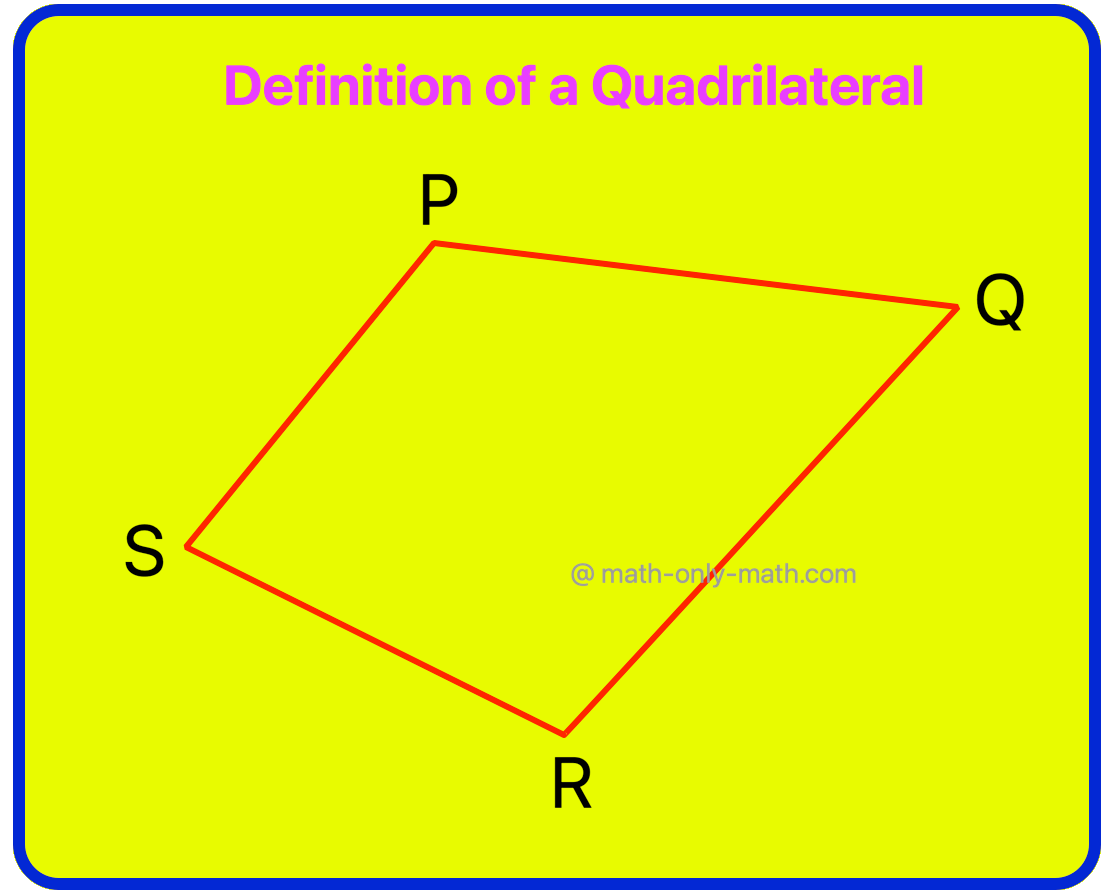
What is a quadrilateral? A simple closed curve or a polygon formed by four line-segments is called a quadrilateral.
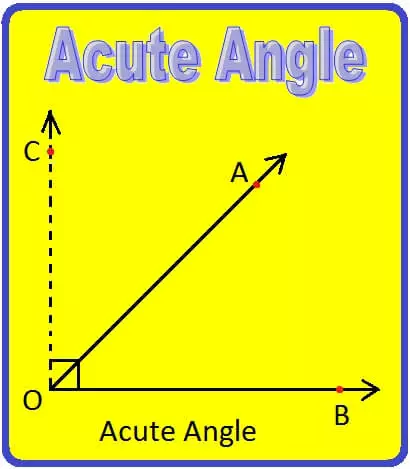
Classification of angles on the basis of their degree measures are given below: Acute angle: An angle whose measure is more than 0° but less than 90° is called an acute angle. Angles having mag
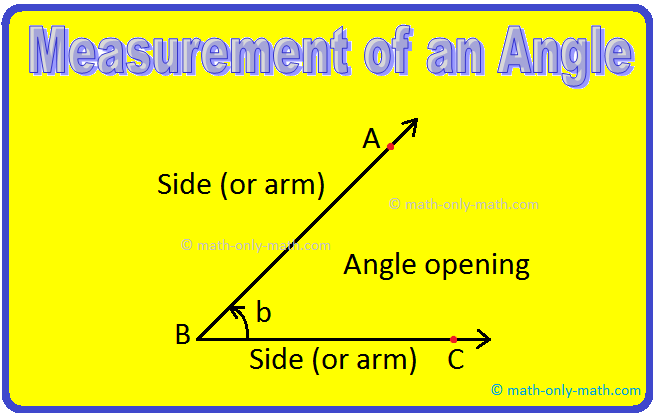
We will discuss here about measuring an angle with the help of a protractor. You can find it in your Geometry Box. It has the semi-circular shape. Its semi-circular edge is divided
We will discuss here about comparing different line segments. Different line segments can be of different lengths. We can compare the lengths of the different line segments
In circle math the terms related to the circle are discussed here. A circle is such a closed curve whose every point is equidistant from a fixed point called its centre. The symbol of circle is O. We have learned to draw a circle, by tracing the outlines of objects like a
A simple closed curve or a polygon formed by three line-segments (sides) is called a triangle. The above shown shapes are triangles. The symbol of a triangle is ∆. A triangle is a polygon with three sides. In the given figure ABC is a triangle. AB, BC and CA are its sides.
What is a polygon? A simple closed curve made of three or more line-segments is called a polygon. A polygon has at least three line-segments.
Related Concepts on Geometry - Simple Shapes & Circle
● Simple Closed Curves
● Polygon
● Different Types of Polygons
● Angle
● Triangle
● Quadrilateral
● Circle Math
● Line Symmetry
● Symmetrical Shapes
4th Grade Math Activities
From Line Symmetry to HOME PAGE
Didn't find what you were looking for? Or want to know more information
about Math Only Math.
Use this Google Search to find what you need.
Share this page:
What’s this?





New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.