Subscribe to our ▶️YouTube channel🔴 for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Division of Surds
In division of surds we need to divide a given surd by another surd the quotient is first expressed as a fraction. Then by rationalizing the denominator the required quotient is obtained with a rational denominator. For this the numerator and the denominator are multiplied by appropriate rationalizing factor. In rationalization of surds the multiplying surd-factor is called the rationalizing factor of the given surd.
Division of surds in general can be obtained by following the law of indices.
a√x÷b√x= a√xb√x= x(1a−1b).
From the above equation we can understand that if surds of rational number x are in different orders, then the indices are expressed in fraction and division is obtained by the subtraction of indices of the surds. Here surds of rational number x are in order a and b, so the indices of the surds are 1a and 1b and after division the result index of x is (1a−1b).
If the surds are in same order, then division of surds can be done by following rule.
a√x÷a√y= a√xa√y= a√xy.
From the above equation we can understand that if two or more rational numbers like x and y are in a same order a, then division of those surds can be obtained by division of the radicands or rational numbers of the surds.
In division if the surds are not in same order, we can convert them in same order to obtain the result of a division problem. But first we should try to express the surds in simplest forms and compare with other surds that they are similar surds or equiradical or dissimilar. Whatever the surds are, we can multiply the rational coefficients.
Sometimes for division of surds, we need to rationalize the denominator to get a simpler form and obtain a result. For this both numerator and denominator need to be multiplied by appropriate rationalizing factor.
Like for example 2√x2√y
= 2√x×2√y2√y×2√y
=2√xyy
In the above example 2√y is the denominator and rationalizing factor for 2√y is 2√y. So 2√y is multiplied to both the nominator and denominator to rationalize the surd.
Now we will solve some problems to understand more on division of surds:
1. Find the division of 2√12 by 2√3.
Solution:
2√12 ÷ 2√3
= 2√123
= 2√4×33
= 2√4
= 2√22
= 2.
2. Divide: √x by √y
Solution:
√x by √y
= √x ÷ √y
= √x/√y
= √xy
3. Find the division of 2√5 by 2√3.
Solution:
2√5 ÷ 2√3
= 2√52√3
= 2√5×2√32√3×2√3 ….multiplying 2√3 as rationalizing factor
= 2√153.
4. Divide the first surd by the second surd: √32, √8
Solution:
√32 divided by √8
= √32 ÷ √8
= √328
= √4
= 2.
5. Find the division of 2√3 by 2√2−1.
Solution:
2√3 ÷ 2√2−1
= 2√32√2−1
As the denominator is 2√2−1, for the division, we need to multiply it with a rationalizing factor 2√2+1.
= 2√3(2√2+1)(2√2−1)(2√2+1)
= 2√3×2√2+2√32−1….. as we know (a+b)(a−b)=a2−b2
= 2√6 + 2√3.
6. Find the quotient dividing the surd √96 by the surd √16.
Solution:
Required quotient
= √96 ÷ √16
= √9616
= √6.
7. Find the division of (x−1) by 2√x−1.
Solution:
(x−1) ÷ 2√x−1
= (x−1)2√x−1
= ((2√x)2−12)2√x−1
= ((2√x+1)(2√x−1)2√x−1….as we know a2−b2=(a+b)(a−b)
= 2√x+1.
8. Divide: √5 by √7
Solution:
√5 divided by √7
= √5 ÷ √7
= √57
= √5×√7√7×√7, [Rationalization of denominator of surds]
= √35/7.
● Surds
- Definitions of Surds
- Order of a Surd
- Equiradical Surds
- Pure and Mixed Surds
- Simple and Compound Surds
- Similar and Dissimilar Surds
- Comparison of Surds
- Addition and Subtraction of Surds
- Multiplication of Surds
- Division of Surds
- Rationalization of Surds
- Conjugate Surds
- Product of two unlike Quadratic Surds
- Express of a Simple Quadratic Surd
- Properties of Surds
- Rules of Surds
- Problems on Surds
11 and 12 Grade Math
From Division of Surds to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
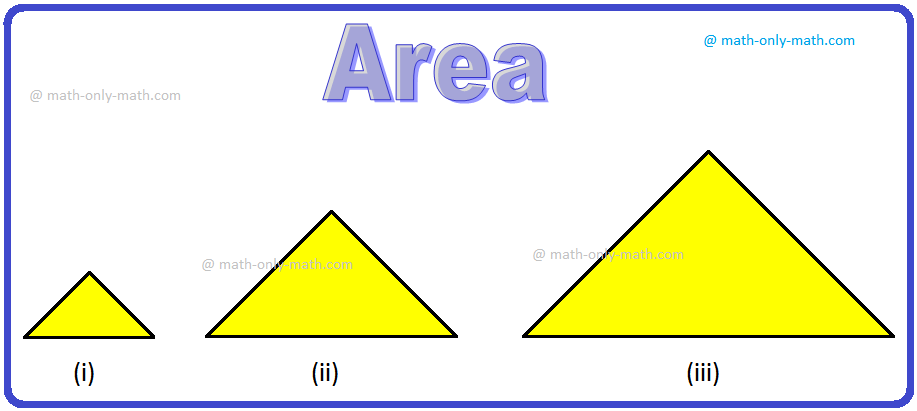
Area of Rectangle Square and Triangle | Formulas| Area of Plane Shapes
Jul 18, 25 10:38 AM
Area of a closed plane figure is the amount of surface enclosed within its boundary. Look at the given figures. The shaded region of each figure denotes its area. The standard unit, generally used for… -
What is Area in Maths? | Units to find Area | Conversion Table of Area
Jul 17, 25 01:06 AM
The amount of surface that a plane figure covers is called its area. It’s unit is square centimeters or square meters etc. A rectangle, a square, a triangle and a circle are all examples of closed pla… -
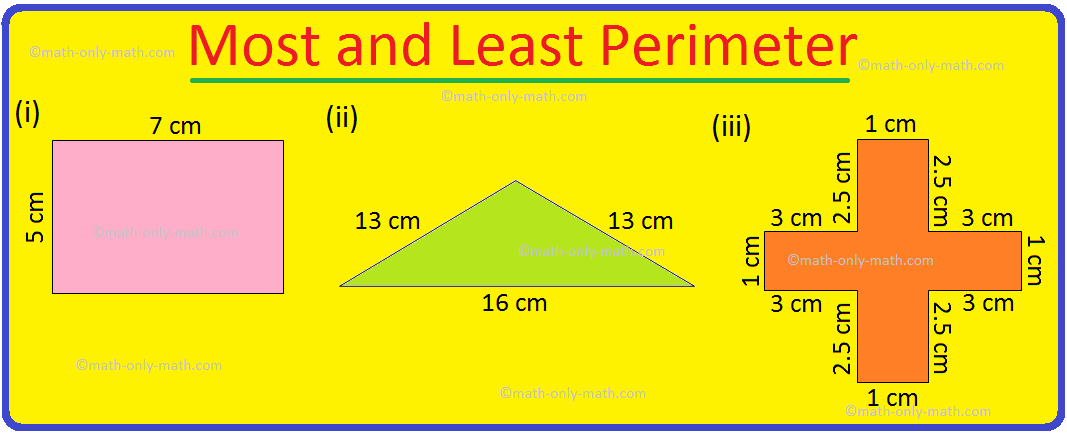
Worksheet on Perimeter | Perimeter of Squares and Rectangle | Answers
Jul 17, 25 12:40 AM
Practice the questions given in the worksheet on perimeter. The questions are based on finding the perimeter of the triangle, perimeter of the square, perimeter of rectangle and word problems. I. Find… -
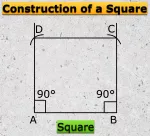
Formation of Square and Rectangle | Construction of Square & Rectangle
Jul 16, 25 11:46 PM
In formation of square and rectangle we will learn how to construct square and rectangle. Construction of a Square: We follow the method given below. Step I: We draw a line segment AB of the required… -
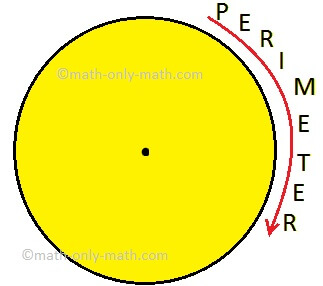
Perimeter of a Figure | Perimeter of a Simple Closed Figure | Examples
Jul 16, 25 02:33 AM
Perimeter of a figure is explained here. Perimeter is the total length of the boundary of a closed figure. The perimeter of a simple closed figure is the sum of the measures of line-segments which hav…


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.