Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Slope of the Line Joining Two Points
We will discuss here about the slope of the line joining two points.
To find the slope of a non-vertical straight line passing through two given fixed points:
Let P (x1, y1) and Q (x2, y2) be the two given points. According to the problem, the straight line PQ is non-vertical x2 ≠ x1.
Required to find, the slope of the line through P and Q.
From P, Q draw perpendiculars PM, QN on x-axis and PL ⊥ NQ. Let θ be the inclination of the line PQ, then ∠LPQ = θ.
From the above diagram, we have
PL = MN = ON - OM = x2 - x1 and
LQ = = NQ - NL = NQ - MP = y2 - y1
Therefore, the slope of the line PQ = tan θ
= LQPL
= y2−y1x2−x1
= DifferenceofordinatesofthegivenpointsDifferenceoftheirabscissae
Hence, the slope (m) of a non-vertical line passing through the points P (x1, y1) and Q (x2, y2) is given by
slope = m = y2−y1x2−x1
1. Find the slope of the line passing through the points M (-2, 3) and N (2, 7).
Solution:
Let M (-2, 3) = (x1, y1) and N (2, 7) = (x2, y2)
We know that the slope of a straight line passing through two points (x1, y1) and (x2, y2) is
m = y2−y1x2−x1
Therefore, slope of MN = y2−y1x2−x1 = 7−32+2 = 44 = 1.
2. Find the slope of the line passing through the pairs of points (-4, 0) and origin.
Solution:
We know that the coordinate of the origin is (0, 0)
Let P (-4, 0) = (x1, y1) and O (0, 0) = (x2, y2)
We know that the slope of a straight line passing through two points (x1, y1) and (x2, y2) is
m = y2−y1x2−x1
Therefore, slope of PO = y2−y1x2−x1
= 0−(00−(−4)
= 04
= 0.
● Equation of a Straight Line
- Inclination of a Line
- Slope of a Line
- Intercepts Made by a Straight Line on Axes
- Slope of the Line Joining Two Points
- Equation of a Straight Line
- Point-slope Form of a Line
- Two-point Form of a Line
- Equally Inclined Lines
- Slope and Y-intercept of a Line
- Condition of Perpendicularity of Two Straight Lines
- Condition of parallelism
- Problems on Condition of Perpendicularity
- Worksheet on Slope and Intercepts
- Worksheet on Slope Intercept Form
- Worksheet on Two-point Form
- Worksheet on Point-slope Form
- Worksheet on Collinearity of 3 Points
- Worksheet on Equation of a Straight Line
From Intercepts Made by a Straight Line on Axes to HOME
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Construction of Bar Graphs | Examples on Construction of Column Graph
Jul 30, 25 03:20 PM
Now we will discuss about the construction of bar graphs or column graph. In brief let us recall about, what is bar graph? Bar graph is the simplest way to represent a data. In consists of rectangular… -
Successor and Predecessor | Successor of a Whole Number | Predecessor
Jul 29, 25 12:59 AM
The number that comes just before a number is called the predecessor. So, the predecessor of a given number is 1 less than the given number. Successor of a given number is 1 more than the given number… -
Worksheet on Area, Perimeter and Volume | Square, Rectangle, Cube,Cubo
Jul 28, 25 01:52 PM
In this worksheet on area perimeter and volume you will get different types of questions on find the perimeter of a rectangle, find the perimeter of a square, find the area of a rectangle, find the ar… -
Worksheet on Volume of a Cube and Cuboid |The Volume of a RectangleBox
Jul 25, 25 03:15 AM
We will practice the questions given in the worksheet on volume of a cube and cuboid. We know the volume of an object is the amount of space occupied by the object.1. Fill in the blanks: -
Volume of a Cuboid | Volume of Cuboid Formula | How to Find the Volume
Jul 24, 25 03:46 PM
Cuboid is a solid box whose every surface is a rectangle of same area or different areas. A cuboid will have a length, breadth and height. Hence we can conclude that volume is 3 dimensional. To measur…
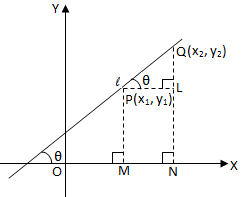





New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.