Latus Rectum of the Hyperbola

We will discuss about the latus rectum of the hyperbola along with the examples.

Definition of the latus rectum of  the hyperbola:

The chord of the hyperbola through its one focus and perpendicular to the transverse axis (or parallel to the directrix) is called the latus rectum of the hyperbola.

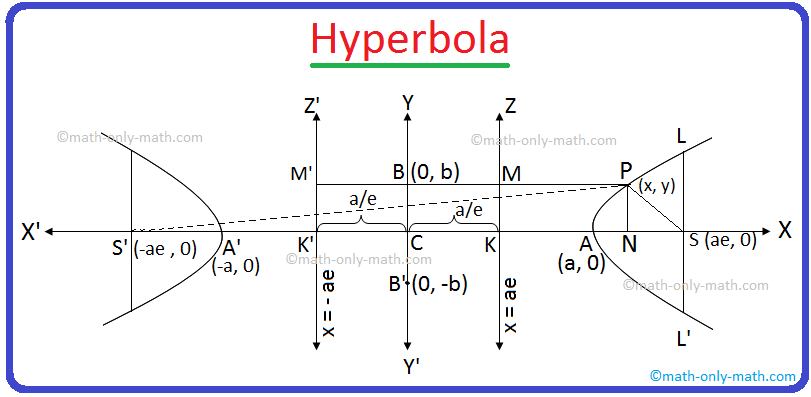
It is a double ordinate passing through the focus. Suppose the equation of the hyperbola be \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1 then, from the above figure we observe that L\(_{1}\)SL\(_{2}\) is the latus rectum and L\(_{1}\)S is called the semi-latus rectum. Again we see that M\(_{1}\)SM\(_{2}\) is also another latus rectum.

According to the diagram, the co-ordinates of the end L\(_{1}\) of the latus rectum L\(_{1}\)SL\(_{2}\) are (ae, SL\(_{1}\)). As L\(_{1}\) lies on the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1, therefore, we get,

\(\frac{(ae)^{2}}{a^{2}}\) - \(\frac{(SL_{1})^{2}}{b^{2}}\) = 1

\(\frac{a^{2}e^{2}}{a^{2}}\) - \(\frac{(SL_{1})^{2}}{b^{2}}\) = 1     

e\(^{2}\) - \(\frac{(SL_{1})^{2}}{b^{2}}\) = 1

⇒ \(\frac{(SL_{1})^{2}}{b^{2}}\) = e\(^{2}\) - 1

⇒ SL\(_{1}\)\(^{2}\) = b\(^{2}\) . \(\frac{b^{2}}{a^{2}}\), [Since, we know that, b\(^{2}\) = a\(^{2}\)(e\(^{2} - 1\))]

⇒ SL\(_{1}\)\(^{2}\) = \(\frac{b^{4}}{a^{2}}\)       

Hence, SL\(_{1}\) = ± \(\frac{b^{2}}{a}\).

Therefore, the co-ordinates of the ends L\(_{1}\) and L\(_{2}\) are (ae, \(\frac{b^{2}}{a}\)) and (ae, - \(\frac{b^{2}}{a}\)) respectively and the length of latus rectum = L\(_{1}\)SL\(_{2}\) = 2 . SL\(_{1}\) = 2 . \(\frac{b^{2}}{a}\) = 2a(e\(^{2} - 1\))

Notes:

(i) The equations of the latera recta of the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1 are x = ± ae.

(ii) A hyperbola has two latus rectum.

Solved examples to find the length of the latus rectum of a hyperbola:

Find the length of the latus rectum and equation of the latus rectum of the hyperbola x\(^{2}\) - 4y\(^{2}\) + 2x - 16y - 19 = 0.

Solution:

The given equation of the hyperbola x\(^{2}\) - 4y\(^{2}\) + 2x - 16y - 19 = 0

Now form the above equation we get,

(x\(^{2}\) + 2x + 1) - 4(y\(^{2}\) + 4y + 4) = 4

⇒ (x + 1)\(^{2}\) - 4(y + 2)\(^{2}\) = 4.

Now dividing both sides by 4

⇒ \(\frac{(x + 1)^{2}}{4}\) - (y + 2)\(^{2}\) = 1.

⇒ \(\frac{(x + 1)^{2}}{2^2} - \frac{(y + 2)^{2}}{1^{2}}\) ………………. (i)

Shifting the origin at (-1, -2) without rotating the coordinate axes and denoting the new coordinates with respect to the new axes by X and Y, we have

x = X - 1 and y = Y - 2 ………………. (ii)

Using these relations, equation (i) reduces to \(\frac{X^{2}}{2^{2}}\) - \(\frac{Y^{2}}{1^{2}}\) = 1 ………………. (iii)

This is of the form \(\frac{X^{2}}{a^{2}}\) - \(\frac{Y^{2}}{b^{2}}\) = 1, where a = 2 and b = 1.

Thus, the given equation represents a hyperbola.

Clearly, a > b. So, the given equation represents a hyperbola whose tranverse and conjugate axes are along X and Y axes respectively.

Now fine the eccentricity of the hyperbola:

We know that e = \(\sqrt{1 + \frac{b^{2}}{a^{2}}}\) = \(\sqrt{1 + \frac{1^{2}}{2^{2}}}\) = \(\sqrt{1 + \frac{1}{4}}\) = \(\frac{√5}{2}\).

Therefore, the length of the latus rectum = \(\frac{2b^{2}}{a}\) = \(\frac{2 ∙ (1)^{2}}{2}\) = \(\frac{2}{2}\) = 1.

The equations of the latus recta with respect to the new axes are X = ±ae

X = ± 2 ∙ \(\frac{√5}{2}\)

⇒ X = ± √5

Hence, the equations of the latus recta with respect to the old axes are

x = ±√5 – 1, [Putting X = ± √5 in (ii)]

i.e., x = √5 - 1 and x = -√5 – 1.

`

● The Hyperbola

• Definition of Hyperbola
• Standard Equation of an Hyperbola
  
• Vertex of the Hyperbola
• Centre of the Hyperbola
• Transverse and Conjugate Axis of the Hyperbola
• Two Foci and Two Directrices of the Hyperbola
• Latus Rectum of the Hyperbola
• Position of a Point with Respect to the Hyperbola
• Conjugate Hyperbola
• Rectangular Hyperbola
• Parametric Equation of the Hyperbola
• Hyperbola Formulae
• Problems on Hyperbola

11 and 12 Grade Math 

From Latus Rectum of the Hyperbola to HOME PAGE