Relations Between the
Trigonometric Ratios
Fundamental relations between the trigonometric ratios of an angle:
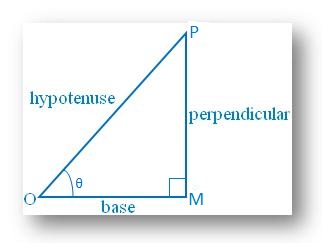
To know the relations between the trigonometric ratios from the above figure, we see;
sin θ = perpendicular/hypotenuse = MP/PO and
cosec θ = hypotenuse/perpendicular = PO/MP
It is clear that one
is the reciprocal of the other.
So, sin θ = 1/cosec θ and
cosec θ = 1/sin θ ………. (a)
Again, cos θ = base/hypotenuse = OM/OP and
sec θ = hypotenuse/ base = OP/OM
One is reciprocal of the other.
That is, cos θ = 1/sec θ and sec θ = 1/cos θ ………. (b)
So, tan θ = perpendicular/base = MP/OM and cot θ = base/perpendicular = OM/MP
tan θ = 1/cot θ and cot θ = 1/tan θ ………. (c)
Moreover, sin θ/cos θ = (MP/OP) ÷ (OM/OP) = (MP/OP) × (OP/OM) = MP/OM = tan θ
Therefore, sin θ/cos θ = tan θ ………. (d)
and cos θ/sin θ = (OM/OP) ÷ (MP/OP) = (OM/OP) × (OP/MP) = OM/MP = cot θ
Therefore, cos θ/sin θ = cot θ ………. (e)
Sin θ = PM/OP
Cos θ = OM/OP
Tan θ = PM/OM
Csc θ = OP/PM
Sec θ = OP/OM
Cot θ = OM/PM
Now from the right-angled triangle POM we get;
PM2 + OM2 = OP2 ……………. (i)
Dividing both sides by OP2 we get,
PM2/OP2 + OM2/OP2 = OP2/OP2
or, (PM/OP)2 + (OM/OP)2 = 1
or, sin2 θ + cos2 θ = 1
Again, dividing both sides of (i) by OM2
PM2/OM2 + OM2/OM2 = OP2/OM2
or, (PM/OM)2 + 1 = (OP/OM)2
or, tan2 θ + 1 = sec2 θ
Finally, dividing both of (i) by PM2 we get;
PM2/PM2 + OM2/PM2 = OP2/PM2
or, 1 + (OM/PM)2 = (OP/PM)2
or, 1 + cot2 θ = csc2 θ
Corollary 1: From the relation sin2 θ + cos2 θ = 1 we deduce that
(i) 1 - cos2 θ = sin2 θ and
(ii) 1 - sin2 θ = cos2 θ
Corollary 2: From the relation 1 + tan2 θ = sec2 θ we deduce that
(i) sec2 θ - 1 = tan2 θ and
(ii) sec2 θ - tan2 θ = 1
Corollary 3: From the relation 1 + cot2 θ = csc2 θ we deduce that
(i) csc2 θ - 1 = cot2 θ and
(ii) csc2 θ - cot2 θ = 1
This is how the ratios are related to show that one is the reciprocal of the other according to the relations between the trigonometric ratios.
Relations Between the Trigonometric Ratios
Problems on Trigonometric Ratios
Reciprocal Relations of Trigonometric Ratios
Problems on Trigonometric Identities
Elimination of Trigonometric Ratios
Eliminate Theta between the equations
Verify Trigonometric Identities
From Relations Between the Trigonometric Ratios to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Worksheet on Mixed Addition and Subtraction | Questions on Addition
Jan 12, 25 02:14 PM
In worksheet on mixed addition and subtraction the questions involve both addition and subtraction together; all grade students can practice the questions on addition and subtraction together. -
Estimating Sums and Differences | Estimations | Practical Calculations
Jan 12, 25 02:02 PM
For estimating sums and differences in the number we use the rounded numbers for estimations to its nearest tens, hundred, and thousand. In many practical calculations, only an approximation is requir… -
Combination of Addition and Subtraction | Mixed Addition & Subtraction
Jan 12, 25 01:36 PM
We will discuss here about the combination of addition and subtraction. The rules which can be used to solve the sums involving addition (+) and subtraction (-) together are: I: First add -
Checking Subtraction using Addition |Use Addition to Check Subtraction
Jan 12, 25 01:13 PM
We can check subtraction by adding the difference to the smaller number. Since the sum of difference and smaller number is equal to the larger number, subtraction is correct. -
Worksheet on Subtraction of 4-Digit Numbers|Subtracting 4-Digit Number
Jan 12, 25 09:04 AM
Practice the questions given in the worksheet on subtraction of 4-digit numbers. Here we will subtract two 4-digit numbers (without borrowing and with borrowing) to find the difference between them.
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.