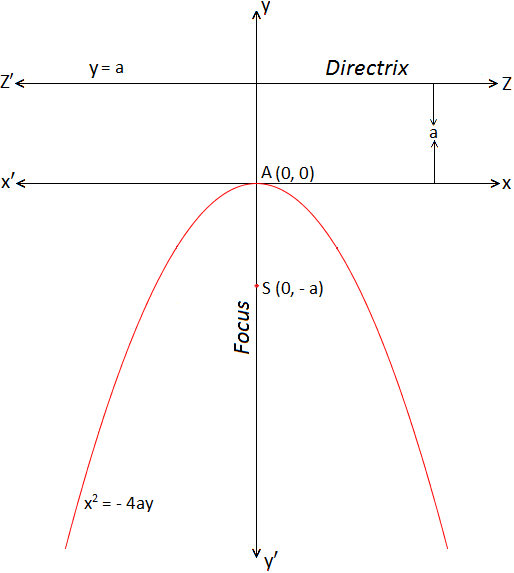
Standard form of Parabola x\(^{2}\)= -4ay

We will discuss about the standard form of parabola x\(^{2}\) = -4ay

Equation y\(^{2}\) = -4ax (a > 0) represents the equation of a parabola whose co-ordinate of the vertex is at (0, 0), the co-ordinates of the focus are (0, -a), the equation of directrix is y = a or y - a = 0, the equation of the axis is x = 0, the axis is along negative y-axis, the length of its latus rectum = 4a and the distance between its vertex and focus is a.

Solved examples based on the standard form of parabola x\(^{2}\) = -4ay:

1. Find the axis, co-ordinates of vertex and focus, length of latus rectum and the equation of directrix of the parabola x\(^{2}\) = -16y

Solution:

The given parabola x\(^{2}\) = -16y

⇒ x\(^{2}\) = -4 ∙ 4 y

Compare the above equation with standard form of parabola x\(^{2}\) = -4ay, we get, a = 4.

Therefore, the axis of the given parabola is along negative y-axis and its equation is x = 0

The co-ordinates of its vertex are (0, 0) and the co-ordinates of its focus are (0, -4); the length of its latus rectum = 4a = 4 ∙ 4 = 16 units and the equation of its directrix is y = a i.e., y = 4 i.e., y - 4 = 0.

2. Find the axis, co-ordinates of vertex and focus, length of latus rectum and the equation of directrix of the parabola 3x\(^{2}\) = -8y

Solution:

The given parabola 3x\(^{2}\) = -8y

⇒ x\(^{2}\) = -\(\frac{8}{3}\)y

⇒ x\(^{2}\) = -4 ∙ \(\frac{2}{3}\) y

Compare the above equation with standard form of parabola x\(^{2}\) = -4ay, we get, a = \(\frac{2}{3}\).

Therefore, the axis of the given parabola is along negative y-axis and its equation is x = 0

The co-ordinates of its vertex are (0, 0) and the co-ordinates of its focus are (0, -\(\frac{2}{3}\)); the length of its latus rectum = 4a = 4 ∙ \(\frac{2}{3}\) = \(\frac{8}{3}\) units and the equation of its directrix is y = \(\frac{2}{3}\) i.e., 3y = 2 i.e., 3y - 2 = 0.

● The Parabola

• Concept of Parabola
• Standard Equation of a Parabola
• Standard form of Parabola y22 = - 4ax
• Standard form of Parabola x22 = 4ay
• Standard form of Parabola x22 = -4ay
• Parabola whose Vertex at a given Point and Axis is Parallel to x-axis
• Parabola whose Vertex at a given Point and Axis is Parallel to y-axis
• Position of a Point with respect to a Parabola
  
• Parametric Equations of a Parabola
• Parabola Formulae
• Problems on Parabola

11 and 12 Grade Math 

From Standard form of Parabola x^2 = -4ay to HOME PAGE