Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Thousandths Place in Decimals
When we write a decimal number with three places, we are
representing the thousandths place. Each part in the given figure represents
one-thousandth of the whole.
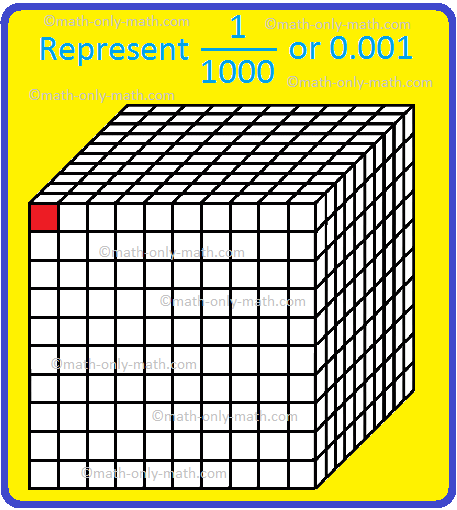
● Look at the given figure, which is divide into 100 equal parts, Each part is further divided into 10 equal parts, i.e., a square is divided into 1000 parts and out of it, 1 part is shaded. We say 11000 part is shaded.
It is written as 11000. In the decimal form it is written as 0.001. It is read as 'zero point zero zero one' or 'one thousandth'.
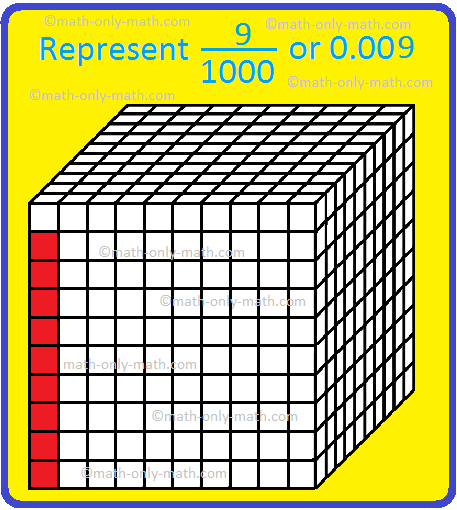
● If we take 9 parts out of 1000 equal parts of an object, then 9 parts make 9/1000 of the whole and it is written as 0.009
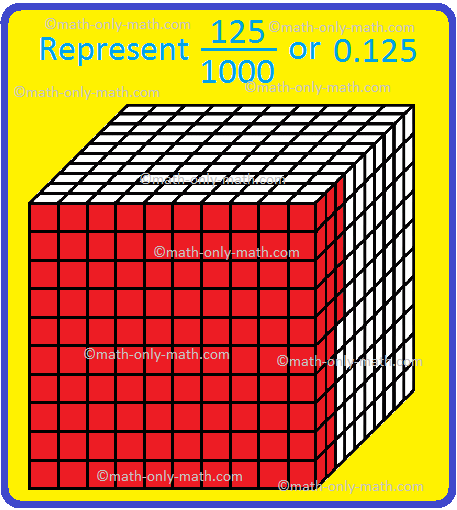
● Let us represent 1251000.
In the given figure 125 parts of 1000 equal parts are
colored. We write this as 0.125 in decimal form, where 1 represents 1 tenths, 2
represents 2 hundredths and 5 represents 5 thousandths. So, in the place-value
chart 1 is written in the tenths column, 2 is written in the hundredth column
and 5 is written in the thousandth column.
Similarly, we write We can also write, 471000, 1871000, 8971000 as 0.047, 0.187 and 0.897 respectively.
We can also write, \(\frac{4653}{1000}\) = 4.653, 570251000 = 57.025 and so on.
From the above discussion, we observe that a fraction in the form \frac{\textrm{number}}}{1000} is written as a decimal obtained by putting decimal point to the left of three right-most digits. If the number is short of digits, we insert zeros to the left of the number.
Decimal Places
You might like these
Rounding decimals are frequently used in our daily life mainly for calculating the cost of the items. In mathematics rounding off decimal is a technique used to estimate or to find the approximate
Word problems on decimals are solved here step by step. The product of two numbers is 42.63. If one number is 2.1, find the other. Solution: Product of two numbers = 42.63 One number = 2.1
Practice the math questions given in the worksheet on dividing decimals. Divide the decimals to find the quotient, same like dividing whole numbers. This worksheet would be really good for the students to practice huge number of decimal division problems.
Practice the math questions given in the worksheet on multiplying decimals. Multiply the decimals to find the product of the two decimal numbers, same like multiplying whole numbers.
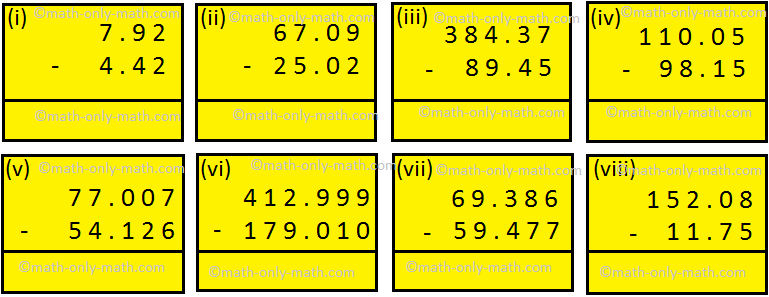
We will practice the questions given in the worksheet on subtraction of decimal fractions. While subtracting the decimal numbers convert them into like decimal then subtract as usual ignoring decimal point and then put the decimal point in the difference directly under the
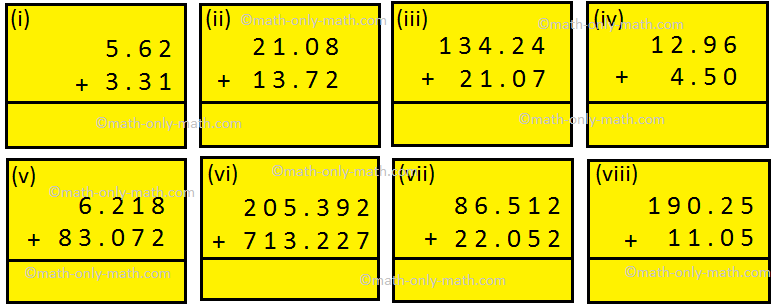
We will practice the questions given in the worksheet on addition of decimal fractions. While adding the decimal numbers convert them into like decimal then add as usual ignoring decimal point and then put the decimal point in the sum directly under the decimal points of all
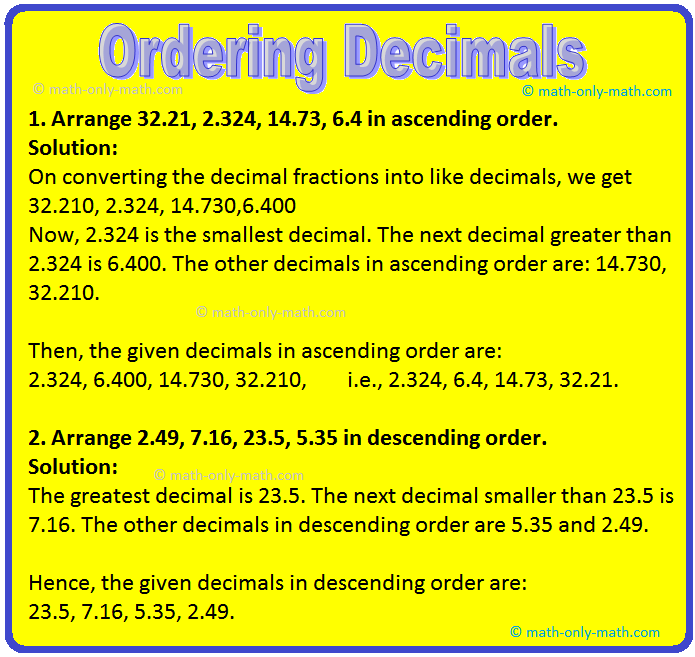
In ordering decimals we will learn how to compare two or more decimals. (i) Convert each of them as like decimals. (ii) Compare these decimals just as we compare two whole numbers ignoring
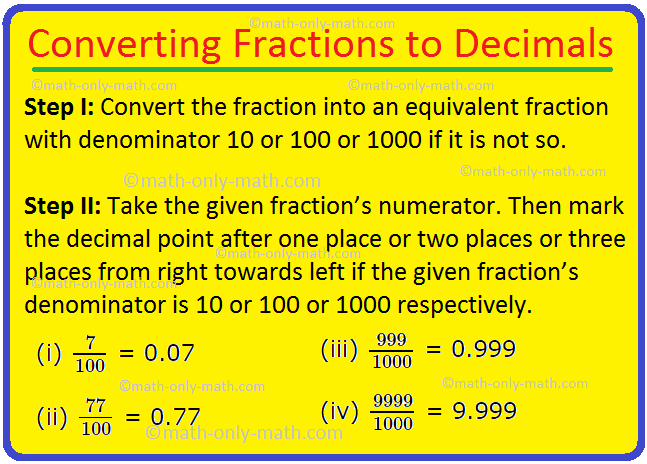
In converting fractions to decimals, we know that decimals are fractions with denominators 10, 100, 1000 etc. In order to convert other fractions into decimals, we follow the following steps:
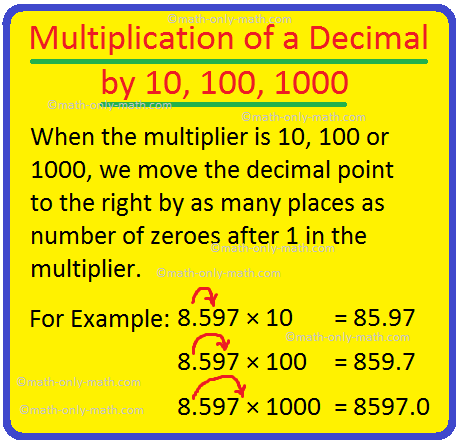
The working rule of multiplication of a decimal by 10, 100, 1000, etc... are: When the multiplier is 10, 100 or 1000, we move the decimal point to the right by as many places as number of zeroes after 1 in the multiplier.
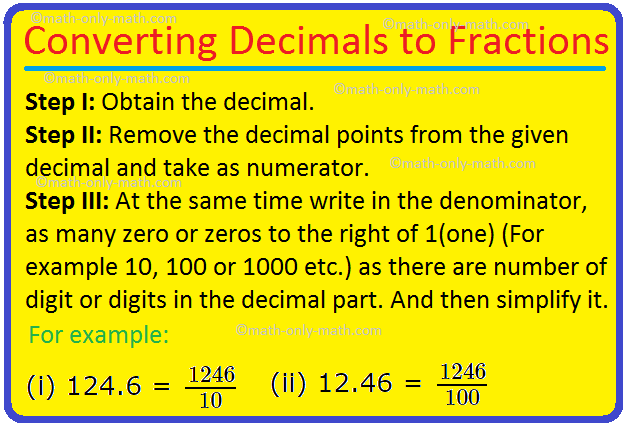
In converting decimals to fractions, we know that a decimal can always be converted into a fraction by using the following steps: Step I: Obtain the decimal. Step II: Remove the decimal points from the given decimal and take as numerator.
Dividing Decimals by Decimals I. Dividing a Decimal by a Whole Number: II. Dividing a Decimal by another Decimal: If the dividend and divisor are both decimal numbers, we multiply both the numbers by 10, 100, 1000, etc so that the divisor becomes a whole number
Division of a decimal by a decimal are discussed here. 1. 14.7 ÷ 2.1 Solution: 14.7 ÷ 2.1 [Count the number of decimal digits in the divisor and we observe that after decimal there is 1
Decimal numbers can be expressed in expanded form using the place-value chart. In expanded form of decimal fractions we will learn how to read and write the decimal numbers. Note: When a decimal is missing either in the integral part or decimal part, substitute with 0.
In dividing decimals word problems worksheet we will get different types of problems on decimals division word problems, dividing a decimal by a whole number, dividing a decimals and dividing a decimals by 10, 100 and 1000.
Multiplying decimal by a whole number is just same like multiply as usual. How to multiply a decimal by a whole number? To multiply a decimal by a whole number follow the below steps
5th Grade Numbers
5th Grade Math Problems
From Thousandths Place in Decimals to HOME PAGE
Didn't find what you were looking for? Or want to know more information
about Math Only Math.
Use this Google Search to find what you need.
Share this page:
What’s this?







New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.