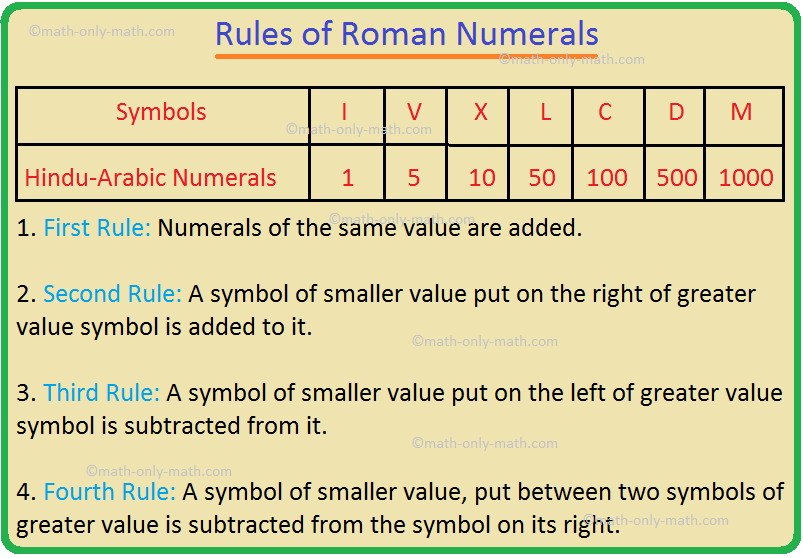
Rules for Formation of Roman-Numerals

The Roman numerals system, developed by the Romans thousand of years ago, is based on seven letters of the alphabet. All the numbers are written using either one of the letters or combinations of letters.

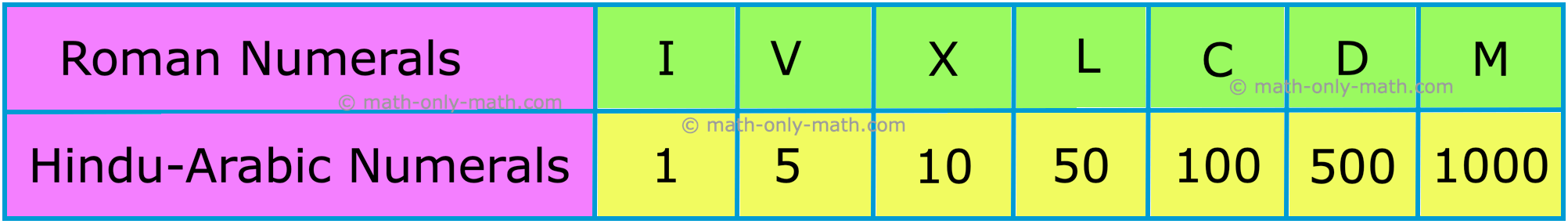
The seven letters used in the Roman numeral system.

Each letter has a value when compared with the Hindu-Arabic numerals. Observe the following table:

In rules for formation of Roman-numerals system there is no symbol or digit for zero. This system has no place value system. The digit or digits of lower value is/are placed after or before the digit of higher value. The value of digits of lower value is added to or subtracted from the value of digit of higher value. Using the certain rules for formation of Roman-numerals is given below.

Rule 1: The roman digits I, X and C are repeated upto three times in succession to form the numbers.

(a) We know the value of I = 1, value of X is 10 and value of C is 100.

(b) The value of I, X and C are added as:

(c) No digit is repeated in succession more than thrice, i.e., I, X and C cannot be repeated more than 3 times.

(d) The digits V, L and D are not repeated. The repetition of V, L and D is invalid in the formation of numbers.

Rule 2: (a) When a digit of lower value is written to the right or after a digit of higher value, the values of all the digits are added. As:

(b) Value of similar digits are also added as indicated in rule 1

Rule 3: When a digit of lower value is written to the left or before a digit of higher value, then the value of the lower digit is subtracted from the value of the digit of higher value. As:

However, V is never written to the left of X.

Rule 4: (a) If we have to write the numbers beyond 10 we should write the number 10 or groups of number 10 and then number 1 or 5 as the case may be. Then these numbers are used to change to the corresponding Roman numerals. As:

12 = 10 + 2 = 1 0 + 1 + 1 = XII

20 = 10 + 10 = XX

22 = 10 + 10 +1 + 1= XXII

26 = 10 + 10 + 5 + 1 = XXVI

39 = 10 + 10 + 10 + (10 - 1) = XXXIX

37 = 10 + 10 + 10 + 5 + 1 + 1 = XXXVII

(b) According to this pattern, numbers higher than number 40 are also formed:

43 = (50 - 10) + 1 +1 + 1= XLIII

56 = 50 + 5 + 1 = LVI

59 = 100 + 50 + (10 - 1) = CLIX

1238 = 1000 + 100 + 100 + 10 + 10 + 10 + 5 + 1 + 1 + 1 = MCCXXXVIII

Rule 5: If a horizontal line is drawn over the symbols or digits of Roman numerals, then the value of the numerals becomes 1000 times. As:

For example:

1. Write the Roman numerals for the following numbers:

(i) 13 = XIII

(ii) 14 = XIV

(iii) 18 = XVIII

(iv) 26 = XXVI

(v) 39 = XXXIX

(vi) 42 = XLII

(vii) 61 = LXI

(viii) 545 = DXLV

(ix) 217 = CCXVII

2. Write the numbers for the following Roman numerals:

(i) VII = 7

(ii) XXXIV = 34

(iii) XXXVII = 37

(iv) XLIII = 43

(v) XLVIII = 48

(vi) LII = 52

(vii) CXL = 140

(viii) CXLV = 145

These are the five rules for formation of Roman-numerals system explained using examples.

REMEMBER: Note that there is no in the Roman system. This system does not use place value to write the numbers

Solved Examples on Rules for Formation of Roman-Numerals:

1. Write in the Hindu-Arabic system.

(i) XXIV

(ii) XXV

(iii) XXXIX

Solution:

(i) XXIV = 10 + 10 + 4 = 24

(ii) XXV = 10 + 10 + 5 = 25

(ii) XXXIX = 10 + 10 + 10 + 10 - 1 = 39

REMEMBER:

Using the symbols I, V, X we can write numbers up to 39.

      Roman Numerals up to 39:

Worksheet on Rules for Formation of Roman-Numerals:

1. Write the answers about yourself in Roman numerals:

(i) My date of birth is __________. (ii) l am __________ years old. (iii) The number of the month I was born in is __________. (iv) I study in class __________.  (v) My father is __________ years old. (vi) My mother is __________ years old.

2. Write True or False:

(i) The Roman numerals for 26 is XXV.

(ii) The roman numeral I can be repeated a maximum of three times.

(iii) 34 can be written in Roman numeral as XXXIX.

(iv) The Roman Numeral XXVII represents the number 27.

Answer:

2. (i) False

(ii) True

(iii) False

(iv) True

3. Match the Numbers Column with Roman Numerals Column: 

Answer:

3. (i) → (e)

(ii) → (f)

(iii) → (g)

(iv) → (h)

(v) → (c)

(vi) → (b)

(vii) → (a)

(viii) → (d)

4. Write the number name for each of the following. Also write the corresponding Hindu-Arabic numeral:

Answer:

4. (i) sixteen     →     16

(ii) seven     →     7

(iii) thirty four     →     34

(iv) fourteen     →     14

(v) thirty nine     →     39

(vi) thirty five     →     35

(vii) nineteen     →     19

(viii) thirty one     →     31

(ix) thirty     →     30

(x) eighteen     →     18

Related Concept

● Roman Numerals

● Rules for Formation of Roman-numerals

● Uses of Roman Numerals

From Rules for Formation of Roman-numerals to HOME PAGE