Subscribe to our YouTube channel for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Basic Math Formulas
The list of basic math formulas which is very useful for mainly 11 grade, 12 grade and college grade students. Math formulas are very important and necessary to know the correct formula while solving the questions on different topics. If we remember math formulas we can solve any type of math questions.
LIST OF IMPORTANT MATH FORMULAS AND RESULTS
Algebra:
● Laws of Indices:
(i) aᵐ ∙ aⁿ = aᵐ + ⁿ
(ii) aᵐ/aⁿ = aᵐ - ⁿ
(iii) (aᵐ)ⁿ = aᵐⁿ
(iv) a = 1 (a ≠ 0).
(v) a-ⁿ = 1/aⁿ
(vi) ⁿ√aᵐ = aᵐ/ⁿ
(vii) (ab)ᵐ = aᵐ ∙ bⁿ.
(viii) (a/b)ᵐ = aᵐ/bⁿ
(ix) If aᵐ = bᵐ (m ≠ 0), then a = b.
(x) If aᵐ = aⁿ then m = n.
● Surds:
(i) The surd conjugate of √a + √b (or a + √b) is √a - √b (or a - √b) and conversely.
(ii) If a is rational, √b is a surd and a + √b (or, a - √b) = 0 then a = 0 and b = 0.
(iii) If a and x are rational, √b and √y are surds and a + √b = x + √y then a = x and b = y.
● Complex Numbers:
(i) The symbol z = (x, y) = x + iy where x, y are real and i = √-1, is called a complex (or, imaginary) quantity;x is called the real part and y, the imaginary part of the complex number z = x + iy.
(ii) If z = x + iy then z = x - iy and conversely; here, z is the complex conjugate of z.
(iii) If z = x+ iy then
(a) mod. z (or, | z | or, | x + iy | ) = + √(x² + y²) and
(b) amp. z (or, arg. z) = Ф = tan−1 y/x (-π < Ф ≤ π).
(iv) The modulus - amplitude form of a complex quantity z is
z = r (cosф + i sinф); here, r = | z | and ф = arg. z (-π < Ф <= π).
(v) | z | = | -z | = z ∙ z = √ (x² + y²).
(vi) If x + iy= 0 then x = 0 and y = 0(x,y are real).
(vii) If x + iy = p + iq then x = p and y = q(x, y, p and q all are real).
(viii) i = √-1, i² = -1, i³ = -i, and i⁴ = 1.
(ix) | z₁ + z₂| ≤ | z₁ | + | z₂ |.
(x) | z₁ z₂ | = | z₁ | ∙ | z₂ |.
(xi) | z₁/z₂| = | z₁ |/| z₂ |.
(xii) (a) arg. (z₁ z₂) = arg. z₁ + arg. z₂ + m
(b) arg. (z₁/z₂) = arg. z₁ - arg. z₂ + m where m = 0 or, 2π or, (- 2π).
(xiii) If ω be the imaginary cube root of unity then ω = ½ (- 1 + √3i) or, ω = ½ (-1 - √3i)
(xiv) ω³ = 1 and 1 + ω + ω² = 0
● Variation:
(i) If x varies directly as y, we write x ∝ y or, x = ky where k is a constant of variation.
(ii) If x varies inversely as y, we write x ∝ 1/y or, x = m ∙ (1/y) where m is a constant of variation.
(iii) If x ∝ y when z is constant and x ∝ z when y is constant then x ∝ yz when both y and z vary.
● Arithmetical Progression (A.P.):
(i) The general form of an A. P. is a, a + d, a + 2d, a + 3d,.....
where a is the first term and d, the common difference of the A.P.
(ii) The nth term of the above A.P. is t₀ = a + (n - 1)d.
(iii) The sum of first n terns of the above A.P. is s = n/2 (a + l) = (No. of terms/2)[1st term + last term]
or, S = ⁿ/₂ [2a + (n - 1) d]
(iv) The arithmetic mean between two given numbers a and b is (a + b)/2.
(v) 1 + 2 + 3 + ...... + n = [n(n + 1)]/2.
(vi) 1² + 2² + 3² +……………. + n² = [n(n+ 1)(2n+ 1)]/6.
(vii) 1³ + 2³ + 3³ + . . . . + n³ = [{n(n + 1)}/2 ]².
● Geometrical Progression (G.P.) :
(i) The general form of a G.P. is a, ar, ar², ar³, . . . . . where a is the first term and r, the common ratio of the G.P.
(ii) The n th term of the above G.P. is t₀ = a.rn−1 .
(iii) The sum of first n terms of the above G.P. is S = a ∙ [(1 - rⁿ)/(1 – r)] when -1 < r < 1
or, S = a ∙ [(rⁿ – 1)/(r – 1) ]when r > 1 or r < -1.
(iv) The geometric mean of two positive numbers a and b is √(ab) or, -√(ab).
(v) a + ar + ar² + ……………. ∞ = a/(1 – r) where (-1 < r < 1).
● Theory of Quadratic Equation :
ax² + bx + c = 0 ... (1)
(i) Roots of the equation (1) are x = {-b ± √(b² – 4ac)}/2a.
(ii) If α and β be the roots of the equation (1) then,
sum of its roots = α + β = - b/a = - (coefficient of x)/(coefficient of x² );
and product of its roots = αβ = c/a = (Constant term /(Coefficient of x²).
(iii) The quadratic equation whose roots are α and β is
x² - (α + β)x + αβ = 0
i.e. , x² - (sum of the roots) x + product of the roots = 0.
(iv) The expression (b² - 4ac) is called the discriminant of equation (1).
(v) If a, b, c are real and rational then the roots of equation (1) are
(a) real and distinct when b² - 4ac > 0;
(b) real and equal when b² - 4ac = 0;
(c) imaginary when b² - 4ac < 0;
(d) rational when b²- 4ac is a perfect square and
(e) irrational when b² - 4ac is not a perfect square.
(vi) If α + iβ be one root of equation (1) then its other root will be conjugate complex quantity α - iβ and conversely (a, b, c are real).
(vii) If α + √β be one root of equation (1) then its other root will be conjugate irrational quantity α - √β (a, b, c are rational).
● Permutation:
(i) ⌊n (or, n!) = n (n – 1) (n – 2) ∙∙∙∙∙∙∙∙∙ 3∙2∙1.
(ii) 0! = 1.
(iii) Number of permutations of n different things taken r ( ≤ n) at a time ⁿP₀ = n!/(n - 1)! = n (n – 1)(n - 2) ∙∙∙∙∙∙∙∙ (n - r + 1).
(iv) Number of permutations of n different things taken all at a time = ⁿP₀ = n!.
(v) Number of permutations of n things taken all at a time in which p things are alike of a first kind, q things are alike of a second kind, r things are alike of a third kind and the rest are all different, is ⁿ<span style='font-size: 50%'>!/₀
(vi) Number of permutations of n different things taken r at a time when each thing may be repeated upto r times in any permutation, is nʳ .
● Combination:
(i) Number of combinations of n different things taken r at a time = ⁿCr = n!r!(n−r)!
(ii) ⁿP₀ = r!∙ ⁿC₀.
(iii) ⁿC₀ = ⁿCn = 1.
(iv) ⁿCr = ⁿCn - r.
(v) ⁿCr + ⁿCn - 1 = n+1Cr
(vi) If p ≠ q and ⁿCp = ⁿCq then p + q = n.
(vii) ⁿCr/ⁿCr - 1 = (n - r + 1)/r.
(viii) The total number of combinations of n different things taken any number at a time = ⁿC₁ + ⁿC₂ + ⁿC₃ + …………. + ⁿC₀ = 2ⁿ – 1.
(ix) The total number of combinations of (p + q + r + . . . .) things of which p things are alike of a first kind, q things are alike of a second kind r things are alike of a third kind and so on, taken any number at a time is [(p + 1) (q + 1) (r + 1) . . . . ] - 1.
● Binomial Theorem:
(i) Statement of Binomial Theorem : If n is a positive integer then(a + x)n = an + nC1 an - 1 x + nC2 an - 2 x2 + …………….. + nCr an - r xr + ………….. + xn …….. (1)
(ii) If n is not a positive integer then
(1 + x)n = 1 + nx + [n(n - 1)/2!] x2 + [n(n - 1)(n - 2)/3!] x3 + ………… + [{n(n-1)(n-2)………..(n-r+1)}/r!] xr+ ……………. ∞ (-1 < x < 1) ………….(2)
(iii) The general term of the expansion (1) is (r+ 1)th term
= tr + 1 = nCr an - r xr
(iv) The general term of the expansion (2) is (r + 1) th term
= tr + 1 = [{n(n - 1)(n - 2)....(n - r + l)}/r!] ∙ xr.
(v) There is one middle term is the expansion ( 1 ) when n is even and it is (n/2 + 1)th term ; the expansion ( I ) will have two middle terms when n is odd and they are the {(n - 1)/2 + 1} th and {(n - 1)/2 + 1} th terms.
(vi) (1 - x)-1 = 1 + x + x2 + x3 + ………………….∞.
(vii) (1 + x)-1 = I - x + x2 - x3 + ……………∞.
(viii) (1 - x)-2 = 1 + 2x + 3x2 + 4x3 + . . . . ∞ .
(ix) (1 + x)-2 = 1 - 2x + 3x2 - 4x3 + . . . . ∞ .
● Logarithm:
(i) If ax = M then loga M = x and conversely.(ii) loga 1 = 0.
(iii) loga a = 1.
(iv) a logam = M.
(v) loga MN = loga M + loga N.
(vi) loga (M/N) = loga M - loga N.
(vii) loga Mn = n loga M.
(viii) loga M = logb M x loga b.
(ix) logb a x 1oga b = 1.
(x) logb a = 1/logb a.
(xi) logb M = logb M/loga b.
● Exponential Series:
(i) For all x, ex = 1 + x/1! + x2/2! + x3/3! + …………… + xr/r! + ………….. ∞.(ii) e = 1 + 1/1! + 1/2! + 1/3! + ………………….. ∞.
(iii) 2 < e < 3; e = 2.718282 (correct to six decimal places).
(iv) ax = 1 + (loge a) x + [(loge a)2/2!] ∙ x2 + [(loge a)3/3!] ∙ x3 + …………….. ∞.
● Logarithmic Series:
(i) loge (1 + x) = x - x2/2 + x3/3 - ……………… ∞ (-1 < x ≤ 1).(ii) loge (1 - x) = - x - x2/ 2 - x3/3 - ………….. ∞ (- 1 ≤ x < 1).
(iii) ½ loge [(1 + x)/(1 - x)] = x + x3/3 + x5/5 + ……………… ∞ (-1 < x < 1).
(iv) loge 2 = 1 - 1/2 + 1/3 - 1/4 + ………………… ∞.
(v) log10 m = µ loge m where µ = 1/loge 10 = 0.4342945 and m is a positive number.
● Formula
- Basic Math Formulas
- Math Formula Sheet on Co-Ordinate Geometry
- All Math Formula on Mensuration
- Simple Math Formula on Trigonometry
11 and 12 Grade Math
From Basic Math Formulas to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade Circle Worksheet | Free Worksheet with Answer |Practice Math
Jul 11, 25 02:14 PM
In 5th Grade Circle Worksheet you will get different types of questions on parts of a circle, relation between radius and diameter, interior of a circle, exterior of a circle and construction of circl… -
Construction of a Circle | Working Rules | Step-by-step Explanation |
Jul 09, 25 01:29 AM
Construction of a Circle when the length of its Radius is given. Working Rules | Step I: Open the compass such that its pointer be put on initial point (i.e. O) of ruler / scale and the pencil-end be… -
Combination of Addition and Subtraction | Mixed Addition & Subtraction
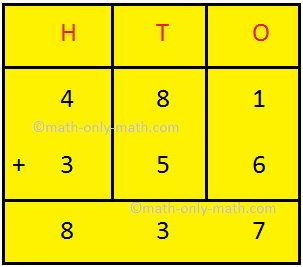
Jul 08, 25 02:32 PM
We will discuss here about the combination of addition and subtraction. The rules which can be used to solve the sums involving addition (+) and subtraction (-) together are: I: First add -
Addition & Subtraction Together |Combination of addition & subtraction
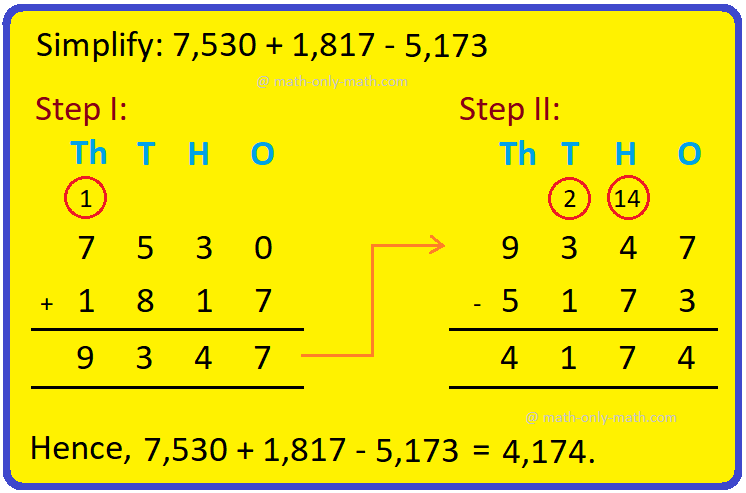
Jul 08, 25 02:23 PM
We will solve the different types of problems involving addition and subtraction together. To show the problem involving both addition and subtraction, we first group all the numbers with ‘+’ and… -
5th Grade Circle | Radius, Interior and Exterior of a Circle|Worksheet
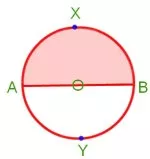
Jul 08, 25 09:55 AM
A circle is the set of all those point in a plane whose distance from a fixed point remains constant. The fixed point is called the centre of the circle and the constant distance is known



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.