Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Properties of Multiplication
In this section, we shall learn some interesting properties of multiplication.
1. Order Property of Multiplication:
Look at the figures given below:
|
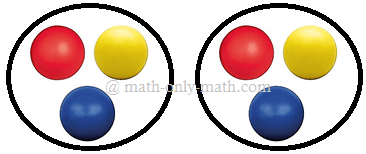
3 + 3 = 6 Thus, 3 two times is 6. So, 2 × 3 = 6 |
2 + 2 + 2 = 6 Thus, 2 three times is 6. So, 3 × 2 = 6 |
What do we conclude?
We conclude that when two numbers are multiplied they may be taken in any order. The result remains the same. This is called the order property.
More examples: 4 × 3 = 3 × 4, 5 × 6 = 6 × 5 etc.
2. Multiplicative Property of 1:
Look at the figures given below:
|
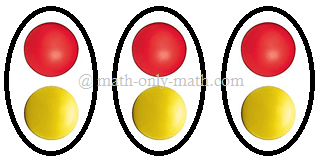
1 + 1 + 1 = 3 Thus, 1 three times is 3 So, 1 * 3 = 3 Hence, 1 * 3 = 3 * 1 What do we conclude? |
Thus, 3 one is 3 So, 3 * 1 = 3 |
What do we conclude?
When a number is multiplied by 1, the answer is the number itself. This is called multiplicative property of 1.
More examples: 1 × 4 = 4, 1 × 8 = 8, 1 × 10 = 10 etc
3. Multiplicative Property of 0:
We know that: 0 + 0 + 0 + 0 = 0
Thus, 0 four times = 0
So, 0 * 4 = 0
By order property: 0 × 4 = 4 × 0
Hence, 0 × 4 = 4 × 0 = 0
Similarly, 0 + 0 + 0 + 0 + 0 = 0
Thus, 0 six times = 0
So, 0 × 6 = 0
But, 0 × 6 = 6 × 0
Hence, 0 × 6 = 6 × 0 = 0
What do we conclude?
We conclude that when any number is multiplied by zero, the product is always zero.
Basic Properties of Multiplication Video
Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Worksheet on Properties of Multiplication:
1. Fill in the empty boxes by using the properties of multiplication:
(i) 4 × 1 = _____
(ii) 9 × 0 = _____
(iii) 2 × 7 = _____ × 2
(iv) 5 × 0 = _____
(v) 10 × 1 = _____
(vi) 0 × 8 = _____
(vii) 3 × 5 = _____ × 3
(viii) 8 × 1 = _____
(ix) 0 × 7 = 7 × _____
(x) _____ × 13 = 13 × 6
(xi) _____ × 9 = 0
(xii) 6 × _____ = 3 × 6
Answer:
1. (i) 1
(ii) 0
(iii) 7
(iv) 0
(v) 10
(vi) 0
(vii) 5
(viii) 8
(ix)0
(x) 6
(xi) 0
(xii) 3
You might like these
2nd grade math Worksheets | Free Math Worksheets | By Grade and Topic
2nd grade math worksheets is carefully planned and thoughtfully presented on mathematics for the students.
Cardinal Numbers and Ordinal Numbers | Cardinal Numbers | Ordinal Num
Cardinal numbers and ordinal numbers are explained here with the help of colorful pictures. There are many steps in a staircase as shown in the above figure. The given staircase has nine steps,
Worksheet on Cardinal Numbers and Ordinal Numbers | Cardinal & Ordinal
Worksheet on cardinal numbers and ordinal numbers, activities are given below so that kids can practice. This sheet will help the children to write the numbers in both cardinal and ordinal.
Worksheet on Two Digit Numbers | Numbers in Words | Two-Digit Numbers
In worksheet on 2-digit numbers we will write the number which come before, after and in between, write the numerals, write the number names, place value and face value of 2-digit numbers, numbers in expanded form, write the numbers in short form, write the tens and the ones
Comparison of Two-digit Numbers | Arrange 2-digit Numbers | Examples
What are the rules for the comparison of two-digit numbers? We know that a two-digit number is always greater than a single digit number. But, when both the numbers are two-digit numbers
Adding 1-Digit Number | Understand the Concept one Digit Number |Video
Understand the concept of adding 1-digit number with the help of objects as well as numbers.
Adding 2-Digit Numbers | Add Two Two-Digit Numbers without Carrying
Here we will learn adding 2-digit numbers without regrouping and start working with easy numbers to get acquainted with the addition of two numbers.
Subtracting 1-Digit Number | Subtract Two One-Digit Number | Video
In subtracting 1-digit number we will subtract or minus one-digit number from one-digit number or one-digit number from 2-digit number and find the difference between them. We know that subtraction means to take away a set of objects from a collection.
Addition Word Problems – 2-Digit Numbers | Addition Word Problems
Basic concept on addition word problems – 2-digit numbers without regrouping for 2nd graders. Students need to read the questions carefully and find the sums of these 2-digit by 2-digit
Subtraction Word Problems - 2-Digit Numbers | Subtraction Problems
Understand the concept on subtraction word problems - 2-digit numbers for the second grade. Read the question carefully to subtract the two-digit numbers to find the differences and follow the
From 2-Digit Numbers Subtraction using Expanded Form to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.