Subscribe to our ▶️YouTube channel🔴 for the latest videos, updates, and tips.
Home | About Us | Contact Us | Privacy | Math Blog
Reduce Algebraic Fractions to its Lowest Term
If the numerator and denominator of an algebraic fraction have no common factor other than 1, it is said to be in the lowest form.
The reduced form of an algebraic fraction means there is no common factor between the numerator and denominator of the given algebraic fractions. That means, if there is any common factor present in the numerator and denominator then by keeping the value of the algebraic fraction unchanged, the common factor is freed by mathematical method and the algebraic fraction will be reduced to its lowest form.
When we reduce an algebraic fraction to its lowest term we need to remember if the ‘numerator’ and ‘denominator’ of the fractions are ‘multiplied’ or ‘divided’ by the same quantity, then the value of the fraction remains unchanged.
To reduce algebraic fractions to its lowest term, we need to follow the following steps:
Step I: take the factorization of polynomial in the numerator and denominator.
Step II: then cancel out the common factors in the numerator and denominator.
Step III: reduce the given algebraic fraction to the lowest term.
Note: The H.C.F. of numerator
and denominator is 1.
For example:
1. In the numerator ma and the denominator mb of mamb, is the common factor, so the algebraic fraction mamb is not in its lowest terms. Now, divide both the numerator and denominator by the common factor ‘m’ then we get ma÷mmb÷m = ab there is no common factor, so ab is the algebraic fraction which is in reduced form.
2. x3+9x2+20xx2+2x−15
We see that the numerator and denominator of the given algebraic fraction is polynomial, which can be factorized.
= x(x2+9x+20)x2+5x−3x−15
= x(x2+5x+4x+20)x2+5x−3x−15
= \frac{x[x(x + 5) + 4(x + 5)]}{x(x + 5) – 3(x + 5)}
= \frac{x(x + 5)(x + 4)}{(x + 5) (x – 3)}
We observed that in the numerator and denominator of the algebraic fraction, (x + 5) is the common factor and there is no other common factor. Now, when the numerator and denominator of the algebraic fraction is divided by this common factor or their H.C.F. the algebraic fraction becomes,
= \frac{\frac{x{(x + 5) (x + 4)}}{(x + 5)}}{\frac{(x + 5) (x - 3)}{(x + 5)}}
= \frac{x(x + 4)}{(x – 3)}, which is the lowest form of the given algebraic fraction.
8th Grade Math Practice
From Reduce Algebraic Fractions to its Lowest Term to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
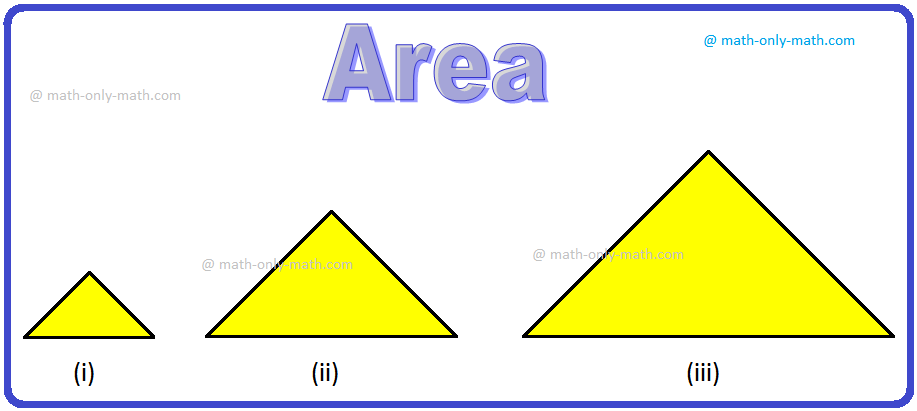
Area of Rectangle Square and Triangle | Formulas| Area of Plane Shapes
Jul 18, 25 10:38 AM
Area of a closed plane figure is the amount of surface enclosed within its boundary. Look at the given figures. The shaded region of each figure denotes its area. The standard unit, generally used for… -
What is Area in Maths? | Units to find Area | Conversion Table of Area
Jul 17, 25 01:06 AM
The amount of surface that a plane figure covers is called its area. It’s unit is square centimeters or square meters etc. A rectangle, a square, a triangle and a circle are all examples of closed pla… -
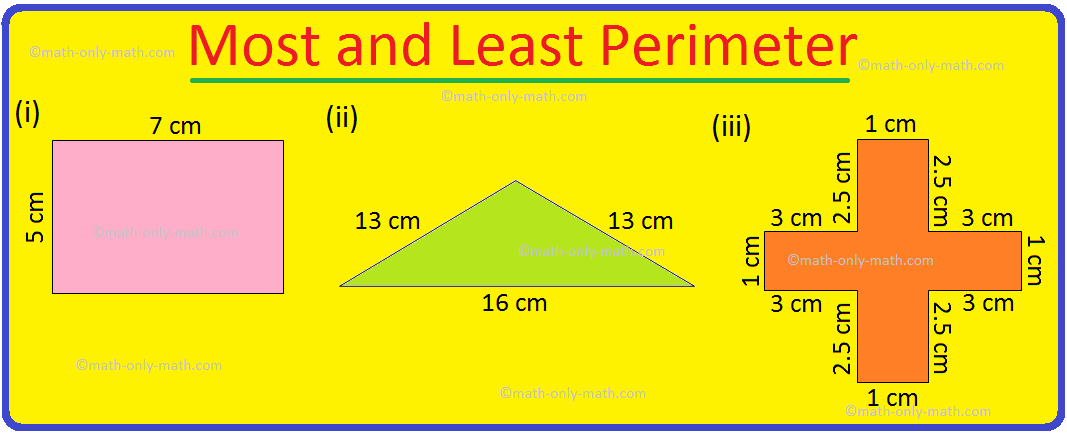
Worksheet on Perimeter | Perimeter of Squares and Rectangle | Answers
Jul 17, 25 12:40 AM
Practice the questions given in the worksheet on perimeter. The questions are based on finding the perimeter of the triangle, perimeter of the square, perimeter of rectangle and word problems. I. Find… -
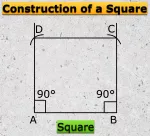
Formation of Square and Rectangle | Construction of Square & Rectangle
Jul 16, 25 11:46 PM
In formation of square and rectangle we will learn how to construct square and rectangle. Construction of a Square: We follow the method given below. Step I: We draw a line segment AB of the required… -
Perimeter of a Figure | Perimeter of a Simple Closed Figure | Examples
Jul 16, 25 02:33 AM
Perimeter of a figure is explained here. Perimeter is the total length of the boundary of a closed figure. The perimeter of a simple closed figure is the sum of the measures of line-segments which hav…


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.