Subscribe to our YouTube channel for the latest videos, updates, and tips.
Application of Basic Proportionality Theorem
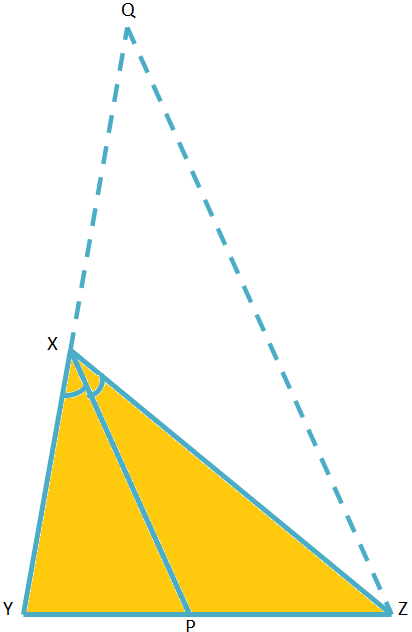
Here we will prove that the internal bisector of an angle of a triangle divides the opposite side in the ratio of the sides containing the angle.
Given: XP is the internal bisector of ∠YXZ, intersecting YZ at P.
To prove: YPPZ = XYXZ.
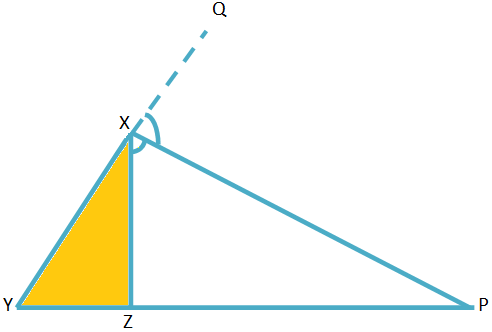
Construction: Draw ZQ ∥ XP such that ZQ meets YX produced at Q.
Proof:
|
Statement 1. ∠YXP = ∠XQZ 2. ∠PXZ = ∠XZQ 3. ∠XQZ = ∠XZQ 4. XQ = XZ 5. YXXQ = YPPZ 6. YXXZ = YPPZ |
Reason 1. XP ∥ QZ and YQ is a transversal 2. XP ∥ QZ and XZ is a transversal 3. ∠YXP = ∠PXZ 4. ∠XQZ = ∠XZQ 5. XP ∥ QZ 6. By statement 4. |
Note:
1. The above proposition is true for external division also.
So, YPZP = XYXZ
2. Converse of the above proposition is also true.
So, if P is a point on YZ such that YP : PZ = XY : XZ then XP bisects the angle YXZ internally or externally.
From Application of Basic Proportionality Theorem to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade Circle Worksheet | Free Worksheet with Answer |Practice Math
Jul 10, 25 11:41 AM
In 5th Grade Circle Worksheet you will get different types of questions on parts of a circle, relation between radius and diameter, interior of a circle, exterior of a circle and construction of circl… -
Construction of a Circle | Working Rules | Step-by-step Explanation |
Jul 09, 25 01:29 AM
Construction of a Circle when the length of its Radius is given. Working Rules | Step I: Open the compass such that its pointer be put on initial point (i.e. O) of ruler / scale and the pencil-end be… -
Combination of Addition and Subtraction | Mixed Addition & Subtraction
Jul 08, 25 02:32 PM
We will discuss here about the combination of addition and subtraction. The rules which can be used to solve the sums involving addition (+) and subtraction (-) together are: I: First add -
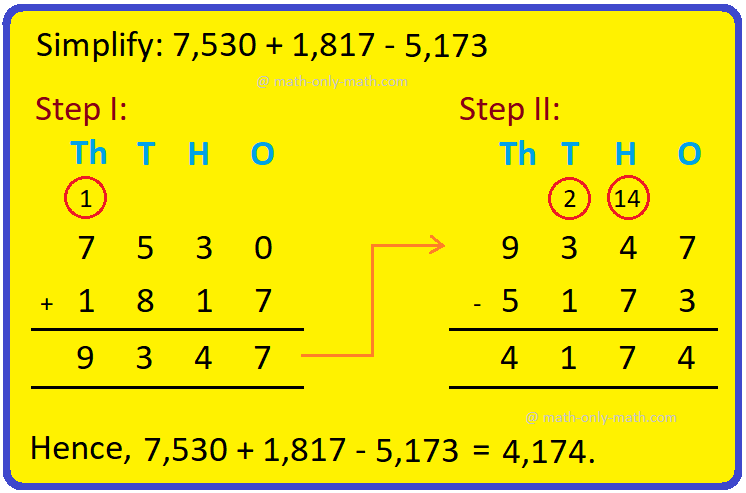
Addition & Subtraction Together |Combination of addition & subtraction
Jul 08, 25 02:23 PM
We will solve the different types of problems involving addition and subtraction together. To show the problem involving both addition and subtraction, we first group all the numbers with ‘+’ and… -
5th Grade Circle | Radius, Interior and Exterior of a Circle|Worksheet
Jul 08, 25 09:55 AM
A circle is the set of all those point in a plane whose distance from a fixed point remains constant. The fixed point is called the centre of the circle and the constant distance is known



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.