Subscribe to our YouTube channel for the latest videos, updates, and tips.
Lateral Surface Area of a Cuboid
Here we will learn how to solve the application problems on lateral surface area of a cuboid using the formula.
Formula for finding the lateral surface area of a cuboid
Area of a Rooms is example of cuboids.
Are of the four walls of a room = sum of the four vertical (or lateral) faces
= 2(l + b)h
Where l = Length, b = breadth and h = height.
Therefore, lateral surface area of a cuboid = 2(l + b)h
Problems on Lateral Surface Area of a Cuboid:
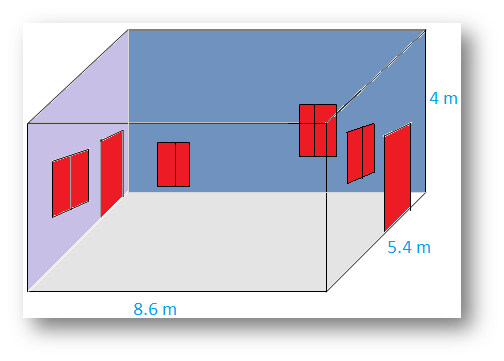
The dimensions of a cubical room are 8.6 m × 5.4 m × 4 m. The room has two doors each measuring 2 m by 1 m and four windows each measuring 1 m by 1 m. What will be the cost of plastering the four walls and the ceiling at RS 65 per m2?
Solution:
The total area of the four walls
= Lateral surface area of the cuboid
= 2(l + b)h
= 2(86 + 5.4) × 4 m2
= 112 m2.
The area of the ceiling = l × b
= 8.6 × 5.4 m2
= 46.44 m2
The sum of the areas of the two doors and the four windows
= 2(2 × 1) m2 + 4(1 × 1) m2
= 8 m2
Therefore, the total area to be plastered = (112 + 46.44 - 8) m2
= 150.44 m2
Therefore, the cost of plastering = 150.44 × Rs. 65
= Rs. 9778.60.
From Lateral Surface Area of a Cuboid to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Worksheet on Simple Interest | Word problem on Simple Interest | Free
Jun 19, 25 02:54 AM
In worksheet on simple interest we will get different types of question on calculating the simple interest, the principal amount, the rate of interest and the word problems on simple interest. -
Terms Related to Simple Interest | Simple Interest Formula | Principal
Jun 19, 25 12:20 AM
In terms related to simple interest we will learn all the terms related to simple interest. The terms related to simple interest are Interest, Principal, Amount, Simple Interest, Time or period of tim… -
Introduction to Simple Interest | Definition | Formula | Examples
Jun 18, 25 01:50 AM
In simple interest we will learn and identify about the terms like Principal, Time, Rate, Amount, etc. PRINCIPAL (P): The money you deposit or put in the bank is called the PRINCIPAL. -
5th Grade Profit and Loss Percentage Worksheet | Profit and Loss | Ans
Jun 18, 25 01:33 AM
In 5th grade profit and loss percentage worksheet you will get different types of problems on finding the profit or loss percentage when cost price and selling price are given, finding the selling pri… -
Worksheet on Profit and Loss | Word Problem on Profit and Loss | Math
Jun 18, 25 01:29 AM
In worksheet on profit and loss, we can see below there are some different types of questions which we can practice in our homework.
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.