Cylinder

A solid with uniform cross section perpendicular to its length (or height) is a cylinder. The cross section may be a circle, a triangle, a square, a rectangle or a polygon. A can, a pencil, a book, a glass prism, etc., are examples of cylinders. Each one of the figures shown below is a cylinder.

$Cylinder Figure$ First Figure

$Cuboid Cylinder$ Second Figure

$Image Cylinder$ Third Figure

$Figure Cylinder$ Fourth Figure

$Prism Cylinder$ Fifth Figure

The second figure shown above is a cuboid, which is also a cylinder. The cylinder depicted in the last figure is also called prism.

The formule for the volume and the surface area of a cylinder are the same as those of solids with uniform cross section.

Volume of a Cylinder = (Area of the cross section) × length (or height or breadth)

= A × h

Lateral surface area of a Cylinder = (Perimeter of the cross section) × length (or height or breadth)

= P × h

Total surface area of a Cylinder = Lateral surface area + Sum of the areas of the two plane ends

= P × h + 2 × A

Solved Problems on Volume and Surface Area of Cylinder:

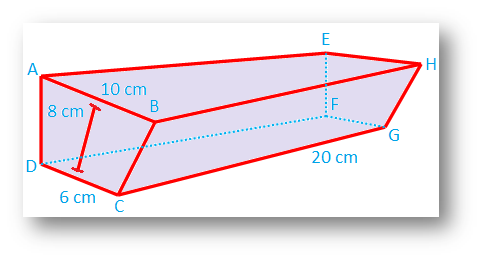
1. The cross section of a cylinder is a trapezium whose parallel sides measure 10 cm and 6 cm, and the distance between the parallel sides is 8 cm. If the cylinder is 20 cm long, find (i) the area of the cross section; and (ii) the volume of the cylinder.

Solution:

$Volume and Surface Area of Cylinder$

(i) The area of the cross section = area of the trapezium

= $\frac{1}{2}$ (10 + 6)8 cm²

= 64 cm²

(ii) The volume of the cylinder = (Area of the cross Section) × length

= 64 cm² × 20 cm

= 1280 cm³

2. The cross section of a cylinder is a regular hexagon of side 4 cm and its height measures 12 cm. Find its (i) lateral surface area, and (ii) total surface area.

Solution:

$Regular Hexagon Cylinder$

(i) The perimeter of the cross section

= Perimeter of a regulator hexagon of side 4 cm

= 6 × 4 cm

= 24 cm.

Therefore, lateral surface area of the cylinder

= (Perimeter of the cross section) × height

= 24 cm × 12 cm

= 288 cm²

(ii) Total surface area = lateral surface area + 2 × (Area of the Cross Section)

= 288 cm² + 2 × (Area of the regular hexagon of side 4 cm)

= 288 cm² + 2 × $\frac{3√3}{2}$ × 4^2 cm²

= (288 + 48√3) cm²

= (288 + 83.04) cm²

= 371.04 cm²

You might like these

Problems on Right Circular Cylinder | Application Problem | Diagram

Problems on right circular cylinder. Here we will learn how to solve different types of problems on right circular cylinder. 1. A solid, metallic, right circular cylindrical block of radius 7 cm and height 8 cm is melted and small cubes of edge 2 cm are made from it.
Hollow Cylinder | Volume |Inner and Outer Curved Surface Area |Diagram

We will discuss here about the volume and surface area of Hollow Cylinder. The figure below shows a hollow cylinder. A cross section of it perpendicular to the length (or height) is the portion bounded by two concentric circles. Here, AB is the outer diameter and CD is the
Right Circular Cylinder | Lateral Surface Area | Curved Surface Area

A cylinder, whose uniform cross section perpendicular to its height (or length) is a circle, is called a right circular cylinder. A right circular cylinder has two plane faces which are circular and curved surface. A right circular cylinder is a solid generated by the
Cross Section | Area and Perimeter of the Uniform Cross Section

The cross section of a solid is a plane section resulting from a cut (real or imaginary) perpendicular to the length (or breadth of height) of the solid. If the shape and size of the cross section is the same at every point along the length (or breadth or height) of the
Lateral Surface Area of a Cuboid | Are of the Four Walls of a Room

Here we will learn how to solve the application problems on lateral surface area of a cuboid using the formula. Formula for finding the lateral surface area of a cuboid Area of a Rooms is example of cuboids. Are of the four walls of a room = sum of the four vertical