Learn math step-by-step.
Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
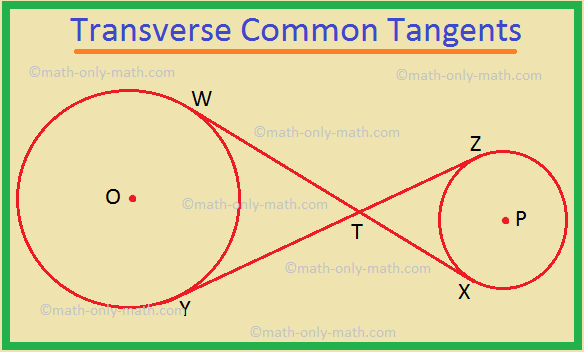
Transverse Common Tangents
A common tangent is called a transverse common tangent if the circles lie on opposite sides of it.
In the figure, WX is a transverse common tangent as the circle with centre O lies below it and the circle with P lie above it.
YZ is the other transverse common tangent as the circle with centre O lies above it and the circle with centre P lies below it. A transverse common tangent is possible only when the circles are apart.
From Transverse Common Tangents to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.

New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.