Slope of the Graph of y = mx + c

Here we will learn how to solve the slope of the graph of y = mx + c.

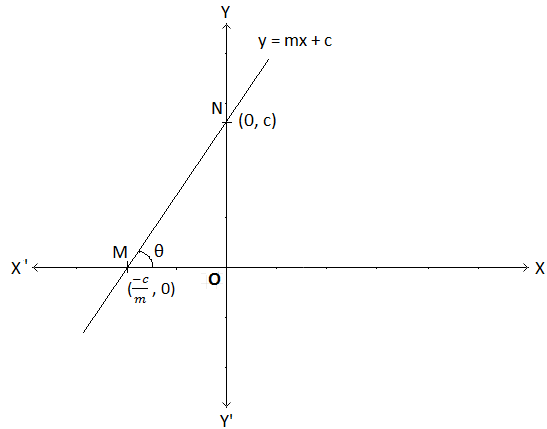
The graph of y = mx + c is a straight line joining the points (0, c) and (−cm, 0).

Let M = (−cm, 0) and N = (0, c) and ∠NMX = θ.

Then, tan θ is called the slope of the line which is the graph of y = mx + c.

Now, ON = c and OM = cm.

Therefore, in the right-angled ∆MON, tan θ = ONOM = ccm = m.

Thus, the slope of the line which is the graph of y = mx + c is m 

And m is equal to the tangent of the angle that the line makes with the positive direction of the x-axis.

Slope of the Graph of y = mx + c

Solved examples on slope of the graph of y = mx + c:

1. What is the slope of the line which makes 60° with the positive direction of the x-axis?

Solution:

The slope = tan 60° = √3


2. What is the slope of the line which is the graph of 2x – 3y + 5 = 0?

Solution:

Here, 2x – 3y + 5 = 0

⟹ 3y = 2x + 5

⟹ y = 23x + 53.

Comparing with y = mx + c, we have m = 23.

Therefore, the slope of the line is 23.






9th Grade Math

From Slope of the graph of y = mx + c to HOME PAGE




Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.



New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.




Share this page: What’s this?

Recent Articles

RSS

  1. Area of Rectangle Square and Triangle | Formulas| Area of Plane Shapes

    Jul 18, 25 10:38 AM

    Area of a Square of Side 1 cm
    Area of a closed plane figure is the amount of surface enclosed within its boundary. Look at the given figures. The shaded region of each figure denotes its area. The standard unit, generally used for…

    Read More

  2. What is Area in Maths? | Units to find Area | Conversion Table of Area

    Jul 17, 25 01:06 AM

    Concept of Area
    The amount of surface that a plane figure covers is called its area. It’s unit is square centimeters or square meters etc. A rectangle, a square, a triangle and a circle are all examples of closed pla…

    Read More

  3. Worksheet on Perimeter | Perimeter of Squares and Rectangle | Answers

    Jul 17, 25 12:40 AM

    Most and Least Perimeter
    Practice the questions given in the worksheet on perimeter. The questions are based on finding the perimeter of the triangle, perimeter of the square, perimeter of rectangle and word problems. I. Find…

    Read More

  4. Formation of Square and Rectangle | Construction of Square & Rectangle

    Jul 16, 25 11:46 PM

    Construction of a Square
    In formation of square and rectangle we will learn how to construct square and rectangle. Construction of a Square: We follow the method given below. Step I: We draw a line segment AB of the required…

    Read More

  5. Perimeter of a Figure | Perimeter of a Simple Closed Figure | Examples

    Jul 16, 25 02:33 AM

    Perimeter of a Figure
    Perimeter of a figure is explained here. Perimeter is the total length of the boundary of a closed figure. The perimeter of a simple closed figure is the sum of the measures of line-segments which hav…

    Read More