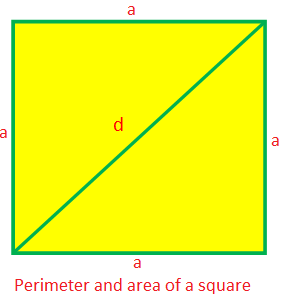
Perimeter and Area of a Square

Here we will discuss about the perimeter and area of a square and some of its geometrical properties.

Perimeter of a square (P) = 4 × side = 4a

Area of a square (A) = (side)² = a²

Diagonal of a square (d) = $\sqrt{(\textrm{side})^{2}+(\textrm{side})^{2}}$

                                    = $\sqrt{\textrm{a}^{2}+\textrm{a}^{2}}$

                                    = √2a

Side of a square (a) = √A = $\frac{P}{4}$

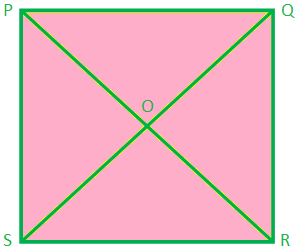
Some geometrical properties of a square

In the square PQRS,

PQ = QR = RS = SP

PR = QS

∠PQR = ∠QRS = ∠RSP = ∠SPQ = 90°.

PR and QS are perpendicular bisectors of each other.

Area of the ∆POQ = Area of the ∆QOR = Area of the ∆ROS = Area of the ∆SOP

Solved Examples on Perimeter and Area of a Square:

1. The perimeter and the area of a square are x cm and x cm$^{2}$ respectively. 

(i) Find the perimeter.

(ii) Find the area.

(iii) Find the length of a diagonal of the square.

Solution:

Let a cm be the measure of a side of the square.

Then the perimeter = 4 a cm, area = a$^{2}$ cm$^{2}$

From the question,

4a = x = a$^{2}$

or, a$^{2}$ - 4a = 0

or, a(a - 4) = 0  

Therefore, a = 0

or, a = 4

But, the side of a square ≠ 0

Hence, the side of the square = 4 cm

(i) Perimeter of a square = 4a

                                     = 4 × 4 cm

                                     = 16 cm

(ii) Area of a square = a$^{2}$ cm$^{2}$

                              = 4$^{2}$ cm$^{2}$

                              = 16 cm$^{2}$

(iii) Length of a diagonal = √2a

                                    = √2 ∙ 4 cm

                                    = 4√2 cm

                                    = 4 × 1.41 cm

                                    = 5.64 cm

9th Grade Math

From Perimeter and Area of a Square to HOME PAGE