Measure of the Angles of the Cyclic Quadrilateral

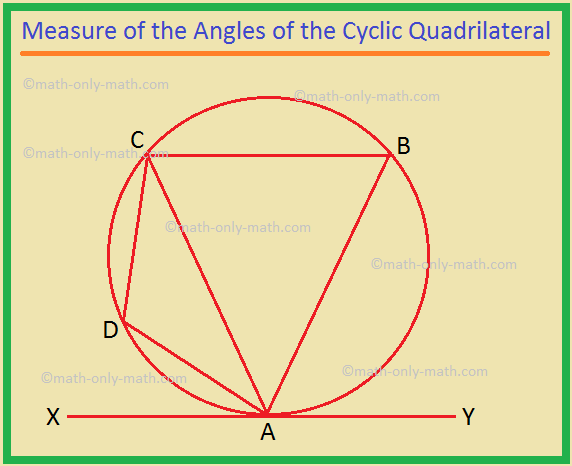
We will prove that, in the figure ABCD is a cyclic quadrilateral and the tangent to the circle at A is the line XY. If ∠CAY : ∠CAX = 2 : 1 and AD bisects the angle CAX while AB bisects ∠CAY then find the measure of the angles of the cyclic quadrilateral. Also, prove that DB is a diameter of the circle.

Measure of the Angles of the Cyclic Quadrilateral

Solution:

∠CAY + ∠CAX = 180° and ∠CAY : ∠CAX = 2 : 1.

Therefore, ∠CAY = \(\frac{2}{3}\) × 180° = 120° and ∠CAX = \(\frac{1}{3}\) × 180° = 60°.

As AD bisects ∠CAX, ∠DAX = ∠CAD = \(\frac{1}{2}\) × 60° = 30°

As AB bisects ∠CAY, ∠YAB = ∠CAB = \(\frac{1}{2}\) × 120° = 60°.

Now, ∠CAY = ∠ADC = 120° (Since, angle between tangent and chord is equal to the angle in the alternate segment).

Therefore, ∠CBA = 180° - ∠ADC = 180° - 120° = 60° (Since opposite angles of a cyclic quadrilateral are supplementary).

Again, ∠DAB = ∠DAC + ∠CAB = 30° + 60° = 90°.

Therefore, ∠BCD = 180° - ∠DAB = 180° - 90° = 90°.

We can see that the chord DB subtends a right angle at A.

Therefore, DB is a diameter of the circle (as an angle in a semicircle is a right-angle).






10th Grade Math

From Measure of the Angles of the Cyclic Quadrilateral to HOME PAGE




Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.



New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.




Share this page: What’s this?

Recent Articles

RSS

  1. Quarter Past and Quarter To | Quarter Past Hour | Quarter to Next Hour

    Nov 23, 24 03:45 PM

    Quarter Past and Quarter To
    The hands of clock move from left to right. This is called the clock wise motion. When the minute hand is on the right side of the clock, it shows the number of minutes past the hour. When the minute…

    Read More

  2. Half Past an Hour | What does Half Past Mean? | Half an Hour|Half Past

    Nov 23, 24 03:14 PM

    Half Past 1
    We learnt that, one hour is equal to 60 minutes. When one hour is divided into two, it is half an hour or 30 minutes. The minute hand points at 6. We say, 30 minutes past an hour or half past an hour…

    Read More

  3. Telling the Time | Teaching Time | Analogue Clock| Reading Time

    Nov 23, 24 02:51 PM

    Wall Clock
    Teaching time is an interactive activity for telling time. This activity helps students to learn how to read the clock to tell time using the analogue clock. While reading or observing the time on a

    Read More

  4. 2nd Grade Fractions Worksheet | Basic Concept of Fractions | Answers

    Nov 23, 24 12:22 AM

    Divide the Collection into 4 Equal Parts
    In 2nd Grade Fractions Worksheet we will solve different types of problems on fractions, one-whole, one-half, one-third, one-fourth, three-fourth or s quarter. In a fraction, it is important that the…

    Read More

  5. Time Duration |How to Calculate the Time Duration (in Hours & Minutes)

    Nov 22, 24 12:34 AM

    Time Duration Example
    Time duration tells us how long it takes for an activity to complete. We will learn how to calculate the time duration in minutes and in hours. Time Duration (in minutes) Ron and Clara play badminton…

    Read More