Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
Laws of Indices
We will discuss here about the different Laws of Indices.
If a, b are real numbers (>0, ≠ 1) and m, n are real numbers, following properties hold true.
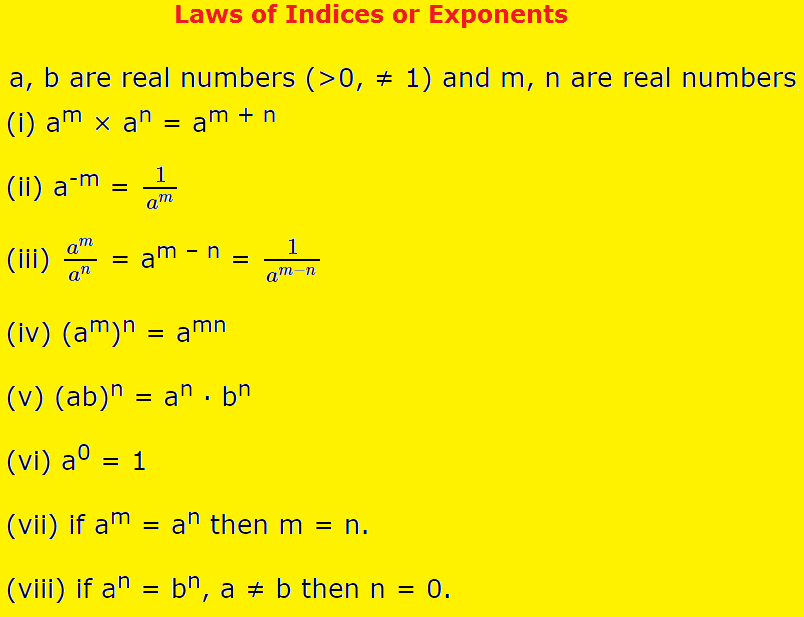
(i) am × an = am + n
(ii) a-m = 1am
(iii) aman = am – n = 1am−n
(iv) (am)n = amn
(v) (ab)n = an ∙ bn
(vi) a0 = 1
(vii) if am = an then m = n.
(viii) if an = bn, a ≠ b then n = 0.
Note: Some of the above properties hold true for any two real numbers a, b. Laws (i) to (v) hold true for any two real numbers a, b. Also note that 10 = 1.
Problems on knowledge and use of the properties of indices:
1. Determine the numerical value for each of the following (not containing exponents):
(i) 64
(ii) (-5)-4
(iii) 90
(iv) (14)-5
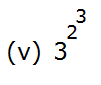
(vi) (13)0
Solution:
(i) 64 = 6 × 6 × 6 × 6 = 1296; [Using the definition of power/exponent].
(ii) (-5)-4 = 1(−5)4; [Using the property of indices].
= 1(−5)×(−5)×(−5)×(−5) ; [Using the definition of power].
= 125×25
= 1625
(iii) 90 = 1; [Using the property of indices: here 9 ≠ 0].
(iv) (14)-5 = (4-1)-5 = 4(-1) × (-5) = 45 = 1024
(vi) (13)0 = 1; [Using the property of indices: here 13 ≠ 0].
From Laws of Indices to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
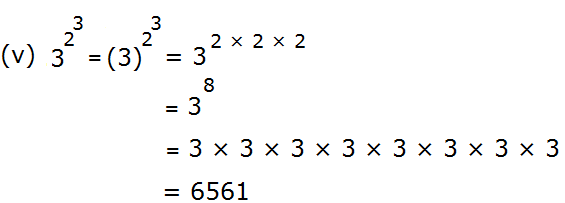


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.