Important Properties of Transverse Common Tangents

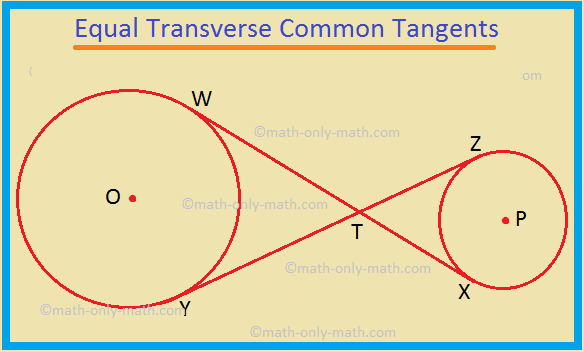
I. The two transverse common tangents drawn to two circles are equal in length.

Given:

WX and YZ are two transverse common tangents drawn to the two given circles with centres O and P. WX and YZ intersect at T.

Equal Transverse Common Tangents

To prove: WX = YZ.

Proof:

Statement

Reason

1. WT = YT.

1. The two tangents, drawn to a circle from an external point, are equal in length.

2. XT = ZT.

2. An in statement 1.

3. WT + XT = YT + ZT

⟹ WX = YZ. (Proved)

3. Adding statements 1 and 2.

Length of a Transverse Common Tangent


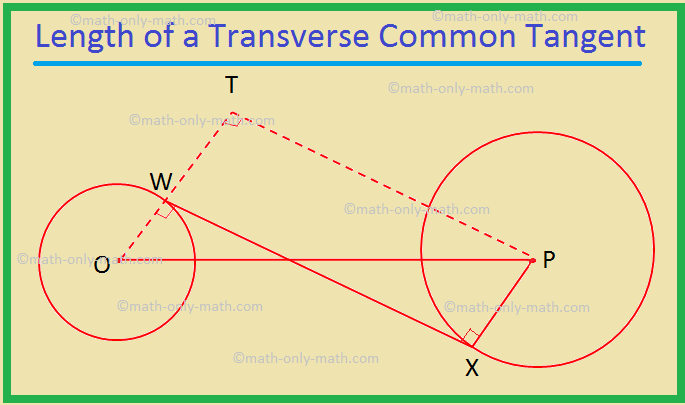
II. The length of a transverse common tangent to two circles is \(\sqrt{d^{2} – (r_{1} + r_{2})^{2}}\), where d is the distance between the centres of the circles, and r\(_{1}\) and r\(_{2}\) are the radii of the given circles.

Proof:

Let two circles be given with centres O and P, and radii r\(_{1}\) and r\(_{2}\) respectively, where r\(_{1}\) < r\(_{2}\). Let the distance between the centres of the circles, OP = d.

Let WX be a transverse common tangent.

Therefore, OW = r\(_{1}\) and PX = r\(_{2}\).

Also, OW ⊥ WX and PX ⊥ WX, because a tangent is perpendicular to the radius drawn through the point of contact

Produce W to T such that WT = PX = r\(_{2}\). Join T to P. In the quadrilateral WXPT, WT ∥ PX, as both are perpendiculars to WX; and WT = PX. Therefore, WXPT is a rectangle. Thus, WX = PT, as the opposite sides of a rectangle are equal.

OT = OW + WT = r\(_{1}\)  +  r\(_{2}\).

In the right-angled triangle OPT, we have

PT2 = OP2 – OT2 (by Pythagoras’ Theorem)

⟹ PT2 = d2 – (r\(_{1}\) + r\(_{1}\))\(^{2}\)

⟹ PT = \(\sqrt{d^{2} – (r_{1} + r_{2})^{2}}\)

⟹ WX = \(\sqrt{d^{2} – (r_{1} + r_{2})^{2}}\) (Since, PT = WX).


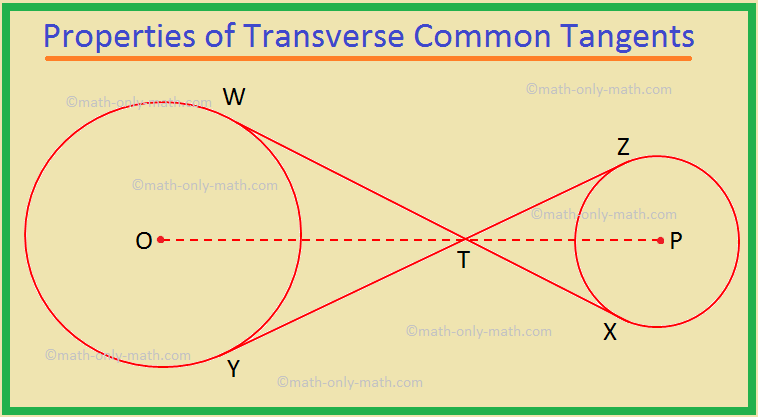
III. The transverse common tangents drawn to two circles intersect on the line drawn through the centres of the circles.

Given: Two circles with centres O and P, and their transverse common tangents WX and YZ, which intersects at T

Properties of Transverse Common Tangents

To prove: T lies on the line joining O to P, i.e., O T and P lie on the same straight line.

Proof:

Statement

Reason

1. OT bisects ∠WTY

⟹ ∠ATO = \(\frac{1}{2}\)∠WTY.

1. The tangents drawn to a circle from an external point are equally inclined to the line joining the point to the centre of the circle.

2. TP bisects ∠ZTX

⟹ ∠XTP = \(\frac{1}{2}\)∠ZTX.

2.  As in statement 1.

3. ∠WTY = ∠ZTX.

3. Vertically opposite angles.

4. ∠WTO = ∠XTP.

4. From statement 1, 2 and 3.

5. OT and TP lie on the same straight line

⟹ O, T, P are collinear. (Prove)

5. The two angles are forming a pair of vertically opposite angles.

You might like these

  • Circumcircle of a Triangle | Circumcentre of the Triangle | Diagram

    We will discuss here the Circumcircle of a Triangle and the circumcentre of a triangle. A tangent that passes through the three vertices of a triangle is known as the circumcircle of the triangle. When the vertices of a triangle lie on a circle, the sides of the triangle

  • Examples of Loci Based on Circles Touching Straight Lines | Diagram

    We will discuss here some Examples of Loci Based on Circles Touching Straight Lines or Other Circles. 1. The locus of the centres of circles touching a given line XY at a point M, is the straight line perpendicular to XY at M. Here, PQ is the required locus. 2. The locus of

  • Problems on Common Tangents to Two Circles | Transverse Common Tangent

    Here we will solve different types of problems on common tangents to two circles. 1.There are two circles touch each other externally. Radius of the first circle with centre O is 8 cm. Radius of the second circle with centre A is 4 cm Find the length of their common tangent

  • Problems on Relation Between Tangent and Secant | Square of Tangent

    Here we will solve different types of Problems on relation between tangent and secant. 1. XP is a secant and PT is a tangent to a circle. If PT = 15 cm and XY = 8YP, find XP. Solution: XP = XY + YP = 8YP + YP = 9YP. Let YP = x. Then XP = 9x. Now, XP × YP = PT^2, as the

  • Equilateral Triangle Inscribed | Sum of Three Angles of a Triangle

    We will prove that, PQR is an equilateral triangle inscribed in a circle. The tangents at P, Q and R form the triangle P’Q’R’. Prove that P’Q’R’ is also an equilateral triangle. Solution: Given: PQR is an equilateral triangle inscribed in a circle whose centre is O.




10th Grade Math

From Important Properties of Transverse Common Tangents to HOME PAGE


New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.



Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.