Subscribe to our ▶️ YouTube channel 🔴 for the latest videos, updates, and tips.
A Rhombus is a Parallelogram whose Diagonals Meet at Right Angles
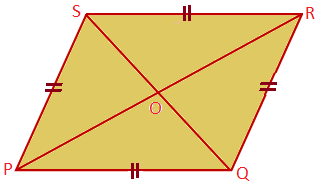
Here we will prove that a rhombus is a parallelogram whose diagonals meet at right angles.
Given: PQRS is a rhombus. So, by definition,
PQ = QR = RD = SP. Its diagonals PR and QS intersect at O.
To prove: (i) PQRS is a parallelogram.
(ii) ∠POQ = ∠QOR = ∠ROS = ∠SOP = 90°.
Proof:
|
Statement |
Reason |
|
(i) In ∆PQR and ∆RSP, 1. PQ = RS and QR = PS |
1. Given. |
|
2. PR = RP |
2. Common side |
|
3. ∆PQR ≅ ∆RSP Therefore, ∠QPR = ∠SRP, ∠QRP = ∠SPR. |
3. By SSS criterion of congruency. CPCTC |
|
4. SR ∥ PQ, PS ∥QR. |
4. Alternate angles are equal. |
|
5. PQRS is a parallelogram. (Proved) (ii) In ∆OPQ and ∆ORS, |
5. By definition. |
|
6. ∠OPQ = ∠ORS |
6. By statement 4, PQ ∥ SR and PR is a transversal. |
|
7. ∠OQP = ∠OSR |
7. P PQ ∥ SR and QS is a transversal |
|
8. PQ = SR |
8. Given. |
|
9. ∆OPQ ≅ ∆ORS Therefore, OP = OR, OQ= OS. In ∆POS ≅ ∆ROS, |
9. By AAS criterion of congruency. CPCTC |
|
10. PS = RS |
10. Given. |
|
11. OP = OR |
11. From statement 10. |
|
12. OS = SO |
12. Common side. |
|
13. Therefore, ∆POS ≅ ∆ROS |
13. By SSS criterion of congruency. |
|
14. ∠POS = ∠ROS |
14. CPCTC |
|
15. ∠POS + ∠ROS = 180° |
15. Linear pair. |
|
16. ∠POS = ∠ROS = 90° |
16. From statements 14 and 15. |
|
17. ∠POQ = ∠ROS, ∠QOR = ∠POS Therefore, ∠POQ = ∠QOR =∠ROS = ∠SOP = 90° (Proved) |
17. Opposite angles. |
From A Rhombus is a Parallelogram whose Diagonals Meet at Right Angles to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.